ABCD is a cyclic quadrilateral in which BC is paralleld to AD, angle ADC=110∘ and angle BAC=50∘. Find angle DAC and angle DCA.
The correct Answer is:60∘,10∘
To solve the problem, we will follow these steps:
Step 1: Draw the cyclic quadrilateral
We start by drawing a circle and labeling the points A, B, C, and D such that ABCD forms a cyclic quadrilateral. We know that BC is parallel to AD.
Step 2: Identify given angles
From the problem, we have:
- Angle ADC = 110°
- Angle BAC = 50°
Step 3: Use the property of cyclic quadrilaterals
In a cyclic quadrilateral, opposite angles are supplementary. Therefore, we can find angle ABC:
Angle ABC=180°−Angle ADC=180°−110°=70°
Step 4: Use the angle sum property in triangle ABC
In triangle ABC, we can apply the angle sum property:
Angle BAC+Angle ABC+Angle BCA=180°
Substituting the known values:
50°+70°+Angle BCA=180°
Angle BCA=180°−120°=60°
Step 5: Use the property of parallel lines
Since BC is parallel to AD, angle BCA is equal to angle CAD (alternate interior angles):
Angle DAC=Angle BCA=60°
Step 6: Find angle DCA
Now, we need to find angle DCA in triangle ACD. We know:
- Angle ADC = 110°
- Angle DAC = 60°
Using the angle sum property in triangle ACD:
Angle DAC+Angle DCA+Angle ADC=180°
Substituting the known values:
60°+Angle DCA+110°=180°
Angle DCA=180°−170°=10°
Final Answers
- Angle DAC = 60°
- Angle DCA = 10°
---
We start by drawing a circle and labeling the points A, B, C, and D such that ABCD forms a cyclic quadrilateral. We know that BC is parallel to AD.
From the problem, we have:
- Angle ADC = 110°
- Angle BAC = 50°
In a cyclic quadrilateral, opposite angles are supplementary. Therefore, we can find angle ABC:
Angle ABC=180°−Angle ADC=180°−110°=70°
In triangle ABC, we can apply the angle sum property:
Angle BAC+Angle ABC+Angle BCA=180°
Substituting the known values:
50°+70°+Angle BCA=180°
Angle BCA=180°−120°=60°
Since BC is parallel to AD, angle BCA is equal to angle CAD (alternate interior angles):
Angle DAC=Angle BCA=60°
Now, we need to find angle DCA in triangle ACD. We know:
- Angle ADC = 110°
- Angle DAC = 60°
Angle DAC+Angle DCA+Angle ADC=180°
Substituting the known values:
60°+Angle DCA+110°=180°
Angle DCA=180°−170°=10°
- Angle DAC = 60°
- Angle DCA = 10°
Topper's Solved these Questions
- TANGENTS AND INTERSECTING CHORDSBook:ICSEChapter:TANGENTS AND INTERSECTING CHORDSExercise:EXERCISE 18 (B)
Explore 18 Videos
- SPECIMEN QUESTION PAPER (MATHEMATICS)Book:ICSEChapter:SPECIMEN QUESTION PAPER (MATHEMATICS)Exercise:Section C
Explore 12 Videos
- TRIGONOMETRICAL IDENTITIESBook:ICSEChapter:TRIGONOMETRICAL IDENTITIESExercise:QUESTIONS
Explore 22 Videos
Similar Questions
ABCD is a cyclic quadrilateral in a circle with centre O.
If ∠ADC=130∘ , find angle BAC ?
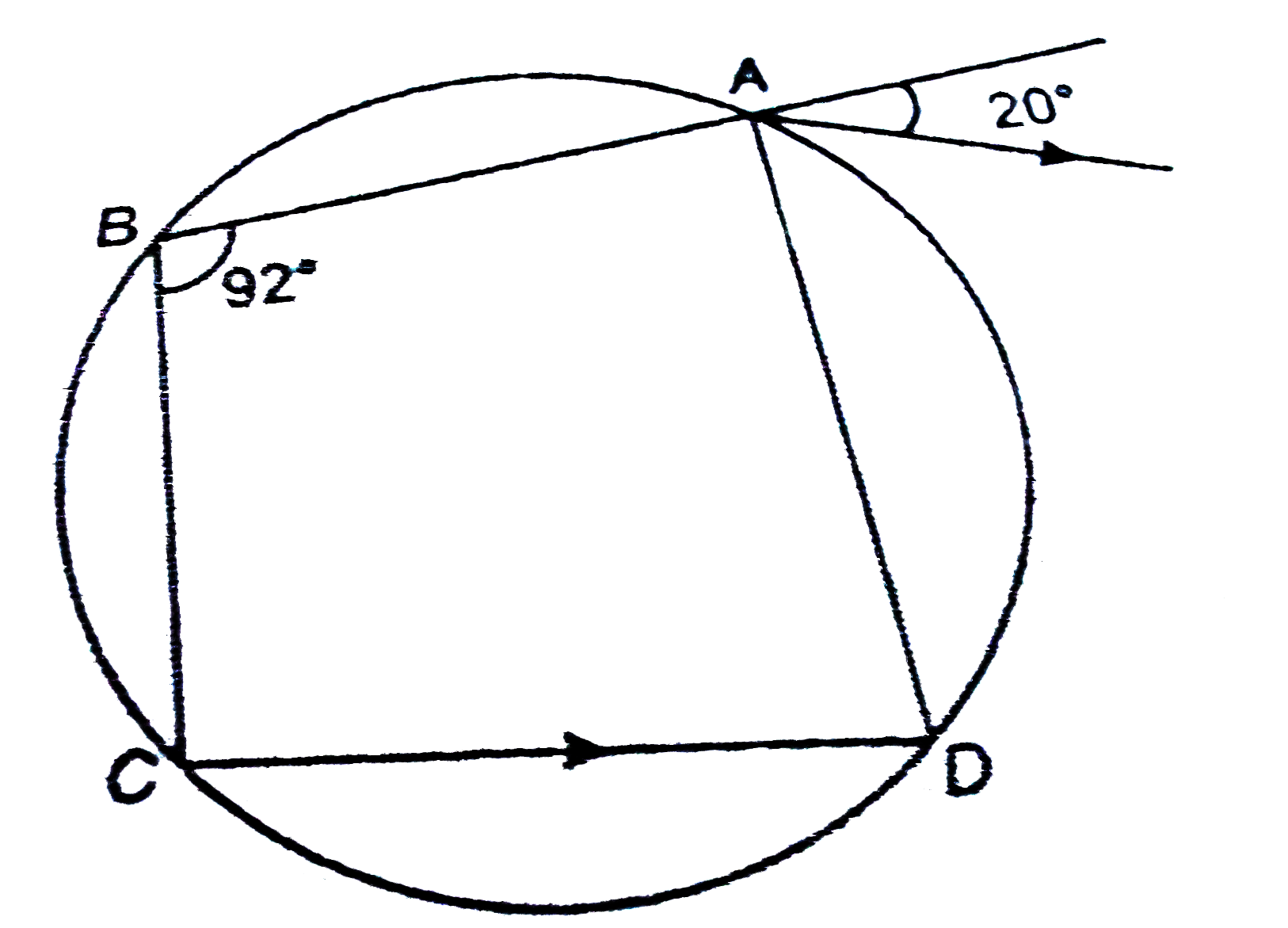
View SolutionIn the given figure ABCD is a cyclic quadrilateral in which AE is drawn parallel to CD and BA is produced. If ∠ABC=92∘ and ∠FAE=20∘, Find ∠BCD.
View SolutionIn cyclic quadrilateral ABCD , AD = BC, ∠BAC=30∘and∠CBD=70∘ , find : angle ADC .
View SolutionIn cyclic quadrilateral ABCD , AD = BC ,∠BAC=30∘and∠CBD=70∘ , find :
∠ABC
View SolutionIn cyclic quadrilateral ABCD , AD = BC ,∠BAC=30∘and∠CBD=70∘ , find :
∠BCA
View SolutionIn cyclic quadrilateral ABCD , AD = BC ,∠BAC=30∘and∠CBD=70∘ , find :
∠BCD
View SolutionABCD is a cyclic quadrilateral in which AB is parallel to DC and AB is a diameter of the circle. Given ∠BED=65∘ , Calculate
(i) ∠DAB
(ii) ∠BDC
View SolutionABCD is a cyclic quadrilateral such that ∠A=90∘,∠B=70∘,∠C=95∘and∠D=105∘.
View SolutionIn the given figure,AB is parallel to DC. ∠BCE=80∘and∠BAC=25∘.F∈d:(i) angle CAD <br>(ii) angle CBD <br>(iii) angle ADC
View SolutionIn the adjoining figure, ABCD is a cyclic quadrilateral whose side AB is the diameter of the circle . If ∠ADC=140∘ , then find the value of ∠BAC.

View Solution