The shaded portion of the figure, given alongside, shows two concentric circles.
If the circumference of the two circles be 396 cm and 374 cm, find the area of the shaded portion.

Video Solution

Text Solution

Verified by Experts

The correct Answer is:1347.5 $c m^{2}$

Updated on:21/07/2023

Answer

Step by step video, text & image solution for The shaded portion of the figure, given alongside, shows two concentric circles. If the circumference of the two circles be 396 cm and 374 cm, find the area of the shaded portion. by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.

Updated on:21/07/2023

Class 9 MATHS SURFACE AREAS AND VOLUMES

Topper's Solved these Questions

AREA AND PERIMETER OF PLANE FIGURESBOOK - ICSECHAPTER - AREA AND PERIMETER OF PLANE FIGURESEXERCISE - EXERCISE 20(D)
12 Videos
AREA AND PERIMETER OF PLANE FIGURESBOOK - ICSECHAPTER - AREA AND PERIMETER OF PLANE FIGURESEXERCISE - EXERCISE 20(B)
46 Videos
AREA THEOREMSBOOK - ICSECHAPTER - AREA THEOREMSEXERCISE - Exercies 16(C )
22 Videos

Knowledge Check

If the circumference of the circle above is $16 π$ , what is the total area of the shaded regions?
A $64 π$
B $32 π$
C $12 π$
D $8 π$

The shaded portion in the adjoining figure shows a circular path enclosed by two concentric circles. If the inner circumference of the path is 176 m and the uniform width of the circular path is 3.5 m, find the area of the path.

View Solution
In figure , AB is a diameter of the circle, AC = 6cm and BC = 8cm . Find the area of the shaded region . (use $π = 3.14$ )

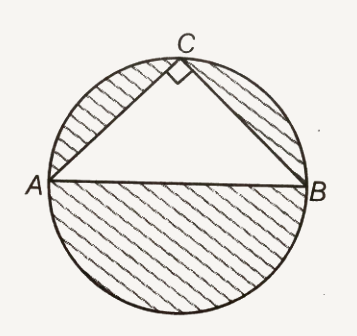
View Solution
In the figure, the area of the shaded portion is 770 $c m^{2}$ . If the circumference of the outer circle is 132 cm, find the width of the shaded part

View Solution
In the figure, AB is a diameter of the circle with centre O and OA = 7cm. Find the area of the shaded region
View Solution
The difference between the circumference and radius of a circle is 37 cm. Find the area of the circle
View Solution
ABC is an equilateral triangle inscribed in a circle of radius 4 cm. Find the area of the shaded portion.

View Solution
The area enclosed by the circumference of two concentric circles is $423.5 c m^{2}$ . If the circumference of outer circle is 132 cm. Calculate the radius of the inner circle
View Solution

ICSE-AREA AND PERIMETER OF PLANE FIGURES-EXERCISE 20(C)

The diameter of two circles are 32 cm and 24 cm. Find the radius of th...
02:41
The radius of a circle is 5 m. Find the circumference of the circle wh...
02:37
The circle of largest area is cut from a rectangular piece of card-boa...
05:38
The following figure shows a square card-board ABCD of side 28 cm. Fou...
05:47
The radii of two cirlces are in the ratio 3 : 8. If the difference be...
06:47
The diameters of three circles are in the ratio 3 : 5 : 6. If the sum...
06:12
Find the area of a ring shaped region enclosed between two concentric ...
03:12
The circumference of a given circular park is 55 m. It is surrounded b...
07:20
There are two circular gardens A and B. The circumference of garden ...
04:03
A wheel had diameter 84 cm. Find how many complete revolutions must it...
05:27
The wheels of a car are of diameter 80 cm each. How many complete r...
05:19
An express train is running between two stations with a uniform speed....
04:12
The minute hand of a clock is 8 cm long. Find the area swept by the m...
03:45
The shaded portion of the figure, given alongside, shows two concentri...
04:54
In the figure, the area of the shaded portion is 770 cm^(2). If the ci...
05:29
The cost of fencing a circular field at the rate of Rs 240 per metre i...
01:33
Two circles touch each other externally. The sum of their areas is 58...
04:49
The given figure shows a rectangle ABCD inscribed in a circle as shown...
04:25
A square is inscribed in a circle of radius 7 cm. Find the area of the...
02:08
A metal wire, when bent in the form of an equilateral triangle of larg...
04:22

Home
Ask Doubt
Profile

The shaded portion of the figure, given alongside, shows two concentric circles. If the circumference of the two circles be 396 cm and 374 cm, find the area of the shaded portion.

The correct Answer is:1347.5 cm2

Topper's Solved these Questions

Similar Questions

Knowledge Check

If the circumference of the circle above is 16π, what is the total area of the shaded regions?

ICSE-AREA AND PERIMETER OF PLANE FIGURES-EXERCISE 20(C)

The shaded portion of the figure, given alongside, shows two concentric circles.
If the circumference of the two circles be 396 cm and 374 cm, find the area of the shaded portion.

The correct Answer is:1347.5 $c m^{2}$

If the circumference of the circle above is $16 π$ , what is the total area of the shaded regions?