A half metre rod is pivoted at the centre with two weights of 20 gf and 12 gf suspended at a perpendicular distance of 6 cm and 10 cm from the pivot respectively as shown below.
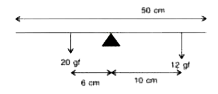
Which of the two forces acting on the rigid rod causes clockwise moment?
The force 12 gf rotates the rod clockwise.
Topper's Solved these Questions
Similar Questions
A half metre rod is pivoted at the centre with two weights of 20 gf and 12 gf suspended at a perpendicular distance of 6 cm and 10 cm from the pivot respectively as shown below:
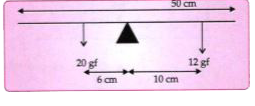
Is the rod in equilibrium ?View SolutionA half metre rod is pivoted at the centre with two weights of 20 gf and 12 gf suspended at a perpendicular distance of 6 cm and 10 cm from the pivot respectively as shown below:
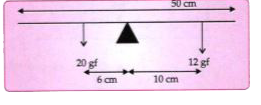
The direction of 20 kgf force is reversed. What is the magnitude of the resultant moment of the forces on the rod ?View Solution
Knowledge Check
- Question 1 - Select One
Find the weight of a metre scale balanced at 30cm mark such that two weights one of 30gf and 10gf are suspended at 5cm mark and 40cm mark respectively.
A10gfB105gfC25gfD32.5gf - Question 2 - Select One
Find the resultant moment of couple if two equal forces each of 6N are acting at a distance of 3cm each from the centre.
A36NmB360NmC3.6NmD0.36Nm
Two protons are a distance of 1×10−10cm from each other. The force acting on them are
View SolutionA rod is pivoted about its centre. A 10 N force is applied 4 m from the pivot and another force of 5 N is applied 2 m from the pivot, as shown. The magnitude of the total torque about the pivot is
 View Solution
View SolutionA body is pivoted at a point. A force of 10N is applied at a distance of 30cm from the pivot. Calculate the moment of force about the pivot.
View SolutionA very light rod of length l pivoted at O is connected with two springs of stiffness k1 & k2 at a distance of a & l from the pivot respectively. A block of mass ma attached with the spring k2 is kept on a smooth horizontal surface. Find the angular frequency of small oscillation of the block m.
_S01_031_Q01.png) View Solution
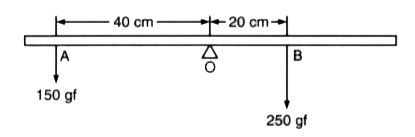
View SolutionThe diagram in Fig.1.34 shows a uniform metre rule weighing 100gf, pivoted at its centre O. Two weights 150 gf and 250 gf hang from the points A and B respectively of the metre rule such that OA= 40cm and OB=20cm . Calculate (i) the total anticlockwise moment about O, (ii) the total clockwise moment about O, (iii) the difference of anticlockwise and clockwise moments, and (iv) the distance from O where a 100gf weight should be placed to balanced the metre rule.
 View Solution
View SolutionA uniform half metre rule balances horizontally on a knife edge at 29 cm mark when a weight of 20 gf is suspended from one end.
Draw a diagram of the arrangement.View Solution
