Two resistors of 2.0 92 and 3.0 22 are connected
in parallel, with a battery of 60 V and negligible internal resistance. For each case draw a circuit diagram and calculate the current through the battery
To solve the problem step by step, we will follow these instructions:
Step 1: Draw the Circuit Diagram
We need to represent the battery and the two resistors in parallel.
Circuit Diagram:
±−−−−(R1:2.0Ω)−−−−−+||60V||∣∣±−−−−(R2:3.0Ω)−−−−−+
Step 2: Calculate the Effective Resistance (R_effective)
For resistors in parallel, the formula for effective resistance \( R_{effective} \) is given by:
\(
\frac{1}{R_{effective}} = \frac{1}{R_1} + \frac{1}{R_2}
\)
Substituting the values:
\(
\frac{1}{R_{effective}} = \frac{1}{2.092} + \frac{1}{3.022}
\)
Calculating each term:
\(
\frac{1}{R_{effective}} = 0.478 + 0.331 = 0.809
\)
Now, taking the reciprocal to find \( R_{effective} \):
\(
R_{effective} = \frac{1}{0.809} \approx 1.236 \, \Omega
\)
Step 3: Calculate the Current through the Battery (I)
Using Ohm's Law, the current \( I \) through the battery can be calculated using the formula:
\(
I = \frac{V}{R_{effective}}
\)
Where:
- \( V = 60 \, V \)
- \( R_{effective} = 1.236 \, \Omega \)
Substituting the values:
\(
I = \frac{60}{1.236} \approx 48.54 \, A
\)
Final Answer
The current through the battery is approximately \( 48.54 \, A \).
---
We need to represent the battery and the two resistors in parallel.
±−−−−(R1:2.0Ω)−−−−−+||60V||∣∣±−−−−(R2:3.0Ω)−−−−−+
For resistors in parallel, the formula for effective resistance \( R_{effective} \) is given by:
\frac{1}{R_{effective}} = \frac{1}{R_1} + \frac{1}{R_2}
\)
\frac{1}{R_{effective}} = \frac{1}{2.092} + \frac{1}{3.022}
\)
\frac{1}{R_{effective}} = 0.478 + 0.331 = 0.809
\)
R_{effective} = \frac{1}{0.809} \approx 1.236 \, \Omega
\)
Using Ohm's Law, the current \( I \) through the battery can be calculated using the formula:
I = \frac{V}{R_{effective}}
\)
- \( V = 60 \, V \)
- \( R_{effective} = 1.236 \, \Omega \)
I = \frac{60}{1.236} \approx 48.54 \, A
\)
The current through the battery is approximately \( 48.54 \, A \).
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - ICSECHAPTER - CURRENT ELECTRICITYEXERCISE - EXERCISE-8(C)
22 Videos
- CURRENT ELECTRICITYBOOK - ICSECHAPTER - CURRENT ELECTRICITYEXERCISE - EXERCISE-8(C) MULTIPLE CHOICE TYPE)
2 Videos
- CURRENT ELECTRICITYBOOK - ICSECHAPTER - CURRENT ELECTRICITYEXERCISE - EXERCISE-8(B) (MULTIPLE CHOICE TYPE)
3 Videos
- CALORIMETRYBOOK - ICSECHAPTER - CALORIMETRYEXERCISE - EXERCISE-11(B) (NUMERICALS)
15 Videos
- ELECTRO-MAGNETISM BOOK - ICSECHAPTER - ELECTRO-MAGNETISM EXERCISE - EXERCISE-10 (C) NUMERICALS
1 Video
Similar Questions
Two resistors of 4 Ω and 6 Ω are connected in parallel. The combination is connected across a 6 V battery of negligible resistance. (i) Draw a circuit diagram. (ii) Calculate :the current through each resistor
View SolutionTwo resistors of 4 Ω and 6 Ω are connected in parallel. The combination is connected across a 6 V battery of negligible resistance. (i) Draw a circuit diagram. (ii) Calculate :the current through the battery
View Solution
Knowledge Check
Three resistors 2Ω,4Ωand5Ω are combined in parallel. This combination is connected to a battery of emf 20V and negligible internal Resistance. The total current drawn from the battery is
A10AB15AC19AD23A
Two resistors of 4 Ω and 6 Ω are connected in parallel. The combination is connected across a 6 V battery of negligible resistance. (i) Draw a circuit diagram. (ii) Calculate : (a) the total resistance of the circuit,
View SolutionTwo resistors of resistances 2Ω and 6Ω are connected in parallel. This combination is then connected to a battery of emf 2 V and internal resistance 0.5Ω. What is the current flowing through the battery ?
View SolutionThree resistors having resistances of 1.60Ω,2.40Ω and 4.80Ω are connected in parallel to a 28.0V battery that has negligible internal resistance. Find
(a) the equivalent resistance of the combination.
(b) the current in each resistor.
(c) the total current through the battery.
(d) the voltage across each resistor.
(e) the power dissipated in each resistor.
(f) which resistor dissipates the maximum power the one with the greatest resistance or the least resistance? Explain why this should be.
View SolutionA battery of emf 2V and internal resistance 0.5(Ω)is connected across a resistance 1.5(Ω). Find the current flow through battery ?
View Solution(a) Three resistors 2Ω,4Ωand5Ω are combined in parallel. What is the total resistance of the combination ?
(b) If the combination is connected to a battery of emf 20 V and negligible internal resistance, determine the current through each resistor, and the total current drawn from the battery.
View SolutionA 3 volt battery with negligible internal resistance is connected in a circuit as shown in the figure. The current I, in the circuit will be
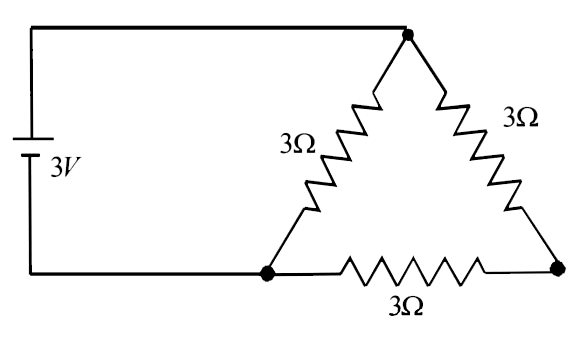
View SolutionA battery of emf 2 V and internal resistance r is connected in series with a resistor of 10Ω through an ammeter of resistance 2Ω. The ammeter reads 50 mA. Draw the circuit diagram and calculate the value of r.
View Solution
