What work is required to bring a charge q=3.00μC originally a distance of 1.50 m from a charge Q=20.0μC until it is 0.50 m away ?
To find the work required to bring a charge q=3.00μC from a distance of r1=1.50m to a distance of r2=0.50m from another charge Q=20.0μC, we can use the concept of electric potential energy.
1. Identify the Formula for Electric Potential Energy:
The electric potential energy U between two point charges is given by the formula:
U=k⋅Q⋅qr
where k is Coulomb's constant (k≈9×109N⋅m2/C2), Q is the charge creating the electric field, q is the charge being moved, and r is the distance between the charges.
2. Calculate Initial Potential Energy U1:
The initial potential energy when the charge q is at distance r1=1.50m:
U1=k⋅Q⋅qr1=9×109⋅20×10−6⋅3×10−61.50
U1=9×109⋅60×10−121.50=9×109⋅40×10−12=360×10−3=0.36J
3. Calculate Final Potential Energy U2:
The final potential energy when the charge q is at distance r2=0.50m:
U2=k⋅Q⋅qr2=9×109⋅20×10−6⋅3×10−60.50
U2=9×109⋅60×10−120.50=9×109⋅120×10−12=1080×10−3=1.08J
4. Calculate the Work Done W:
The work done W in bringing the charge from r1 to r2 is given by the change in potential energy:
W=U2−U1=1.08J−0.36J=0.72J
Final Answer:
The work required to bring the charge q from a distance of 1.50m to 0.50m away from the charge Q is 0.72J.
The electric potential energy U between two point charges is given by the formula:
U=k⋅Q⋅qr
where k is Coulomb's constant (k≈9×109N⋅m2/C2), Q is the charge creating the electric field, q is the charge being moved, and r is the distance between the charges.
The initial potential energy when the charge q is at distance r1=1.50m:
U1=k⋅Q⋅qr1=9×109⋅20×10−6⋅3×10−61.50
U1=9×109⋅60×10−121.50=9×109⋅40×10−12=360×10−3=0.36J
The final potential energy when the charge q is at distance r2=0.50m:
U2=k⋅Q⋅qr2=9×109⋅20×10−6⋅3×10−60.50
U2=9×109⋅60×10−120.50=9×109⋅120×10−12=1080×10−3=1.08J
The work done W in bringing the charge from r1 to r2 is given by the change in potential energy:
W=U2−U1=1.08J−0.36J=0.72J
The work required to bring the charge q from a distance of 1.50m to 0.50m away from the charge Q is 0.72J.
Similar Questions
What minimum work must be done by an external force to bring a charge q=3.00μC from a great distance away (take t=∞) to a point 0.500m from a charge =20.0μC ?
View SolutionA particle of mass 100 gm and charge 2μC is released from a distance of 50 cm from a fixed charge of 5μC. Find the speed of the particle when its distance from the fixed charge becomes 3 m.
View Solution
Knowledge Check
The work done in bringing a unit positive charge from infinite distance to a point at distance x from a positive charge Q is W. Then the potential ϕ at that point is
AWQxBWCWxDWQ
There exists a uniform electric field E =4 ×105Vm−3 directed along negative x-axis such that electric potential at origin is zero. A charge of - 200 μC is placed at origin and a charged of + 200 μ C is placed at a (3 m, 0) . The electrostatic potential energy of the system is
View SolutionFind the electric work done in bringing a charge q from A to B in a sphere of charge Q distributed uniformly throughout its volume.
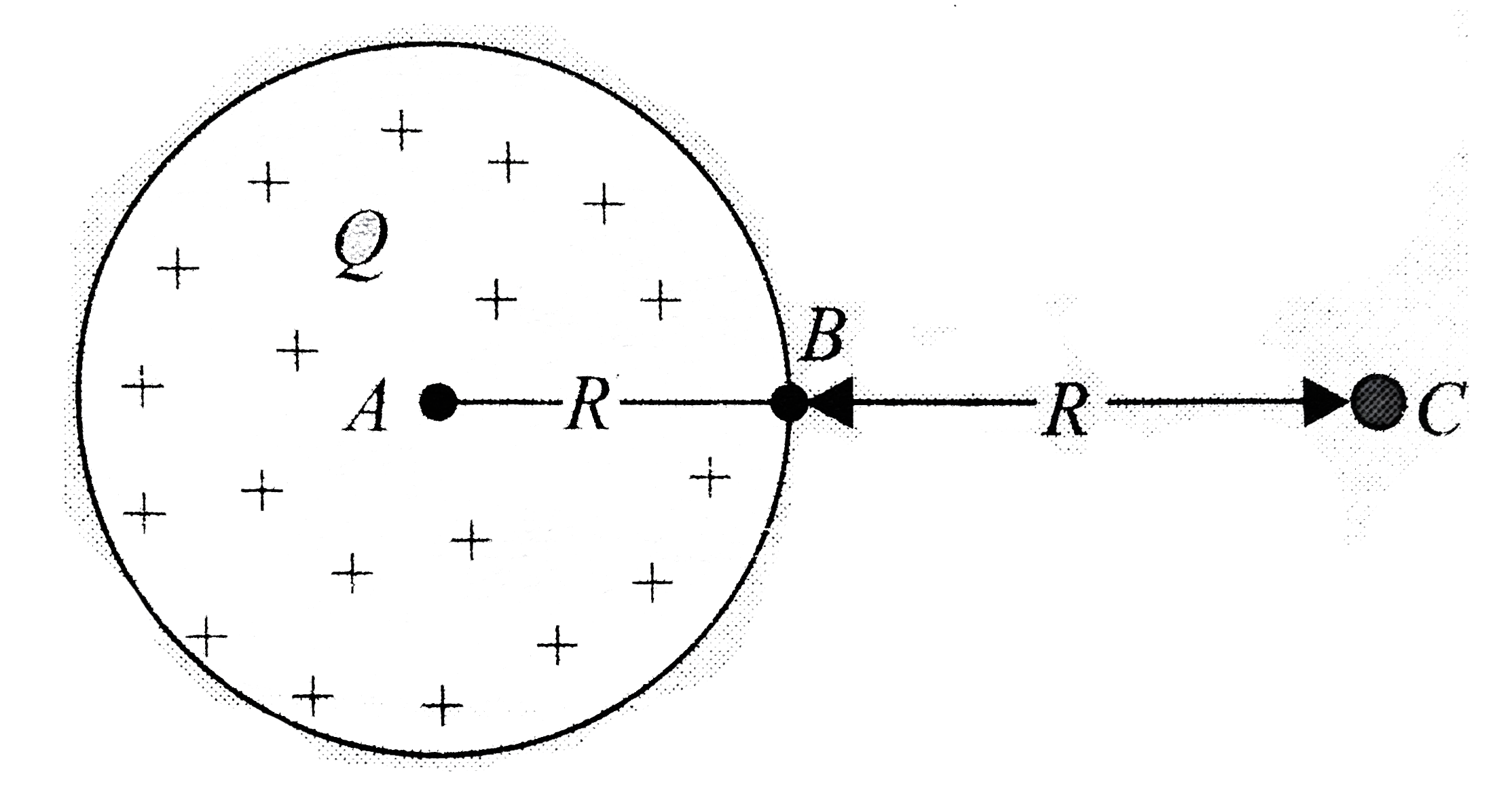 .
.
View SolutionTwo identical rings PandQ of radius 0.1m are mounted coaxially at a distance 0.5m apart. The charges on the two rings are 2μCand4μC, respectively. The work done in transferring a charge of 5μC from the center of P to that of Q is.
View SolutionA point charge q1=1.0μC is held fixed at origin. A second point charge q2=−2.0μC and a mass 10−4 kg is placed on the x-axis, 1.0 m from the origin. The second point charge is released from rest. What is its speed when it is 0.5 m from the origin?
View SolutionA point charge q1=4.00nC is placed at the origin, and a second point charge q2=−3.00nC, placed on the x-axis at x = + 20.0 cm. A third point charge q3=2.00nC is placed on the X-axis between q1,andq2. (Take as zero the potential energy of the three charges when they are infinitely far apart).
(a) What is the potential energy of the system of the three charges if q3 is placed at x= + 10.0 cm?
(b) Where should q3 be placed to make the potential energy of the system equal to zero?
View SolutionTwo ball with charges 5μCand10μC are at a distance of 1 m from each other. In order to reduce the distance between them to 0.5 m , the amount of work to be performed is
View SolutionAn inductor of inductance 2.0mH s connected across a charged capacitor of capacitance 5.0μF and the resulting LC circuit is set oscillating at its natural frequency. Let Q denote the instantaneous charge on the capacitor and I the current in the circuit. It is found that the maximum value of charge Q is 200μC.
a. When Q=100μC, what is the value of ∣∣∣dIdt∣∣∣?
b. When Q=200μC, what is the value of I?
c. Find the maximum value of I.
d. When I is equal to one-half its maximum value, what is the value of |Q|?
View Solution