A bullet loses 1/20 of its velocity in passing through a plank. What is the least number of plank required to stop the bullet .
11
20
21
infinite
The correct Answer is:A
To solve the problem of determining the least number of planks required to stop a bullet that loses 120 of its velocity upon passing through each plank, we can follow these steps:
Step 1: Determine the velocity after passing through one plank
Let the initial velocity of the bullet be u. When the bullet passes through one plank, it loses 120 of its velocity. Thus, the velocity after passing through one plank, denoted as v, can be calculated as follows:
v=u−u20=u(1−120)=u(1920)
Step 2: Use the equation of motion
We can use the equation of motion to relate the initial and final velocities with acceleration and distance. The equation is:
v2−u2=2as
Where:
- v is the final velocity after passing through the plank,
- u is the initial velocity,
- a is the deceleration (negative acceleration),
- s is the distance traveled through the plank.
Substituting v=19u20 into the equation gives:
(19u20)2−u2=2as
Step 3: Simplify the equation
Calculating (19u20)2:
(19u20)2=361u2400
Now substituting back into the equation:
361u2400−u2=2as
Rearranging gives:
361u2400−400u2400=2as
This simplifies to:
−39u2400=2as
Step 4: Relate the distance to multiple planks
Let n be the number of planks. The total distance s′ traveled through n planks is:
s′=n⋅s
Substituting s′ into the equation gives:
2a(n⋅s)=−39u2400
Step 5: Solve for n
We know that the bullet must come to a stop, which means the final velocity after passing through all n planks must be 0. Therefore, we can set up the equation:
0−u2=2a(n⋅s)
Setting the two equations equal gives:
2a(n⋅s)=−39u2400
Substituting the expression for 2as from earlier, we have:
n⋅−39u2400=−u2
Now, simplifying this gives:
n⋅39400=1
Thus, solving for n:
n=40039≈10.25
Step 6: Round up to the nearest whole number
Since n must be a whole number (you can't have a fraction of a plank), we round up to the next whole number:
n=11
Final Answer
The least number of planks required to stop the bullet is 11.
---
Let the initial velocity of the bullet be u. When the bullet passes through one plank, it loses 120 of its velocity. Thus, the velocity after passing through one plank, denoted as v, can be calculated as follows:
We can use the equation of motion to relate the initial and final velocities with acceleration and distance. The equation is:
- v is the final velocity after passing through the plank,
- u is the initial velocity,
- a is the deceleration (negative acceleration),
- s is the distance traveled through the plank.
Calculating (19u20)2:
Let n be the number of planks. The total distance s′ traveled through n planks is:
We know that the bullet must come to a stop, which means the final velocity after passing through all n planks must be 0. Therefore, we can set up the equation:
Since n must be a whole number (you can't have a fraction of a plank), we round up to the next whole number:
The least number of planks required to stop the bullet is 11.
Topper's Solved these Questions
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - Assignment (SECTION - B)
35 Videos
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - Assignment (SECTION - C)
80 Videos
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - EXERCISE
37 Videos
- WAVESBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WAVESEXERCISE - ASSIGNMENT ( SECTION-D ( Assertion - Reason Type Questions ))
12 Videos
Similar Questions
A bullet losses 1/n of its velocity in passing through a plank. What is the least number of plancks required to stop the bullet ? (Assuming constant retardation)
View SolutionA bullet moving with a speed of 100 m/s can just penetrate two planks of equal thickness. Now if the speed is doubled, then the number of such planks required to stop the bullet will be
View Solution
Knowledge Check
A bullet is fired normally towards an immovable wooden block. If it loses 25% of its kinetic energy in penetrating through the block at thickness x, the distance penetrated by the bullet into the block is
A4xB6xC8xD2x
A 10 g bullet is fried at a plank od wood with a speed of 200m/s. After passing through the plank which is 1.0 m thick the speed of the bullet reduces to 100m/s. Find the average resistance offered by the plank.
View SolutionTwo wooden plank of mass M1=1kg,M2=2.98kg smooth surface. A bullet of mass m=20gm strikes the block M1 and pierces through it, then strikes the plank B and sticks to its. Consequently both the planks move with equal velocities. Find the percentage change in speed of the bullet when it escapes from the first block.
View SolutionA gun of mass 10kg fires 4 bullets per second. The mass of each bullet is 20 g and the velocity of the bullet when it leaves the gun is 300ms−1. The force required to hold the gun while firing is
View SolutionA gun is firing bullets with velocity v0 by rotating it through 360∘ in the horizontal plane. The maximum area covered by the bullets is
View SolutionA bullet of mass 'm' moving with velocity 'u' passes through a wooden block of mass M=nm as shown in figure. The block is resting on a smooth horizontal floor. After passing through the block, velocity relative to the block is
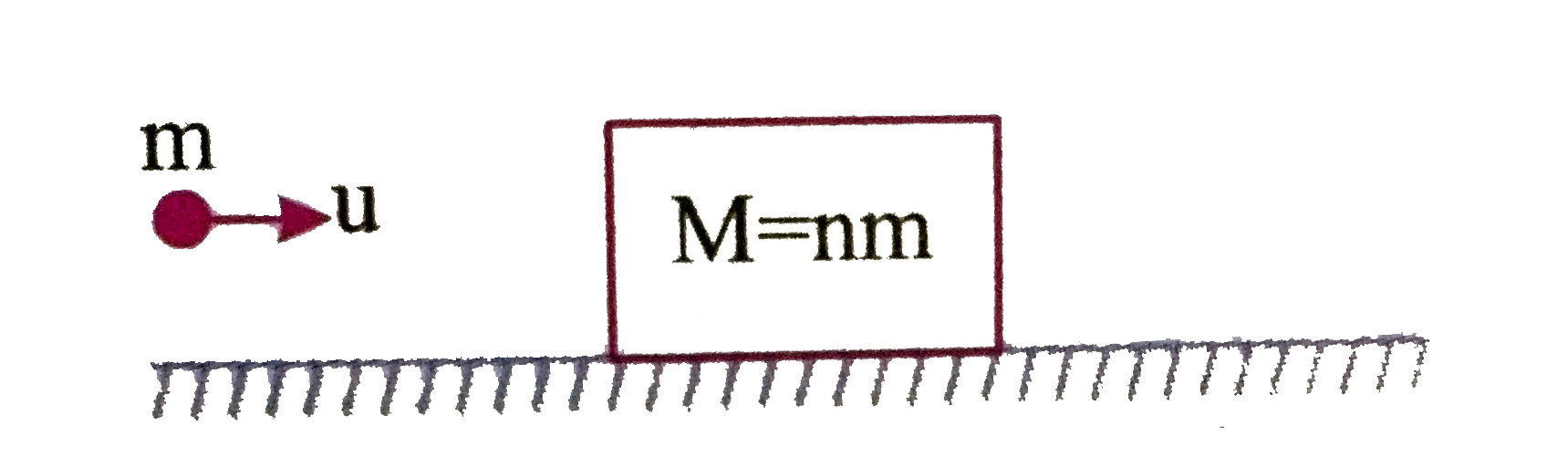 .
.
View SolutionA 2 g bullet moving with a velocity of 200 m/s is brought to a sudden stoppage by an obstacle. The total heat produced goes to the bullet. If the specific heat of the bullet is 0.03cal/g∘C, the rise in its temperature will be
View SolutionA bullet of mass 5 g is fired at a velocity of 900ms−1 from a rifle of mass 2.5 kg. What is the recoil velocity of the rifle?
View Solution
