A particle of mass 100 g, is made to describe a vertical circle of radius 1 m. Its instantaneous speed is 1ms−1 when the string makes an sngle of 30∘ with the vertical Find the tension in the string at this position. Can the particle complete its circular path? (g=10ms−2)
Hint : Tension in the string when it makes an angle θ with the vertical is
T=mv2r+mgcosθ
Let speed at the lowest point be v1
v21+v2+2gr(1−cosθ)
(v1)min=√5gr check ifv1<(v1)min
If yes then it would not compete its vertical circular path.
The tension in the string, when it makes an angle θ with the vertical is
T=mv2r+mgcosθ=0.1×121+0.1×10×0.866=0.966N
Let the speed at the lowest point be v1
v21=v2+2gr(1−cosθ)
=12+2×10×1×(1−0.866)
=1+20×0.134
=3.68
v1=√3.98=1.91ms−1
(v1)min=√5gr=√5×10×1=7.07ms−1
v1<(v1)min⇒ the particle would not be able to complete its circular path.
Similar Questions
A particle of mass 200 g , is whirled into a vertical circle of radius 80 cm uisig a massless string The speed of the particle when the string makes an angle of 60∘ with the vertical line is 1.5ms−1. The tension in the string at this position is
View SolutionA small stone of mass 100 g in rotated I a verticlal circle of radius 40 cm. What is the minimum speed needed at the lowest point for looping the loop? Also find the tension in the string at this point (g=10ms−2).
View SolutionA ball of mass 0.6kg attached to a light inextensible string rotates in a vertical circle of radius 0.75m such that it has speed of 5ms−1 when the string is horizontal. Tension in the string when it is horizontal on other side is (g=10ms−2).
View SolutionA particle of mass 100 g tied to a string is rotated along the circle of radius 0.5 m. The breaking tension of the string is 10 N. The maximum speed with which particle can be rotated without breaking the string is
View SolutionA particle of mass 200 g is moving in a circle of radius 2 m. The particle is just looping the loop. The speed of the particle and the tension in the string at highest point of the circule path are (g=10ms−2)
View SolutionA particle of mass 200 g is moving in a circle of radius 2 m. The particle is just looping the loop. The speed of the particle and the tension in the string at highest point of the circule path are (g=10ms−2)
View SolutionA particle of mass m attached to an inextensible light string is moving in a vertical circle of radius r. The critical velocity at the highest point is v0 to complete the vertical circle. The tension in the string when it becomes horizontal is
View SolutionCalculate the tension in the string shown in the figure. All the surfaces are frictionless. (Take g=10/ms−1)
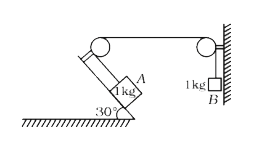
View SolutionA particle is moving on a circular track of radius 30 cm with a constant speed of 6ms−1. Its acceleration is
View SolutionA simple pendulum is constructed by attaching a bob of mass m to a string of length L fixed at its upper end. The bob oscillates in a vertical circle. It is found that the speed of the bob is v when the string makes an angle θ with the vertical. Find the tension in the string at this instant.
View Solution
