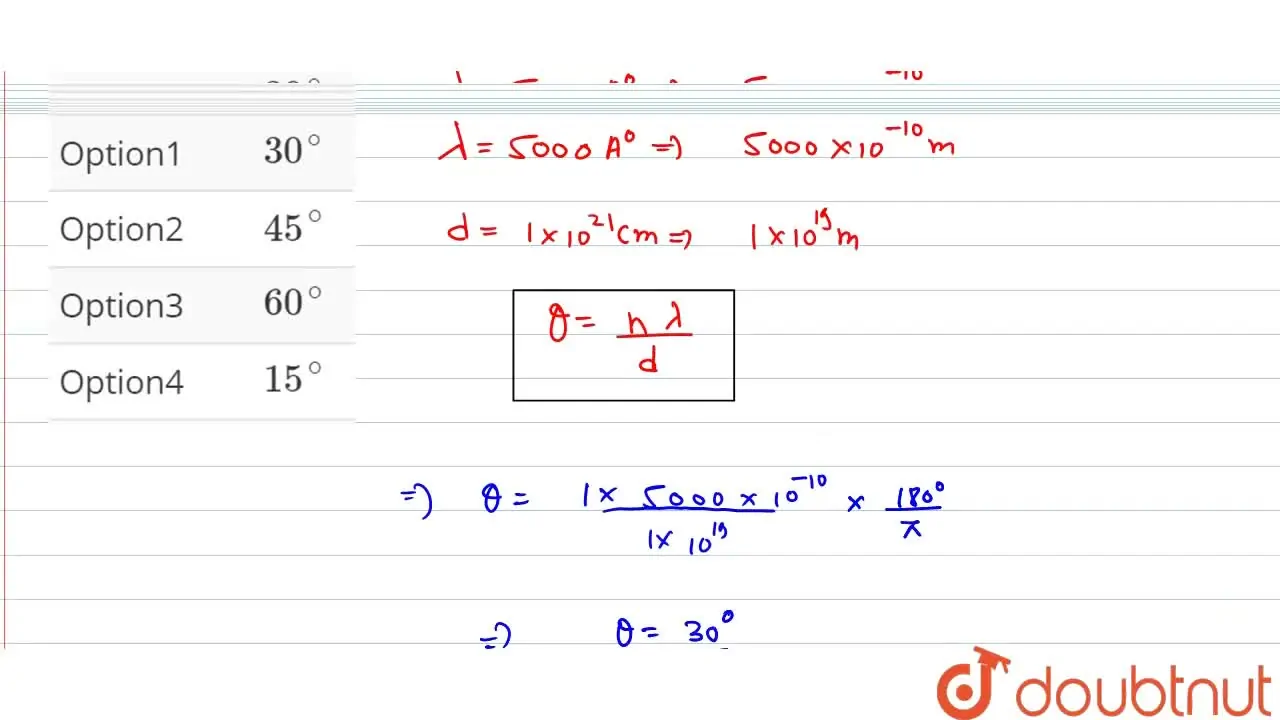
The first diffraction minimum due to single slit diffraction is θ for a light of wavelength 5000A if the width of the slit is 1×1021cm then the value of θ is
30∘
45∘
60∘
15∘
θ=λd=5000×10−1010−4×10−2×180∘π=28.6∘
Similar Questions
The first diffraction minima due to a single slit diffraction is at θ=30∘ for a light of wavelength 5000Å The width of the slit is
View SolutionThe Ist diffraction minimum due to single slit diffraction is θ, for a light of wave length 5000Å . If the width of the slit si 1×10−4cm then the value of θ
View SolutionThe first diffraction minimum due to a single slit Fraunhoffer diffraction is at the angle of diffraction 30∘ for a light of wavelength 5460A∘. The width of the slit is
View SolutionFirst diffraction minima due to a single slit of width 10−4cm is at θ=30∘. The wavelength of the light used is –
View SolutionIn a single slit diffraction of light of wavelength λ by a slit of width e, the size of the central maximum on a screen at a distance b is
View SolutionIn a single-slit diffraction experiment, the width of the slit is made half of the original width:
View SolutionUse Huygens's principle to explain the formation of diffraction pattern due to a single slit illuminated by a monochromatic source of light.
When the width of the slit is made double the original width, how would this affect the size and intensity of the central diffraction band ?View SolutionWhat will be the angle of diffracting for the first minimum due to Fraunhofer diffraction with sources of light of wavelength 550nm and slit of width 0.55mm?
View SolutionThe distance between the first and fifth minima of a single slit diffraction patterns is 0.40 mm with screen 50 cm away from the slit, using light of wavelength 550 . (a) Find the slit width (b) Calculate the angle of the first diffraction minimum
View SolutionIn a Fraunhofer diffraction experiment at a single slit using a light of wavelength 400 nm, the first minimum is formed at an angle of 30∘. The direction θ of the first secondary maximum is given by :
View Solution