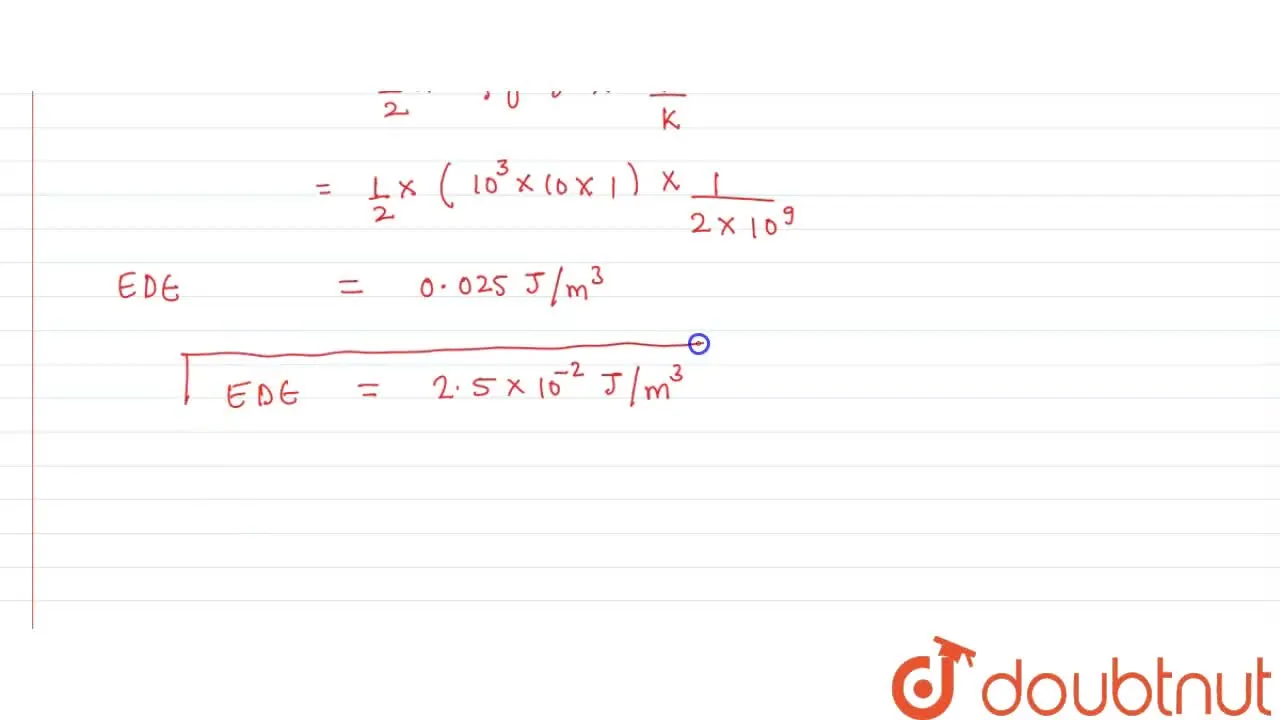
Find the volume density of the elastic deformation energy in fresh water at a depth of h = 1 m. (Bulk modulus of water = 2 ×109N/m2)
2.5×10−2J/m3
Related Playlists
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - SECTION - I6videos
MECHANICAL PROPERTIES OF FLUIDS
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MAGNETISM AND MATTER EXERCISE - ASSIGNMENT (SECTION D)26videos
MAGNETISM AND MATTER
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - Assignment(Section-D)14videos
MECHANICAL PROPERTIES OF SOLIDS
Similar Questions
Calculate the apprroximate change in density of water in a lake at a depth of 400m below the surface. The density of water at the surface id 1030kg/m3 and bulk modulus of water is 2×109N/m2.
View SolutionCalculate the apprroximate change in density of water in a lake at a depth of 400m below the surface. The density of water at the surface id 1030kg/m3 and bulk modulus of water is 2×109N/m2.
View SolutionArtificial diamond is made by subjected carbon in the form of graphite to a pressure of 1.55×1010N/m2 at a high temperature. Assuming that the natrual diamond formed at similar high presssure within the earth, calcualte its original volume if the mass of the diamond before cutting was 150g. The density of diamond = 3000 kg/m2.Bulk modulus =6.2×10111N/m2.
View SolutionHow much should the pressure on a litre of water be changed to compress it by 0.10%? Bulk modulus of elasticity of water = 2.2×109Nm−2.
View SolutionFind the increase in pressure required to decrease the volume of a water sample of 0.01%. Bulk modulus of water =2.1×109Nm−2.
View SolutionWhen a compressible wave is sent towards bottom of sea from a stationary ship it is observed that its echo is hear after 2s. If bulk modulus of elasticity of water is 2×109N/m2, mean temperature of water is 4∘ and mean density of water is 1000kg/m3, then depth of sea will be
View SolutionA wire of density 9×103kg/m3 is stretched between two clamps 1m apart and is stretched to an extension of 4.9×10−4m. Young's modulus of material is 9×1010N/m2.
View SolutionThe volume of a solid is 6×10−3m3 under 2 atm pressure. Find the change in volume when subjected to a pressure of 102 atm. Bulk modulus of the material =1011Nm−2.
View SolutionCompute the fractional change in volume of a glass slab, when subjected to a hydraulic pressure of 10 atmosphere. Bulk modulus of elasticity of glass = 37×109Nm−2 and 1 atm = 1.013×105Pa.
View SolutionCalculate the elastic potential energy per unit volume of water at a depth of 1km. Compressibility (α) of water =5×10−10 SI units. Density of water =103kg/m3
View Solution