A thin ring of radius R is made of a material of density ρ and Young's modulus Y. If the ring is rotated about its centre in its own plane with angular velocity ω , find the small increase in its radius.
Consider an element PQ of length dl. Let T be the tension and A the area of cross section of the wire.
Mass of element dm=Volume×density
=A(dl)ϕ
The component of T, towards the centre provides the necessary centripetal force
∴2Tsin(dθ)2=(dm)Rω2 ...(i)

For small angle, sin(dθ)2≈dθ2≈(dlR)2
Substitution in equation (i), we have
2Tdθ2=(dm)Rω2
T.dlR=A(dl)ϕRω2
T=Aϕω2R2
Tension in the ring is Aϕω2R2
ΔRR=(T)(AY)=ϕω2R2Y⇒ΔR=ϕω2R3Y

Topper's Solved these Questions
- MECHANICAL PROPERTIES OF SOLIDSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - Try Yourself
32 Videos
- MECHANICAL PROPERTIES OF SOLIDSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - Assignment (SECTION - A)
31 Videos
- MECHANICAL PROPERTIES OF FLUIDSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - SECTION - J
9 Videos
- Mock test 03BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - Mock test 03EXERCISE - EXAMPLE
44 Videos
Similar Questions
A ring of radius R is made of a thin wire of material of density ρ, having cross-section area a and Young's modulus y. The ring rotates about an axis perpendicular to its plane and through its centre. Angular frequency of rotation is ω.
The tension in the ring will be
View SolutionA ring of radius R is made of a thin wire of material of density ρ, having cross-section area a and Young's modulus y. The ring rotates about an axis perpendicular to its plane and through its centre. Angular frequency of rotation is ω.
The ratio of kinetic energy to potential energy is
View SolutionA ring of radius R is made of a thin wire of material of density ρ having cross section area a. The ring rotates with angular velocity ω about an axis passing through its centre and perpendicular to the plane. If we consider a small element of the ring,it rotates in a circle. The required centripetal force is provided by the component of tensions on the element towards the centre. A small element of length dl of angular width dθ is shown in the figure.
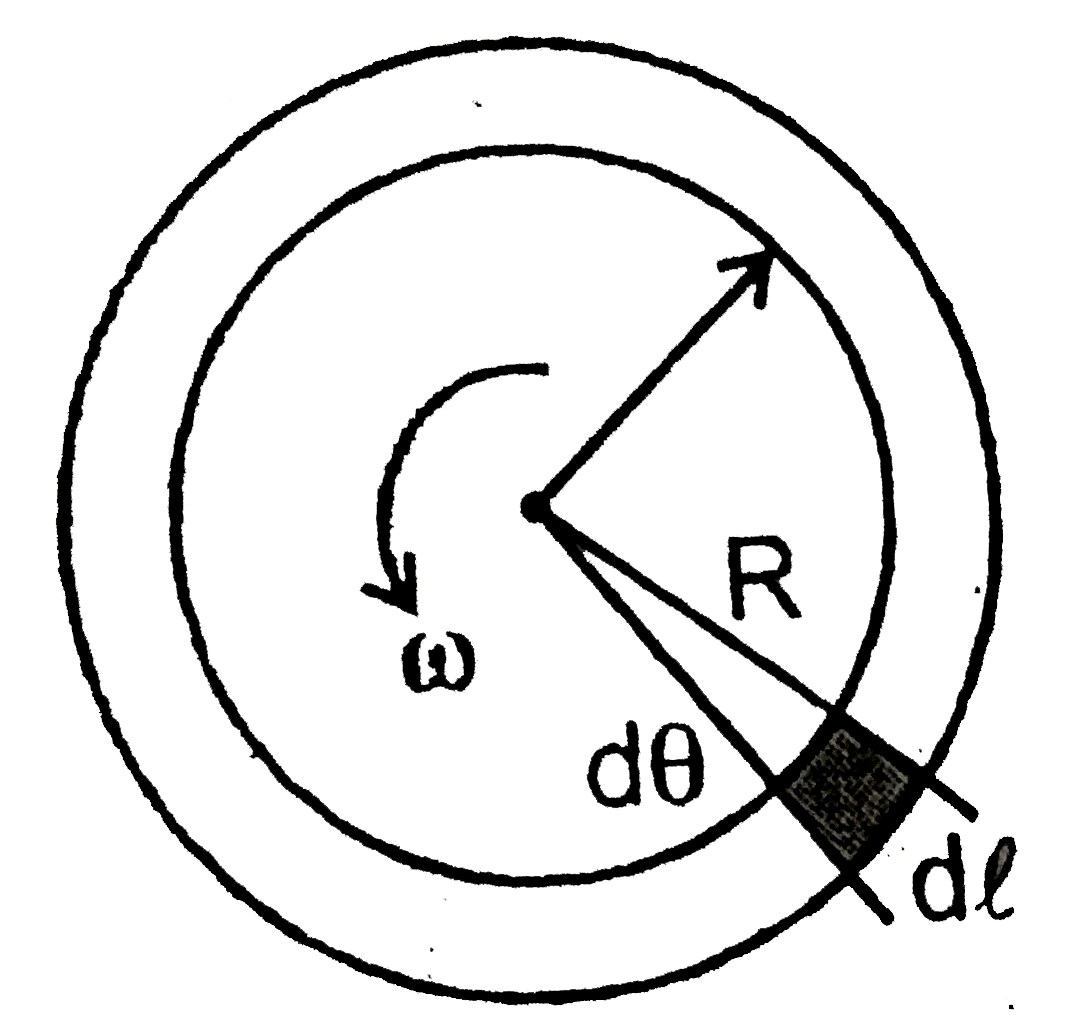
If T is the tension in the ring, then
View SolutionA ring of radius R is made of a thin wire of material of density ρ having cross section area a. The ring rotates with angular velocity ω about an axis passing through its centre and perpendicular to the plane. If we consider a small element of the ring,it rotates in a circle. The required centripetal force is provided by the component of tensions on the element towards the centre. A small element of length dl of angular width dθ is shown in the figure.

If for a given mass of the ring and angular velocity, the radius R of the ring is increased to 2R, the new tension will be
View SolutionA ring of mass m and radius R is being rotated about its axis with angular velocity o. If o increases then tension in ring
View SolutionA thin disc of radius R has charge Q distributed uniformly on its surface. The disc is rotated about one of its diametric axis with angular velocity ω. The magnetic moment of the arrangement is
View SolutionA ring of mass m and radius r rotates about an axis passing through its centre and perpendicular to its plane with angular velocity ω. Its kinetic energy is
View Solutionconsider a nonconducting ring of radius r and mass m which has a total charge q distributed uniformly on it the ring is rotated about its axis with an angular speed ω. (a) Find the equivalent electric current in the ring (b) find the magnetic moment μ of the . (c) show thatμ=q2m where l is the angular momentum of the ring about its axis of rotation.
View SolutionA solid sphere of radius r and mass m rotates about an axis passing through its centre with angular velocity ω. Its K.E. Is
View SolutionA ring of radius 'r' and mass per unit length 'm' rotates with an angular velocity 'ω' in free space then tension will be :
View Solution
