A cylinder of cross-section area. A has two pistons of negligible mass separated by distances l loaded with spring of negligible mass. An ideal gas at temperature T1 is in the cylinder where the springs are relaxed. When the gas is heated by some means its temperature becomes T2 and the springs get compressed by l2 each . if P0 is atmospheric pressure and spring constant k=2P0Al, then find the ratio of T2 and T1 .
_S01_124_Q01.png)
The correct Answer is:4
Step by step video, text & image solution for A cylinder of cross-section area. A has two pistons of negligible mass separated by distances l loaded with spring of negligible mass. An ideal gas at temperature T_(1) is in the cylinder where the springs are relaxed. When the gas is heated by some means its temperature becomes T_(2) and the springs get compressed by (l)/(2) each . if P_(0) is atmospheric pressure and spring constant k=(2P_(0)A)/(l), then find the ratio of T_(2) and T_(1) . by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
A cylinder of cross-section area. A has two pistons of negligible mass separated by distances l loaded with spring of negligible mass. An ideal gas at temperature T1 is in the cylinder where the springs are relaxed. When the gas is heated by some means its temperature becomes T2 and the springs get compressed by l2 each . if P0 is atmospheric pressure and spring constant k=2P0Al, then find the ratio of T2 and T1 .
_S01_124_Q01.png)
View SolutionA 4.0 kg body, moving with a speed of 2.0m/s ,collides with a spring bumper of negligible mass and force constant 100Nm−1 .The maximum compression of the spring is ?
View Solution
Knowledge Check
An ideal monoatomic gas is confined in a horizontal cylinder by a spring loaded piston (as shown in the figure). Initially the gas is at temperature T1, pressure P1 and volume V1and the spring is in its relaxed state. The gas is tehn heated very slowly to temperature T2,pressure P2and volume V2. During this process the piston moves out by a distance x. Ignoring the friction between the piston and the cylinder, the correct statement (s) is (are)
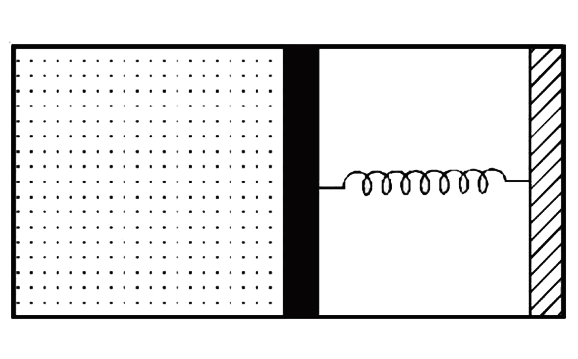 AIf V2=2V1andT2=3T1, then the o the energy stored in the spiring is 14P1V1BIf V2=2V1andT2=3T1, then the change in internal energy is 3P1V1CIf V2=3V1andT2=4T1, then the work done by the gas is 73P1V1DIf V2=3V1andT2=4T1, then the heat supplied to the gas is 176P1V1
AIf V2=2V1andT2=3T1, then the o the energy stored in the spiring is 14P1V1BIf V2=2V1andT2=3T1, then the change in internal energy is 3P1V1CIf V2=3V1andT2=4T1, then the work done by the gas is 73P1V1DIf V2=3V1andT2=4T1, then the heat supplied to the gas is 176P1V1When a mass of 5 kg is suspended from a spring of negligible mass and spring constant K, it oscillates with a periodic time 2π. If the mass is removed, the length of the spring will decrease by
AgK metreBg metreC2π metreDmK metreWhen an ideal gas at pressure P, temperature T and volume V is isothermally compressed to V/n. its pressure becomes Pi. If the gas is compressed adiabatically to V/n, its pressure becomes Pa. The ratio Pi/Pa is: ( l=CP/CV)
A1BnCn'lDn(1−l)
A monoatomic ideal gas, initially at temperature T1 is enclosed in a cylinder fitted with a frictionless piston. The gas is allowed to expand adiabatically to a temperature T2 by releasing the piston suddenly. If L1 and L2 are the lengths of the gas column. before and after expansion respectively, then T1T2 is given by
View SolutionA cylinder is fitted with a piston, beneath which is a spring, as in the figure. The cylinder is open at the top. Friction is absent. The spring is 3600N/m. The piston has a negligible mass and a radius of 0.025m (a) when air beneath the piston is conpletely pumped out, how much does the atmospheric pressure cause the spring to compress? (b) How much work does the atmospheric pressure do in compressing the spring?
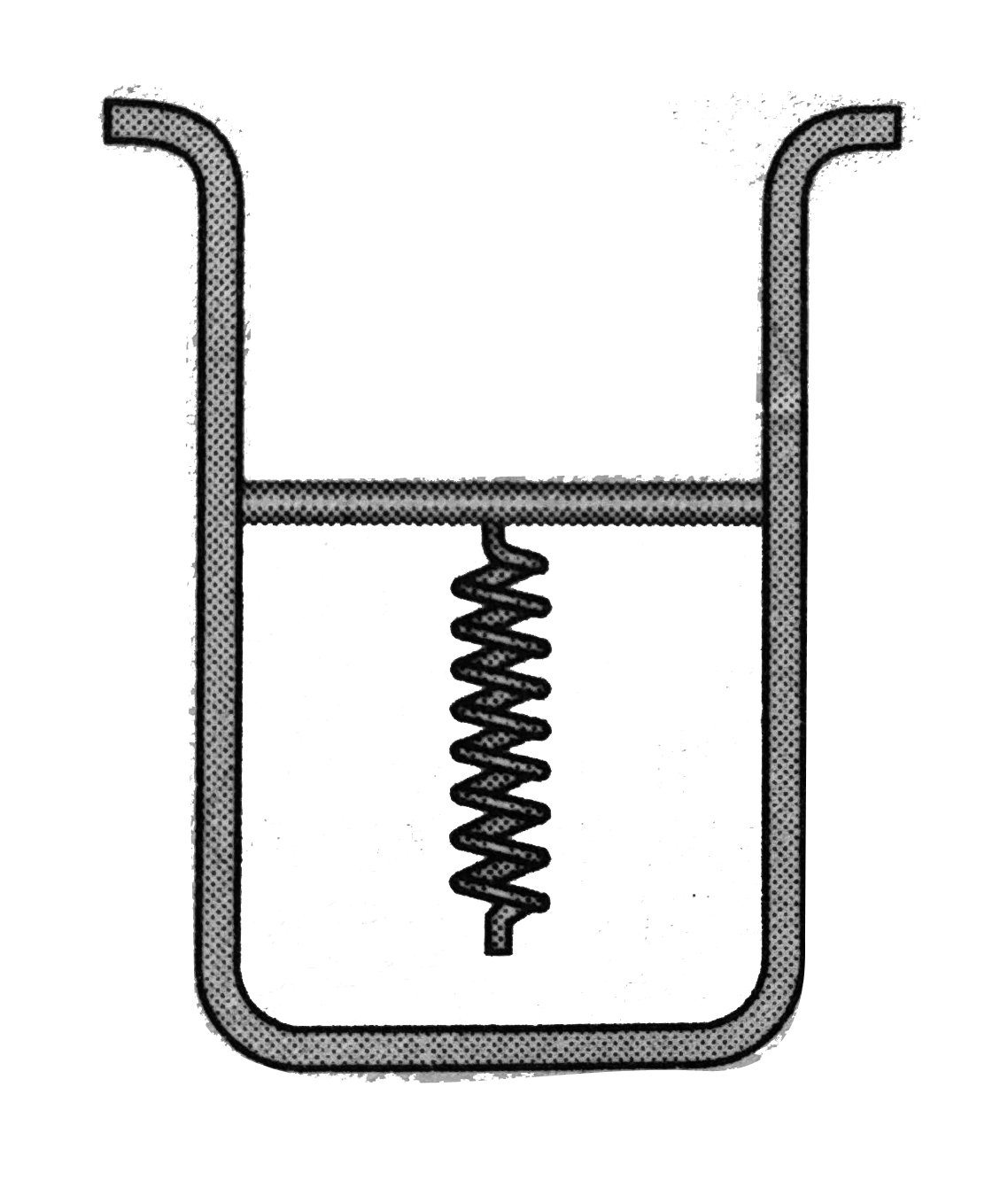
View SolutionTwo masses m and 2m are attached to two ends of an ideal spring as shown in figure. When thye spring is in the compressed state, the energy of the spring is 60 J, if the spring is released, then at its natural length

View SolutionIf a spring extends by x on loading, then the energy stored by the spring is (if T is tension in the spring and k is spring constant)
View SolutionA vertical spring has a time period of oscillations of T1 with a load m1 at its end. Its time period is T2 when the load is replaced by another of mass m2. The force constant of the spring is
View Solution
