
Four identical metal butterflies are hanging from a light string of length 5l at equally placed points as shown. The ends of the string are attached to horizontal fixed support. The middle section of the string is horizontal . The relation between the angle θ1 and θ2 is given by
sinθ1=2sinθ2
2cosθ1=sinθ2
tanθ1=2tanθ2
θ2<θ1 and no other conclusion can be derived.
The correct Answer is:C

T1sinθ1=2mg
T2sinθ2=mg
T1cosθ1=T2cosθ2
2mgcotθ1=mgcotθ2
⇒tanθ1=2tanθ2
Similar Questions
A stone hanging from a massless string of length 15 m is projected horizontally with speed 12ms−1. The speed of the particle at the point where the tension in the string is equal to the weight of the particle, is close to
View SolutionA heavy particle hanging from a string of length I is projected horizontally with speed √gl . Find the speed of the particle at the point where the tension in the string equals weight of the particle.
View SolutionA particle is rotated in a vertical circle by connecting it to a string of length l and keeping the other end of the string fixed. The minimum speed of the particle when the string is horizontal for which the particle will complete the circle is
View SolutionIn a simple pendulum, the breaking strength of the string is double the weight of the bob. The bob is released from rest when the string is horizontal. The string breaks when it makes an angle θ with the vertical.
View SolutionThe ring shown in fig. is given a constant horizontal acceleration (a0=g√3). The maximum deflection of the string from the vertical is θ0. Then
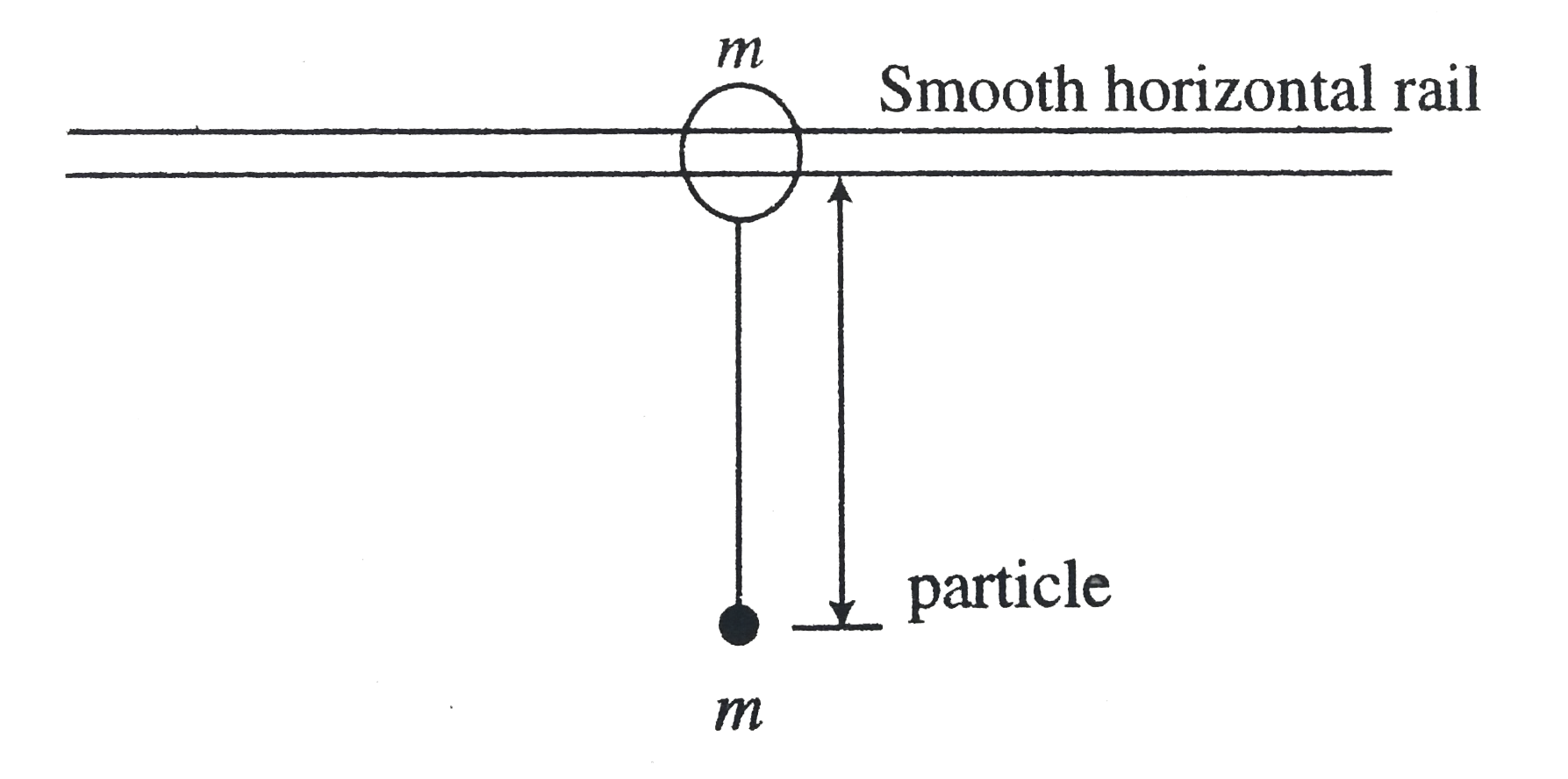
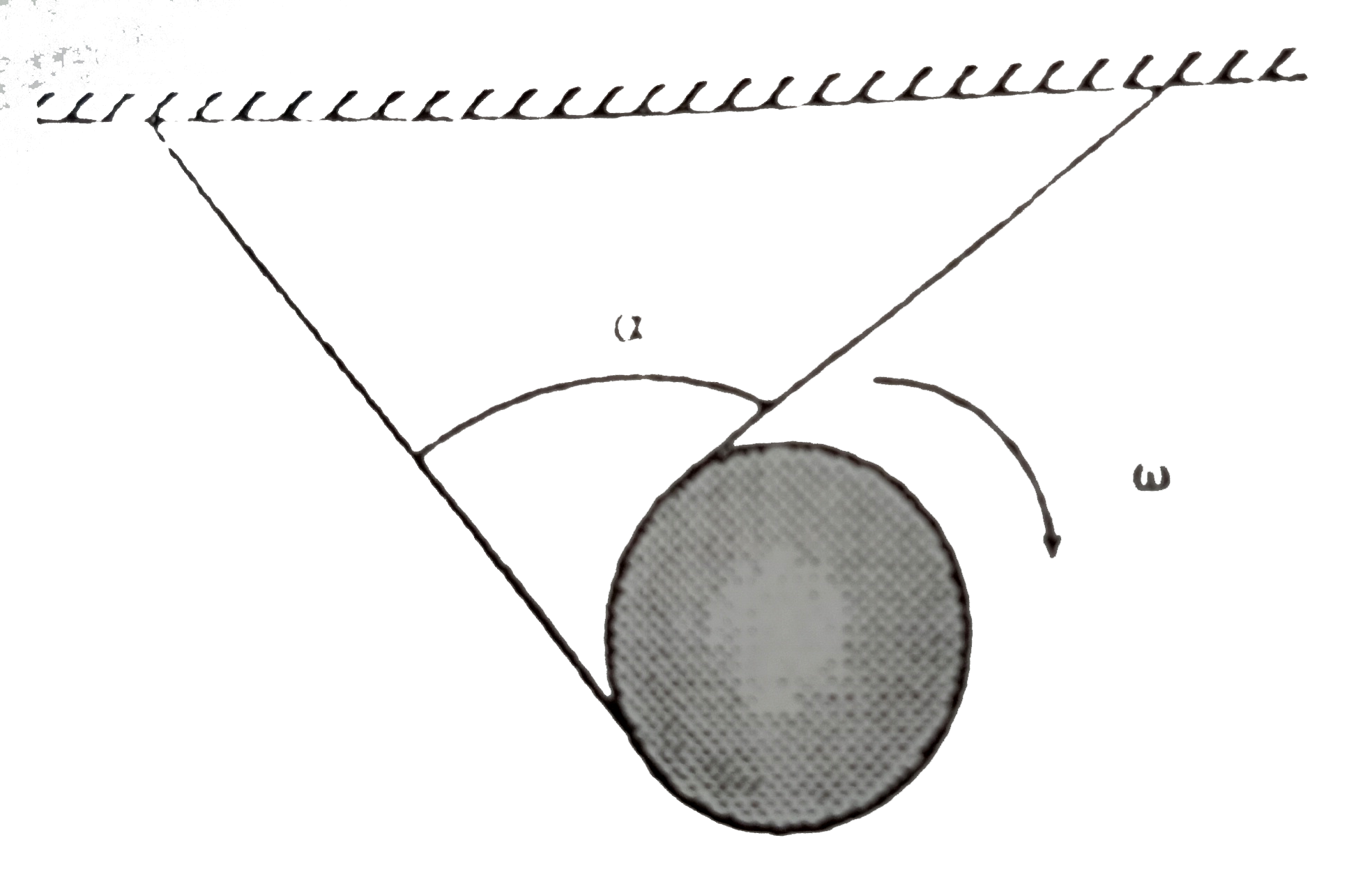
View SolutionA heavy disc with radius R is rolling down hanging on two non-stretched string wound around the disc very tightly. The free ends of the string are attached to a fixed horizontal support. The strings are always tensed during the motion. At some instant, the angular velocity of the disc is ω, and the angle between the strings is α. Find the velocity of centre of mass of the disc at this moment
View SolutionA stone hanging from a massless string of length 15m is projected horizontally with speed √147ms−1 Then the Speed of the particle, at the point where tension in string equals the weight of particle, is
View SolutionA particle is suspended by a light vertical inelastic string of length 1 from a fixed support. At its equilbrium position it is projected horizontally with a speed √6gl. Find the ratio of the tension in the string in its horizontal position to that in the string when the particle is vertically above the point of support.
View Solution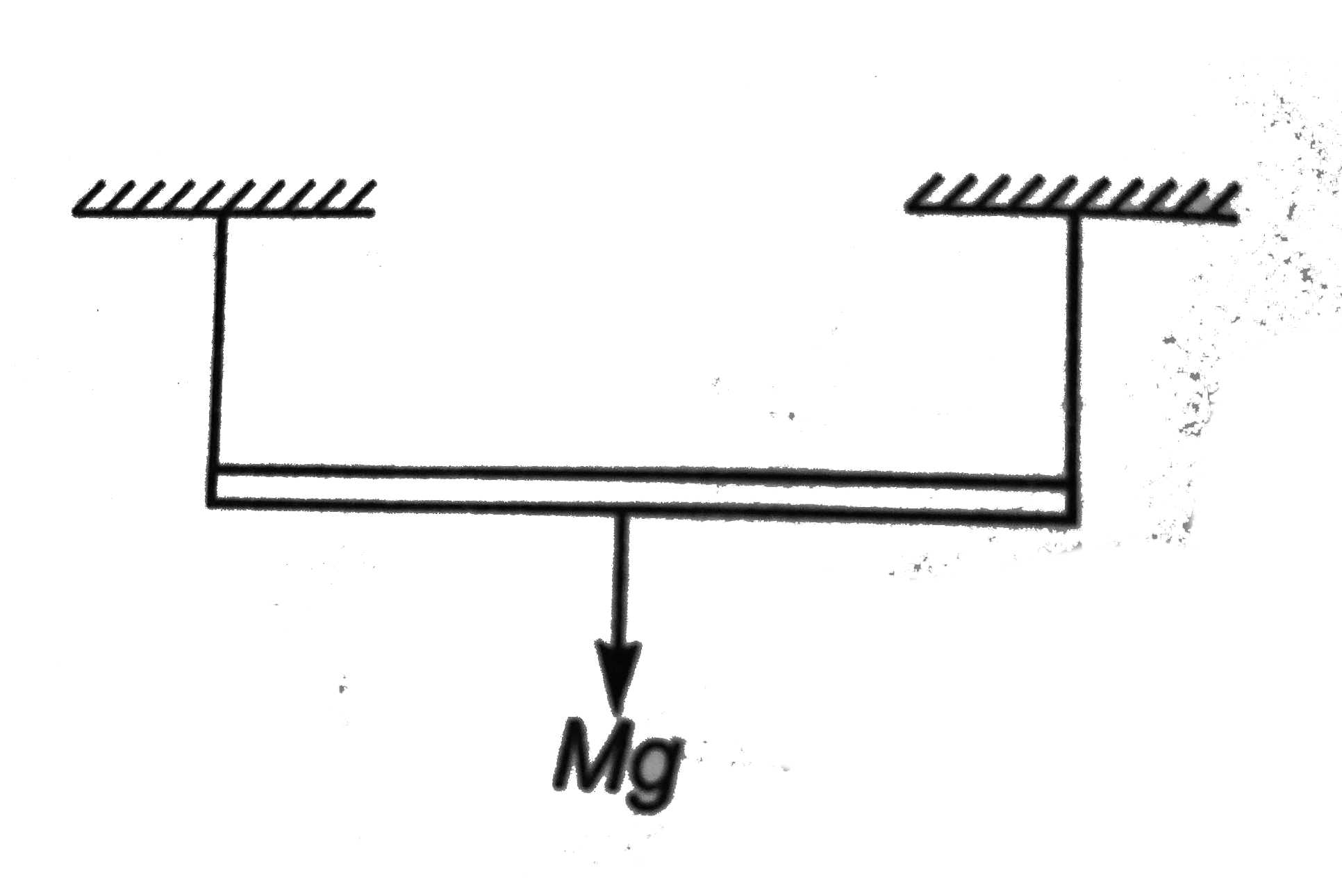
A rod is supported horizontally by means of two strings of equal length as shown in figure. If one of the string is cut. Then tension in other string at the same instant will.
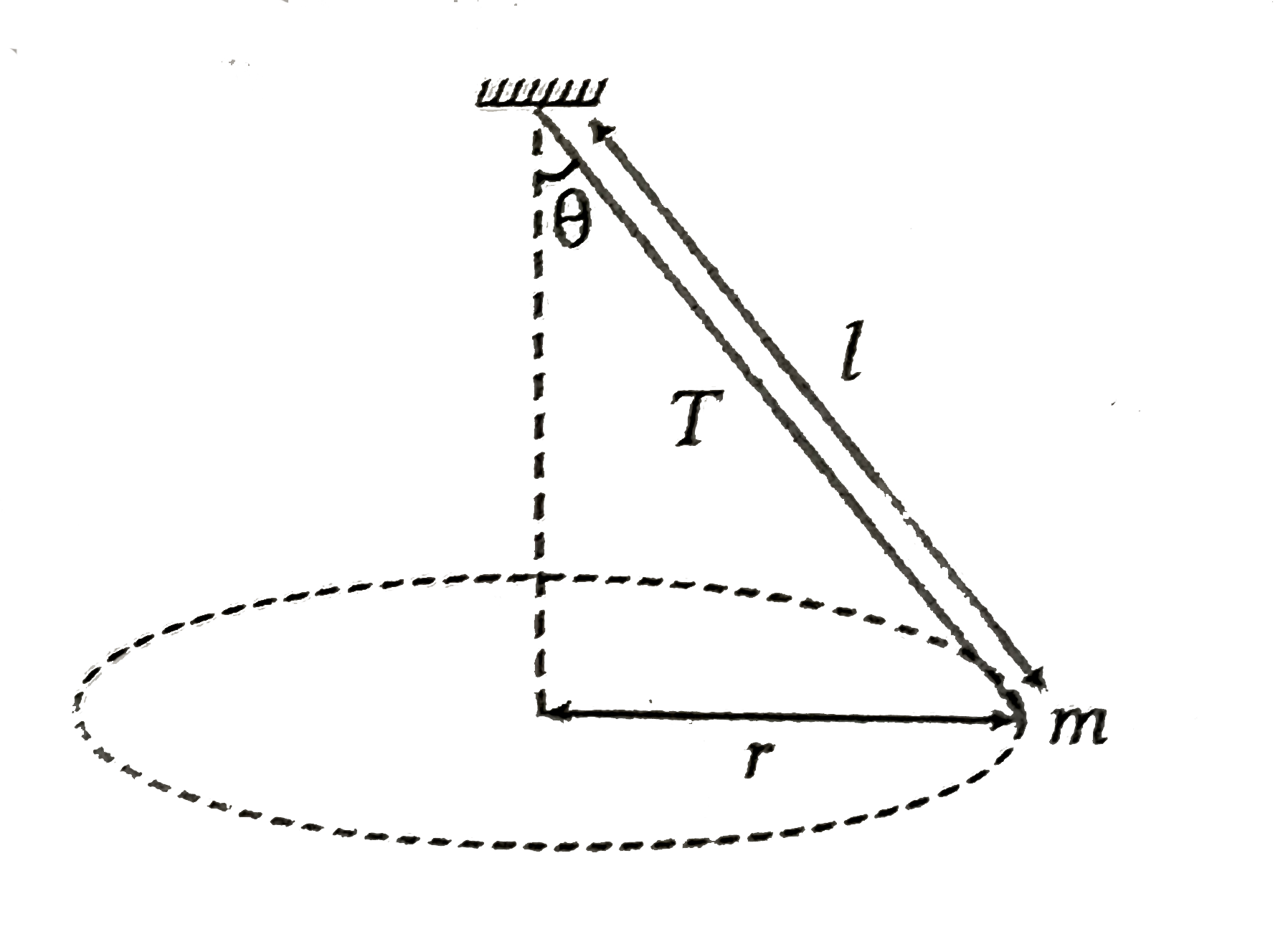
View SolutionA string of lenth l fixed at one end carries a mass m at the other end. The strings makes 2πrevs−1 around the axis through the fixed end as shown in the figure, the tension in the string is
View Solution
