The adjacent sides of a rectangle with given perimeter as 100 cm and enclosing maximum area are
10 cm and 40 cm
20 cm and 30 cm
25 cm and 25 cm
15 cm and 35 cm
The correct Answer is:C
To solve the problem of finding the adjacent sides of a rectangle with a given perimeter of 100 cm that encloses the maximum area, we can follow these steps:
Step 1: Understand the perimeter of the rectangle
The perimeter P of a rectangle is given by the formula:
P=2A+2B
where A and B are the lengths of the adjacent sides of the rectangle.
Step 2: Set up the equation for the perimeter
Given that the perimeter is 100 cm, we can write:
2A+2B=100
Dividing the entire equation by 2 gives:
A+B=50
This is our first relation.
Step 3: Express one variable in terms of the other
From the equation A+B=50, we can express A in terms of B:
A=50−B
Step 4: Write the area of the rectangle
The area A of the rectangle is given by:
Area=A×B
Substituting A=50−B into the area formula gives:
Area=(50−B)×B=50B−B2
Step 5: Differentiate the area function
To find the maximum area, we need to differentiate the area function with respect to B:
d(Area)dB=50−2B
Step 6: Set the derivative equal to zero
To find the critical points, set the derivative equal to zero:
50−2B=0
Solving for B gives:
2B=50⇒B=25
Step 7: Find the corresponding value of A
Now, substitute B=25 back into the equation A=50−B:
A=50−25=25
Step 8: Verify that this gives a maximum area
To confirm that this critical point gives a maximum area, we can check the second derivative:
d2(Area)dB2=−2
Since the second derivative is negative, this indicates that we have a maximum area at B=25.
Conclusion
Thus, the adjacent sides of the rectangle that enclose the maximum area with a perimeter of 100 cm are:
A=25cm,B=25cm
The perimeter P of a rectangle is given by the formula:
P=2A+2B
where A and B are the lengths of the adjacent sides of the rectangle.
Given that the perimeter is 100 cm, we can write:
2A+2B=100
Dividing the entire equation by 2 gives:
A+B=50
This is our first relation.
From the equation A+B=50, we can express A in terms of B:
A=50−B
The area A of the rectangle is given by:
Area=A×B
Substituting A=50−B into the area formula gives:
Area=(50−B)×B=50B−B2
To find the maximum area, we need to differentiate the area function with respect to B:
d(Area)dB=50−2B
To find the critical points, set the derivative equal to zero:
50−2B=0
Solving for B gives:
2B=50⇒B=25
Now, substitute B=25 back into the equation A=50−B:
A=50−25=25
To confirm that this critical point gives a maximum area, we can check the second derivative:
d2(Area)dB2=−2
Since the second derivative is negative, this indicates that we have a maximum area at B=25.
Thus, the adjacent sides of the rectangle that enclose the maximum area with a perimeter of 100 cm are:
A=25cm,B=25cm
Similar Questions
Knowledge Check
The adjacent sides of a parallelogram are in the ratio 5:3. if its perimeter is 96cm, find the sides of the parallelogram.
A30cm and 18cm.B55cm and 18cm.C26cm and 10cm.D37cm and 28cm.
Find the breadth of the rectangle whose perimeter is 360cm and whose length is 100cm.
View SolutionThe sides of a rectangle are in the ratio 2:3, and its perimeter is 20cm. Draw the rectangle.
View SolutionIf the lengths and breadths of rectangles are positive integral values, find the dimensions of all possible rectangles with perimeter 18cm. Also, find their respective areas.
View SolutionThe perimeter of a rectangle is 30 cm. If the lengths of the sides of the rectangles are natural numbers, find all rectangles with same perimeter and also find their respective areas.
View SolutionIn the adjoining figure, all the adjacent sides are at right angles. Find:
(i) the perimeter of the figure.
(ii) the area of the figure.
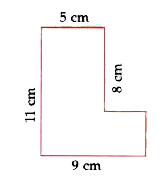
View SolutionThe side of a square is 70cm. Find its area and perimeter.
View SolutionThe sides of a rectangle are in the 4:5. Find its sides if the perimeter is 90cm.
View Solution
