Young's modulus of a material has the same units as (A) pressure (B) strain (C) compressibility (D) Force
pressure
strain
compressibility
force
The correct Answer is:A
To determine which of the given options has the same units as Young's modulus, we first need to understand the definition and units of Young's modulus.
Step 1: Definition of Young's Modulus
Young's modulus (Y) is defined as the ratio of stress to strain.
Step 2: Understanding Stress and Strain
- Stress is defined as the force (F) applied per unit area (A):
Stress=FA
- Strain is defined as the change in length (ΔL) divided by the original length (L):
Strain=ΔLL
Step 3: Units of Stress
The units of stress can be derived as follows:
- Force (F) has units of Newton (N), which is equivalent to kg⋅m/s2.
- Area (A) has units of m2.
Thus, the units of stress are:
Units of Stress=ForceArea=Nm2=kg⋅m/s2m2=kgm⋅s2
Step 4: Units of Strain
Strain is a dimensionless quantity since it is a ratio of two lengths:
Units of Strain=LL=1(dimensionless)
Step 5: Units of Young's Modulus
Now, substituting the units of stress and strain into the formula for Young's modulus:
Y=StressStrain=FAStrain=Nm21=Nm2
Thus, the units of Young's modulus are:
Units of Young's Modulus=kgm⋅s2⋅1m2=kgm⋅s2=Pressure
Step 6: Comparison with Given Options
Now, we compare the units of Young's modulus with the options given:
- (A) Pressure: Units are kgm⋅s2 (same as Young's modulus)
- (B) Strain: Dimensionless (not the same)
- (C) Compressibility: Different units (not the same)
- (D) Force: Units are kg⋅m/s2 (not the same)
Conclusion
The correct answer is:
(A) Pressure
Young's modulus (Y) is defined as the ratio of stress to strain.
- Stress is defined as the force (F) applied per unit area (A):
Stress=FA
- Strain is defined as the change in length (ΔL) divided by the original length (L):
Strain=ΔLL
The units of stress can be derived as follows:
- Force (F) has units of Newton (N), which is equivalent to kg⋅m/s2.
- Area (A) has units of m2.
Units of Stress=ForceArea=Nm2=kg⋅m/s2m2=kgm⋅s2
Strain is a dimensionless quantity since it is a ratio of two lengths:
Units of Strain=LL=1(dimensionless)
Now, substituting the units of stress and strain into the formula for Young's modulus:
Y=StressStrain=FAStrain=Nm21=Nm2
Thus, the units of Young's modulus are:
Units of Young's Modulus=kgm⋅s2⋅1m2=kgm⋅s2=Pressure
Now, we compare the units of Young's modulus with the options given:
- (A) Pressure: Units are kgm⋅s2 (same as Young's modulus)
- (B) Strain: Dimensionless (not the same)
- (C) Compressibility: Different units (not the same)
- (D) Force: Units are kg⋅m/s2 (not the same)
The correct answer is:
(A) Pressure
Topper's Solved these Questions
- CENGAGE PHYSICS DPPBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENGAGE PHYSICS DPPEXERCISE - subjective type
51 Videos
- CENGAGE PHYSICS DPPBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENGAGE PHYSICS DPPEXERCISE - Multiple correct Answer Type
54 Videos
- CAPACITOR AND CAPACITANCEBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CAPACITOR AND CAPACITANCEEXERCISE - Integer
5 Videos
- COULOMB LAW AND ELECTRIC FIELDBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - COULOMB LAW AND ELECTRIC FIELDEXERCISE - Single Answer Correct Type
22 Videos
Similar Questions
The Young's modulus of the material of a wire is equal ot the
View SolutionIf the Young's modulus of the material is 3 times its modulus of rigidity, then its volume elasticity will be
View SolutionA uniform wire of length 6m and a cross-sectional area 1.2 cm2 is stretched by a force of 600N . If the Young's modulus of the material of the wire 20×1010Nm−2 , calculate (i) stress (ii) strain and (iii) increase in length of the wire.
View SolutionA wire havinga length L and cross- sectional area A is suspended at one of its ends from a ceiling . Density and young's modulus of material of the wire are ρ and Y, respectively. Its strain energy due to its own weight is ρ2g2AL3αY. Find the vqalue of α
View SolutionThe Young's modulus of a wire Y. If the energy per unit volume is E, then the strain will be
View SolutionWhen a force is applied at one end of an elastic wire, it produce a strain E in the wire. If Y is the Young's modulus of the material of the wire, the amount of energy stored per unit volume of the wire is given by
View SolutionShow that the Young.s modulus of the material of a wire is equal to the stress required to double the initial length of the wire. Assume that Hookes law holds good throughout the process.
View SolutionThe stress-strain graphs for two materials A and B are shown in the figure. The graphs are drawn to the same scale.
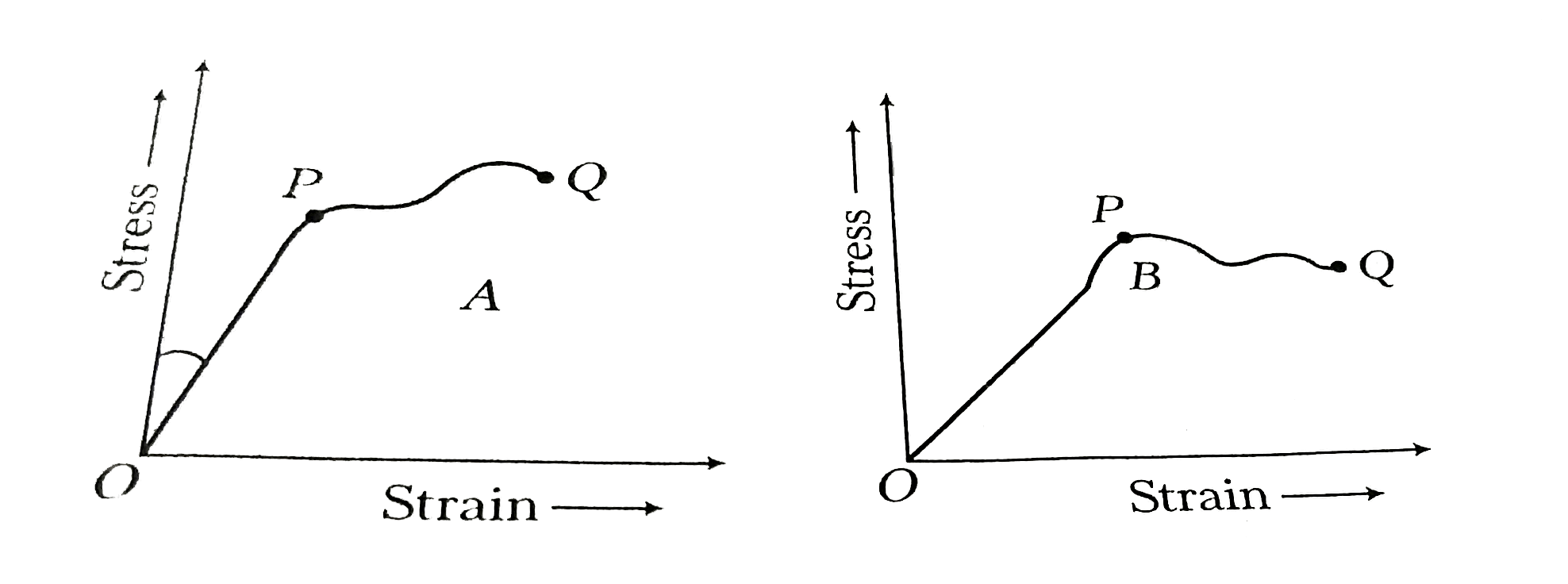
(a) Which material has a greater Young's modulus ?
(b) Which material is more ductile ?
(c) Which material is more brittle?
(d) Which of the two is the stronger material ?
View SolutionIf s is stress and Y is Young's modulus of material, the energy stored per unit volum is
View SolutionDefine stress, strain and Young's modulus of an elastic material. Describe an experiment for measuring the Young's modulus of a material in the form of a wire.
View Solution
