A sound wave of wavelength λ travels towards the right horizontally with a velocity V. It strikes and reflects from a vertical plane surface , travelling at a speed v towards the left . The number of positive crests striking in a time interval of 3s on the wall is
3(V+v)/λ
3(V−v)/λ
(V+v)/3λ
(V−v)/3λ
The correct Answer is:A
To solve the problem of finding the number of positive crests striking a vertical plane surface in a time interval of 3 seconds, we can follow these steps:
Step 1: Understand the Problem
We have a sound wave traveling towards a wall and reflecting off it. We need to find out how many positive crests (or waves) hit the wall in a given time period.
Step 2: Identify Given Data
- Wavelength of the sound wave: λ
- Speed of the sound wave towards the wall: V
- Speed of the sound wave after reflection: v
- Time interval: t=3s
Step 3: Calculate the Relative Velocity
When the wave strikes the wall, it travels towards the wall with speed V and reflects back with speed v. Therefore, the effective speed at which the wave approaches the wall is the sum of these two speeds:
Relative velocity=V+v
Step 4: Calculate the Distance Traveled in 3 Seconds
The total distance traveled by the wave in 3 seconds can be calculated using:
Distance=Relative velocity×Time
Substituting the values, we get:
Distance=(V+v)×3
Step 5: Calculate the Number of Waves
The number of waves (or positive crests) striking the wall can be calculated by dividing the total distance traveled by the wavelength λ:
Number of waves=Distanceλ=(V+v)×3λ
Final Answer
Thus, the number of positive crests striking the wall in a time interval of 3 seconds is:
Number of positive crests=3(V+v)λ
---
We have a sound wave traveling towards a wall and reflecting off it. We need to find out how many positive crests (or waves) hit the wall in a given time period.
- Wavelength of the sound wave: λ
- Speed of the sound wave towards the wall: V
- Speed of the sound wave after reflection: v
- Time interval: t=3s
When the wave strikes the wall, it travels towards the wall with speed V and reflects back with speed v. Therefore, the effective speed at which the wave approaches the wall is the sum of these two speeds:
Relative velocity=V+v
The total distance traveled by the wave in 3 seconds can be calculated using:
Distance=Relative velocity×Time
Substituting the values, we get:
Distance=(V+v)×3
The number of waves (or positive crests) striking the wall can be calculated by dividing the total distance traveled by the wavelength λ:
Number of waves=Distanceλ=(V+v)×3λ
Thus, the number of positive crests striking the wall in a time interval of 3 seconds is:
Number of positive crests=3(V+v)λ
Topper's Solved these Questions
- SUPERPOSITION AND STANDING WAVESBook:CENGAGE PHYSICS ENGLISHChapter:SUPERPOSITION AND STANDING WAVESExercise:Multiple
Explore 26 Videos
- SUPERPOSITION AND STANDING WAVESBook:CENGAGE PHYSICS ENGLISHChapter:SUPERPOSITION AND STANDING WAVESExercise:Assertion - Reasoning
Explore 6 Videos
- SUPERPOSITION AND STANDING WAVESBook:CENGAGE PHYSICS ENGLISHChapter:SUPERPOSITION AND STANDING WAVESExercise:Subjective
Explore 24 Videos
- SOUND WAVES AND DOPPLER EFFECTBook:CENGAGE PHYSICS ENGLISHChapter:SOUND WAVES AND DOPPLER EFFECTExercise:Integer
Explore 16 Videos
- THERMODYNAMICSBook:CENGAGE PHYSICS ENGLISHChapter:THERMODYNAMICSExercise:24
Explore 1 Video
Similar Questions
A sound wave of frequency f travels horizontally to the right . It is teflected from a larger vertical plane surface moving to left with a speed v. the speed of sound in medium is c
(a) The number of waves striking the surface per second is f(c+υ)c
(b) The wavelength of reflected wave is c(c−υ)f(c+υ)
(c) The frequency of the reflected wave is f((c+υ))(c+υ)
(d) The number of beats heard by a stationary listener to the left of the reflecting surface is υfc−υ
View Solution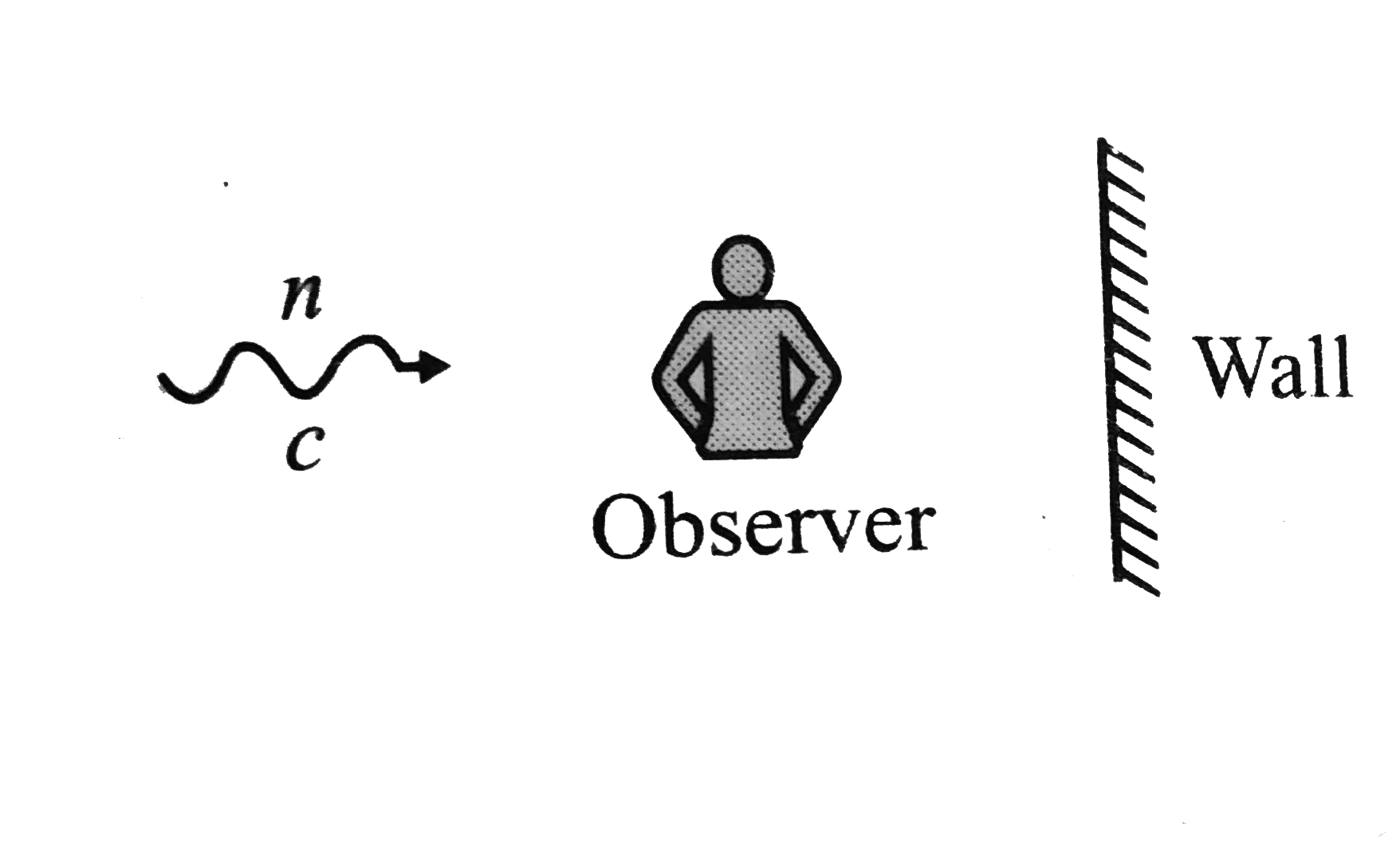
A sound wave of frequency n travels horizontally to the right with speed with speed c. It is reflected from a broad wall moving to the left with speed v. The number of beats heard by a stationary observer to the left of the wall is
View Solution
Knowledge Check
A ball of mass m travelling horiozntally with velocity v strikes a massive vertical wall and rebounds back along its original direction with no change in speed. What is the magnitude of the impulse delivered by the wall to the ball?
A0B12mvCmvD2mv

A sound wave of frequency n travels horizontally to the right with speed with speed c. It is reflected from a broad wall moving to the left with speed v. The number of beats heard by a stationary observer to the left of the wall is
View SolutionA source of sound of frequency 256 Hz moves rapidly towards a wall with a velocity of 5ms−1. How many beats per second will be heard if sound travels at a speed of 330 m/s?
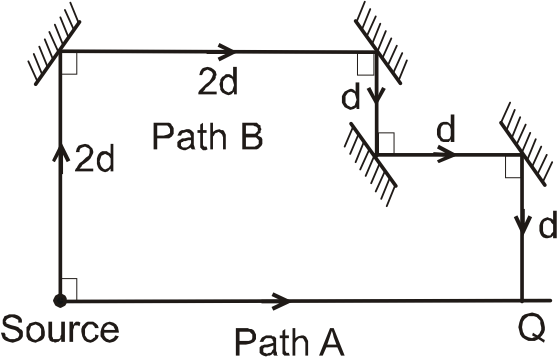
View SolutionA sound source emits two sinusoidal sound waves, both of wavelength λ, along paths A and B as shown in figure.The sound travelling along path B is reflected from five surfaces as shown and then merges at point Q, producing minimum intensity at that point.The minimum value of d in terms of λ is :
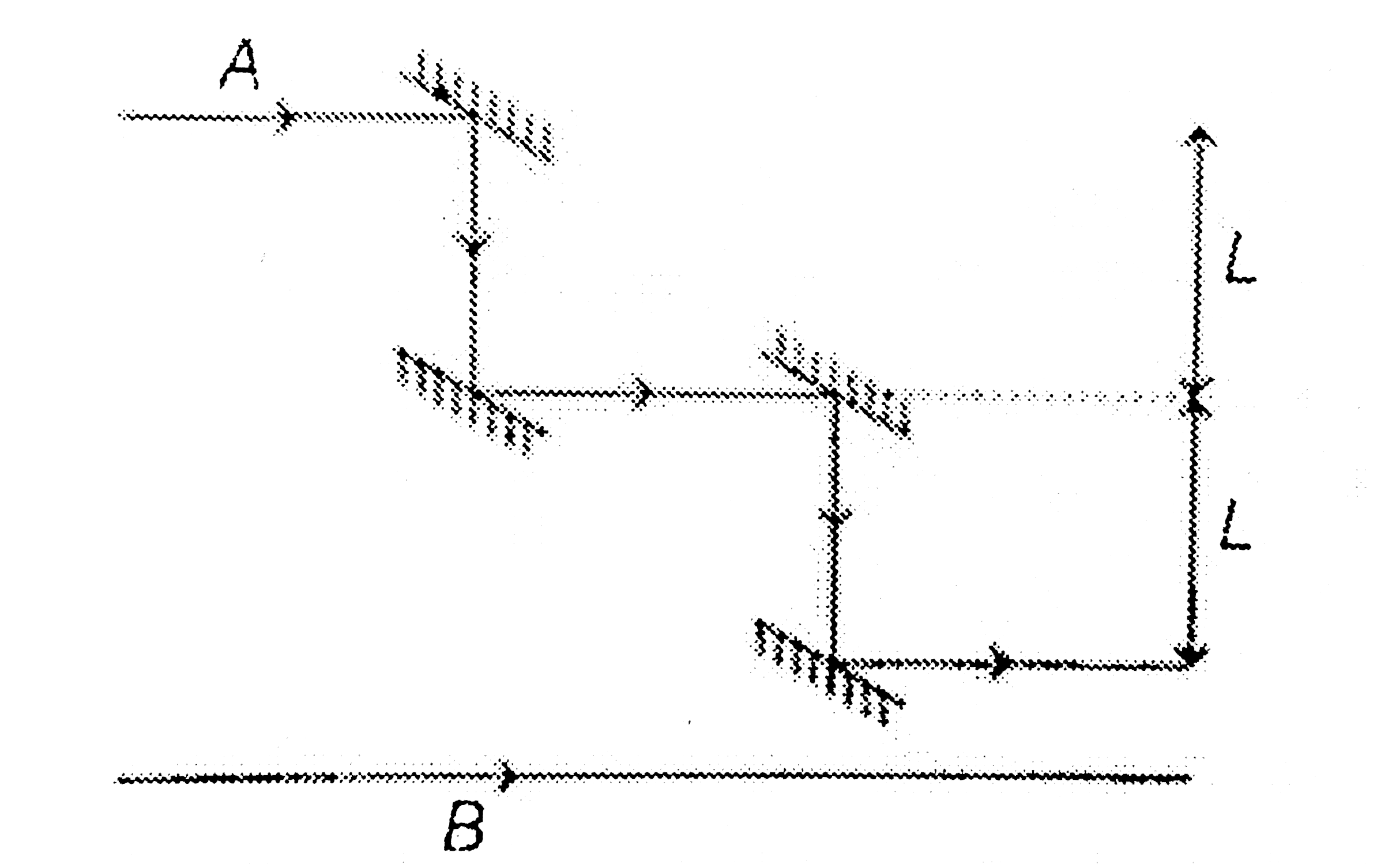
View SolutionIn the figure shown, light waves A and B both of wavelength λ are initially in phase and travelling rightward as incident by the two rays. Wave A is reflected from four parallel surfaces but ends up travelling in original direction. The possible value(s) of distance L in terms of wavelength λ in figure which put A and B exactly out of phase with each other after all the reflected is/are
View SolutionA ball is projected horizontally from an inclined plane with a velocity V, as shown in the figure. It will strike the plane after a time

View SolutionA sound wave of frequency f propagating through air with a velocity c, is reflected from a surface which is moving away from the source with a constant speed v. Find the frequency of the reflected wave, measured by the observer at the position of the source.
View SolutionA transverse sine wave of amplitude 10 cm and wavelength 200 cm travels from left to right along a long horizontal stretched, string with a speed of 100 cm/s. Take the origin at left end of the string. At time t = 0 the left end of the string is at the origin and is moving downward. Then the equation of the wave will be ( in CGS system )
View Solution