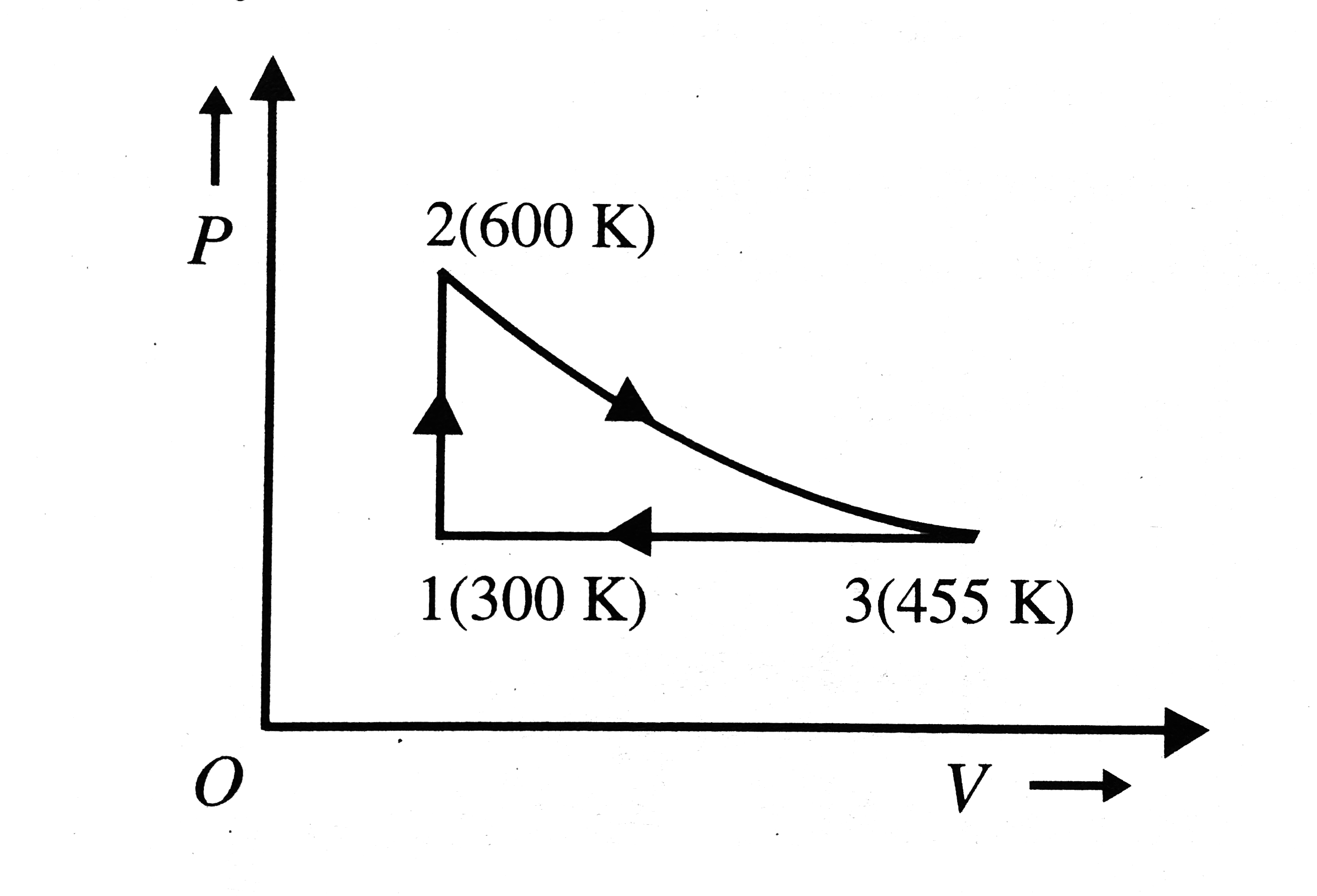
A reversible heat engine carries of an ideal monatomic gas around the cycle , as shown in the diagram. The process is adiabatic. Call the processes and as and and the heat , change in internal energy , and work done respectively. The temperature at are and . Indicate the pressure and volume at and by and respectively. Assume that intially pressure
Which of the following represents the correct values for the quantities indicated ?
The correct Answer is:B
To solve the problem step by step, we will analyze the reversible heat engine cycle involving an ideal monatomic gas. We will find the pressure and volume at points A, B, and C, given the temperatures and the initial pressure at point A.
Step 1: Identify the Given Values
- Temperature at point A, T1=300K
- Temperature at point B, T2=600K
- Temperature at point C, T3=455K
- Initial pressure at point A, P1=1.00atm
- The gas is monatomic and behaves ideally.
Step 2: Use the Ideal Gas Law
The ideal gas law is given by:
PV=nRT
where n is the number of moles, R is the ideal gas constant, and T is the temperature in Kelvin.
Since we have 1 mole of gas, we can express the pressure and volume at each point using the ideal gas law.
Step 3: Calculate Pressure at Point B
The process from A to B is isochoric (constant volume), and we can relate the pressures and temperatures at points A and B using the ratio:
P1P2=T1T2
Substituting the known values:
1atmP2=300K600K
Cross-multiplying gives:
P2=2atm
Step 4: Calculate Pressure at Point C
For the adiabatic process from B to C, we can use the relation for adiabatic processes for an ideal gas:
TBTC=(PBPC)γ−1γ
For a monatomic gas, γ=53. Rearranging gives:
PC=PB(TCTB)γγ−1
Substituting the known values:
PC=2atm(455K600K)5/32/3=2atm(455600)52
Calculating this gives:
PC≈1.5atm
Step 5: Calculate Volumes at Each Point
Using the ideal gas law, we can find the volumes at each point:
- At point A:
VA=nRT1P1=1⋅0.0821⋅3001≈24.63L
- At point B:
VB=nRT2P2=1⋅0.0821⋅6002≈24.63L
- At point C:
VC=nRT3PC=1⋅0.0821⋅4551.5≈24.63L
Summary of Results
- PA=1atm
- PB=2atm
- PC≈1.5atm
- VA≈24.63L
- VB≈24.63L
- VC≈24.63L
Conclusion
The correct values for the quantities indicated are:
- P1=1atm
- P2=2atm
- P3≈1.5atm
- Volumes remain approximately constant due to the nature of the processes.
- Temperature at point A, T1=300K
- Temperature at point B, T2=600K
- Temperature at point C, T3=455K
- Initial pressure at point A, P1=1.00atm
- The gas is monatomic and behaves ideally.
The ideal gas law is given by:
PV=nRT
where n is the number of moles, R is the ideal gas constant, and T is the temperature in Kelvin.
The process from A to B is isochoric (constant volume), and we can relate the pressures and temperatures at points A and B using the ratio:
P1P2=T1T2
Substituting the known values:
1atmP2=300K600K
Cross-multiplying gives:
P2=2atm
For the adiabatic process from B to C, we can use the relation for adiabatic processes for an ideal gas:
TBTC=(PBPC)γ−1γ
For a monatomic gas, γ=53. Rearranging gives:
PC=PB(TCTB)γγ−1
Substituting the known values:
PC=2atm(455K600K)5/32/3=2atm(455600)52
Calculating this gives:
PC≈1.5atm
Using the ideal gas law, we can find the volumes at each point:
- At point A:
VA=nRT1P1=1⋅0.0821⋅3001≈24.63L
- At point B:
VB=nRT2P2=1⋅0.0821⋅6002≈24.63L
- At point C:
VC=nRT3PC=1⋅0.0821⋅4551.5≈24.63L
- PA=1atm
- PB=2atm
- PC≈1.5atm
- VA≈24.63L
- VB≈24.63L
- VC≈24.63L
The correct values for the quantities indicated are:
- P1=1atm
- P2=2atm
- P3≈1.5atm
- Volumes remain approximately constant due to the nature of the processes.
Topper's Solved these Questions
- KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSBook:CENGAGE PHYSICS ENGLISHChapter:KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSExercise:Interger
Explore 11 Videos
- KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSBook:CENGAGE PHYSICS ENGLISHChapter:KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSExercise:Assertion-Reasoning
Explore 6 Videos
- KINETIC THEORY OF GASESBook:CENGAGE PHYSICS ENGLISHChapter:KINETIC THEORY OF GASESExercise:Compression
Explore 2 Videos
- LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONBook:CENGAGE PHYSICS ENGLISHChapter:LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONExercise:Single correct anwer type
Explore 14 Videos
Similar Questions
A reversible heat engine carries 1 mole of an ideal monatomic gas around the cycle 1-2-3-1. Process 1-2 takes place at constant volume, process 2-3 is adiabatic, and process 3-1 takes place at constant pressure. Complete the values for the heat ΔQ, the change in internal energy ΔU, and the work done Δ, for each of the three processes and for the cycle as a whole.
View SolutionOne mole of a monatomic ideal gas is taken along the cycle ABCA as shown in the diagram.

The ratio of specific heat in the process CA to the specific heat in the process BC is
View Solution
Knowledge Check
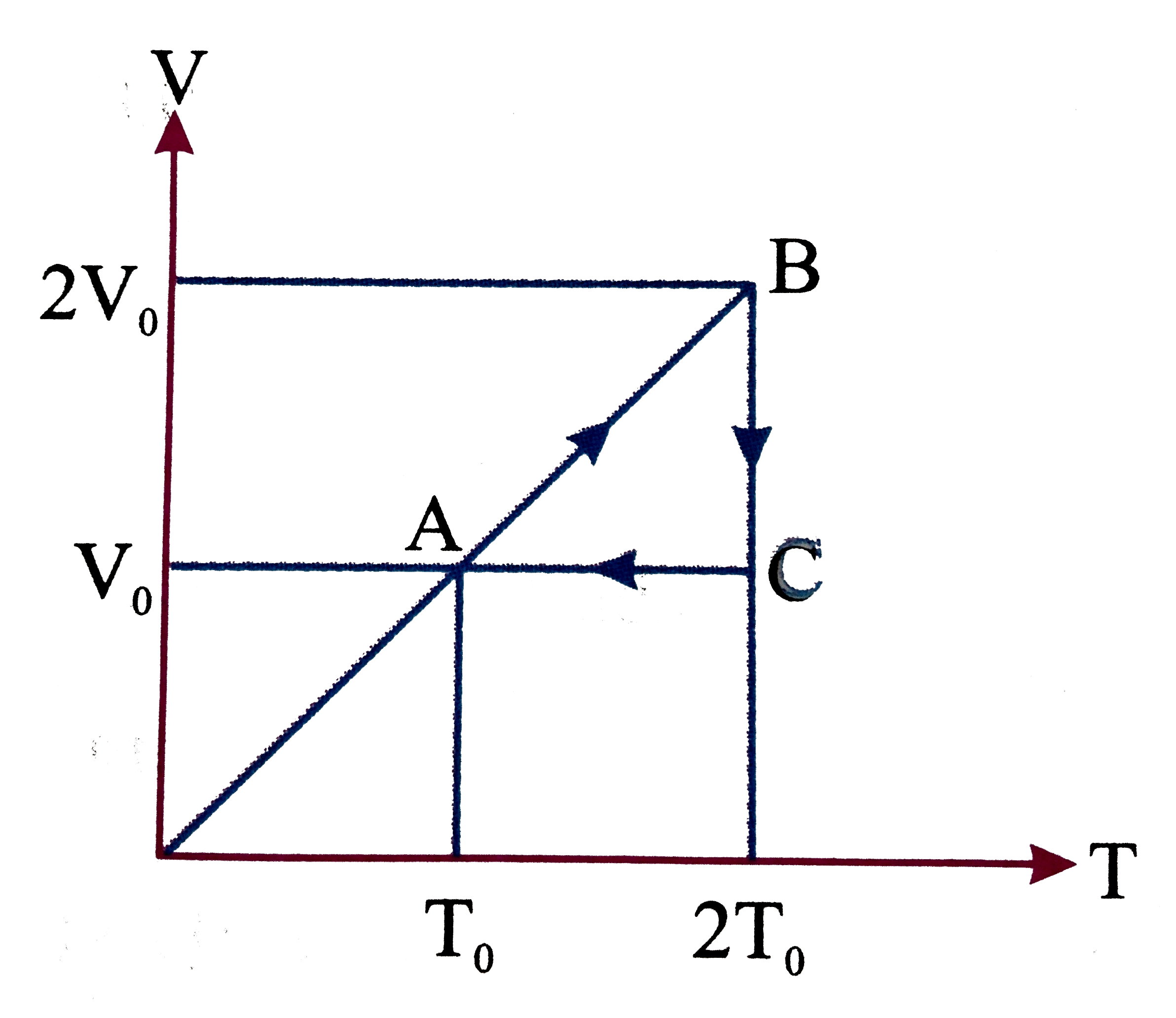
The cyclic process for 1 mole of an ideal gas is shown in the V-T diagram. The work done in AB, BC and CA respectively is
 A0,RT1Ln(V1V2),R(T1−T2)BR,(T1−T2)R,RT1In(V1V2)C0,RT2ln(V2V1),RT1V1(V1−V2)D0,RT2ln(V1)V2,R(T1−T2)
A0,RT1Ln(V1V2),R(T1−T2)BR,(T1−T2)R,RT1In(V1V2)C0,RT2ln(V2V1),RT1V1(V1−V2)D0,RT2ln(V1)V2,R(T1−T2)
One mole of a diatomic ideal gas undergoes a cyclic process ABC as shown in figure. The process BC is adiabatic. The temperature at A,B and C are 400K, 800K and 600K respectively. Choose the correct statement:
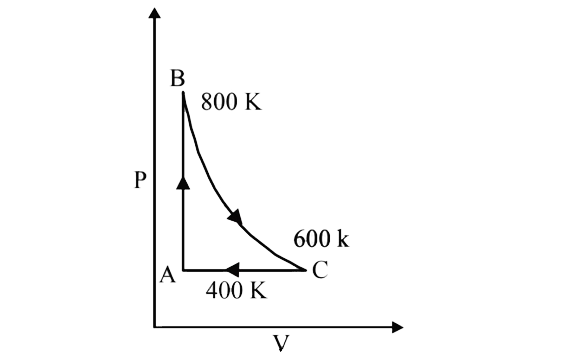
View SolutionOne mole of a diatomic ideal gas undergoes a cyclic process ABC as shown in figure. The process BC is adiabatic. The temperature at A,B and C are 400K, 800K and 600K respectively. Choose the correct statement:

View SolutionAn ideal monoatomic gas undergoes a cyclic process ABCA as shown in the figure. The ratio of heat absorbed during AB to the work done on the gas during BC id
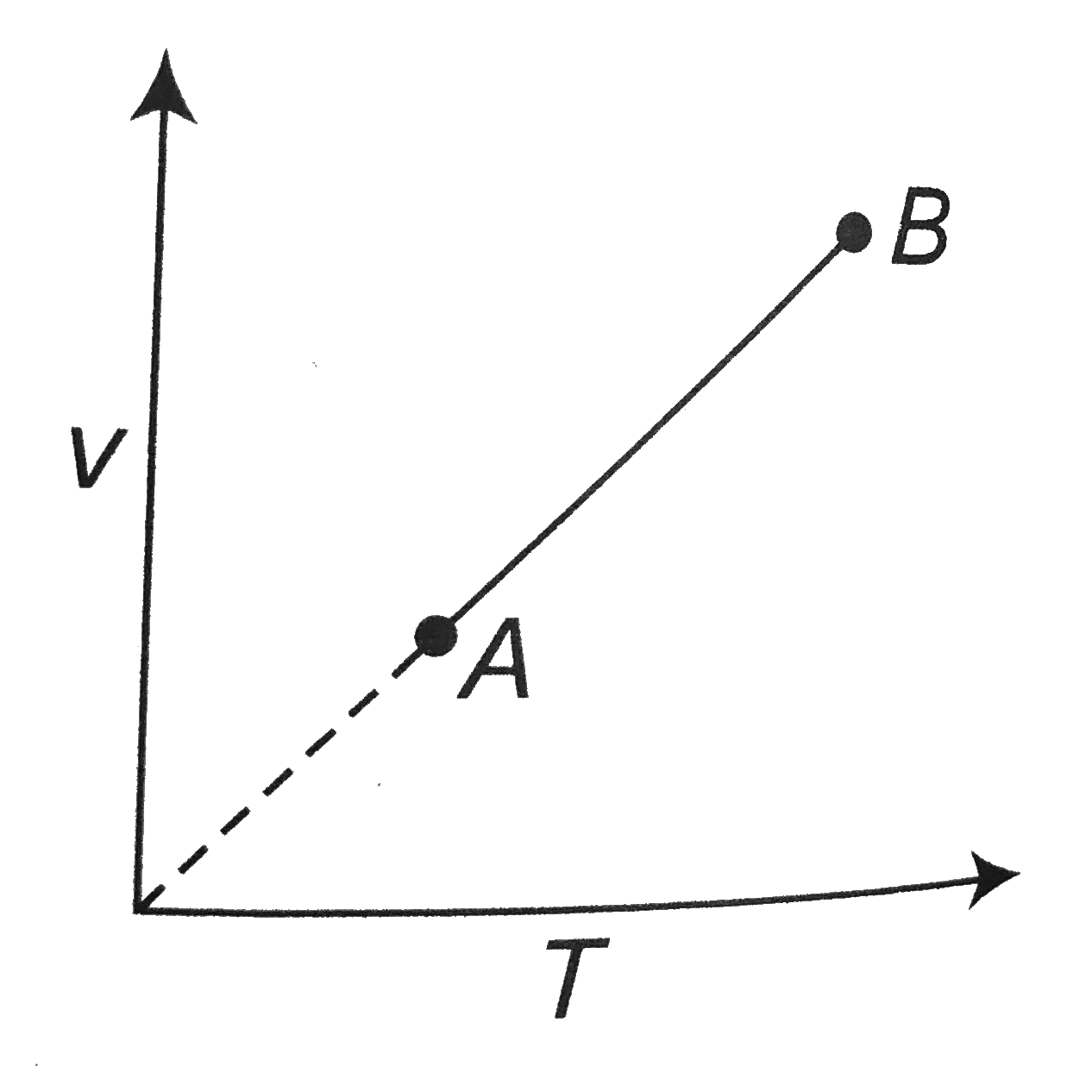
View SolutionAn ideal monoatomic gas undergoes the process AB as shown in the figure. If the heat supplied and the work done in the process are ΔQ and Delta Wrespectively.TheratioDeltaQ : DeltaW is
View SolutionOne mole of an ideal monoatomic gas undergoes a cyclic process, as shown in the figure. If the temperature of the gas at state 1 is 300 K and at state 4 is 500 K, then heat exchanged during process 2→3, is
View SolutionThe cyclic process for 1 mole of an ideal gas is shown in the V-T diagram. The work done in AB, BC and CA respectively is

View SolutionTwo moles of an ideal monoatomic gas is taken through a cycle ABCA as shown in the p - T diagram. During the process AB, the pressure and temperature of the gas vary such that pT = constant. If T1=300K and the amount of heat released by the gas during the process AB is Q=−xR, then the value of x is
View Solution