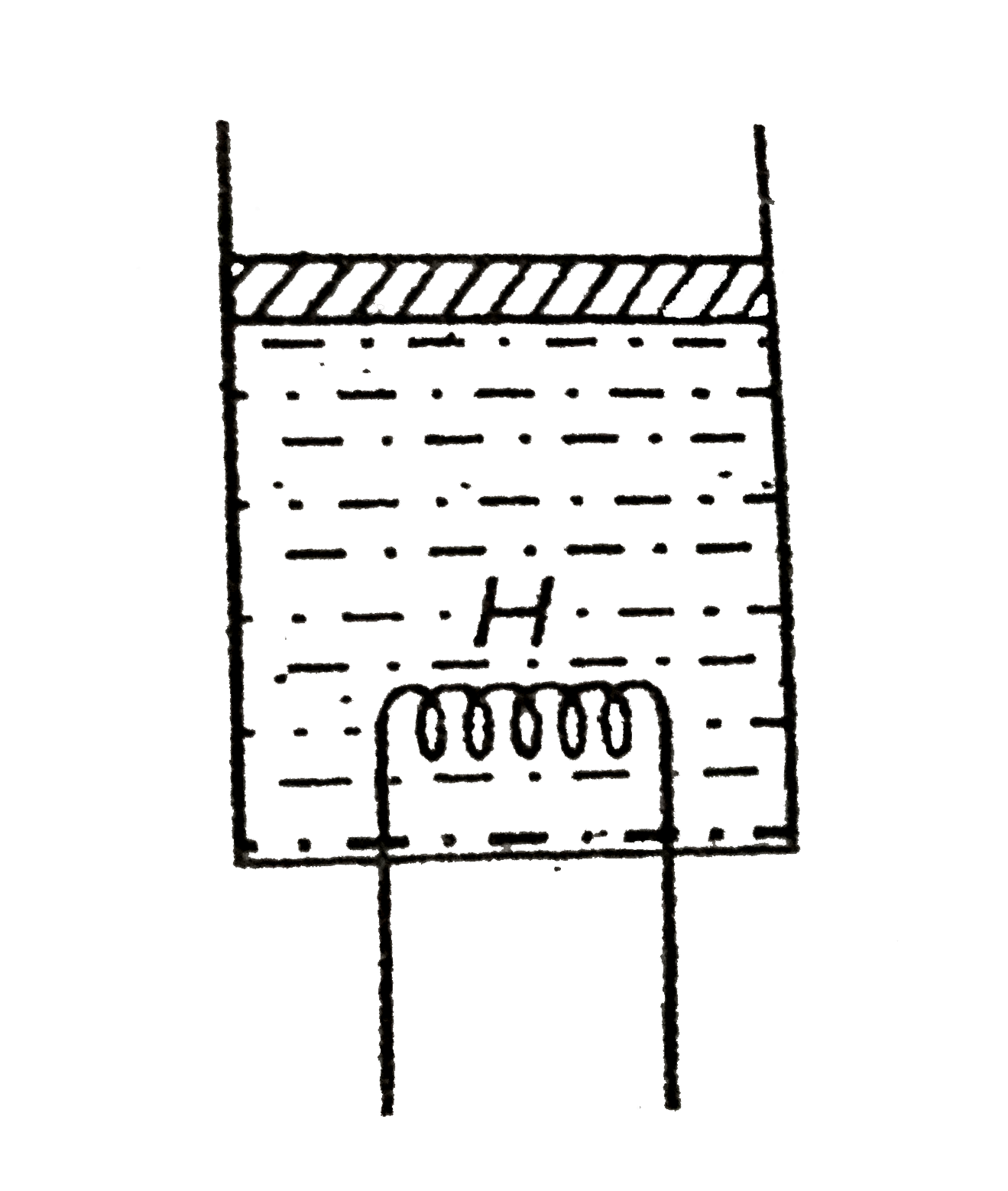
An ideal monoatomic gas is confined in a cylinder by a spring-loaded piston of cross-section 8.0×10−3m2. Initially the gas is at 300K and occupies a volume of 2.4×10−3m3 and the spring is in its relaxed (unstretched, unompressed) state, fig. The gas is heated by a small electric heater until the piston moves out slowly by 0.1m. Calculate the final temperature of the gas and the heat supplied (in joules) by the heater. The force constant of the spring is 8000N/m, atmospheric pressure is 1.0×105Nm−2. The cylinder and the piston are thermally insulated. The piston is massless and there is no friction between the piston and the cylinder. Neglect heat loss through lead wires of the heater. The heat capacity of the heater coil is negligible. Assume the spring to be massless.
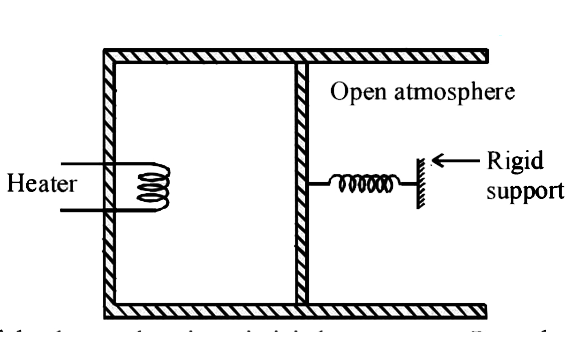
Initially, the pressure of the gas in the cylinder is atmosphere pressure as spring is in relaxed state. Therefore,
P1 atmospheric pressure =1.0×105N/m2
V1= initial volume =2.4×103m3
T1 - initial temperature = 300 K
When the heat is supplied by the heater, the piston is compressed by 0.1 m. The reaction force of compression of spring is equal to kx which acts on the piston or on the gas as
F=kx=8000×0.1×800N
Pressure exerted on the piston by the spring is
ΔP=FA=8008×10−3=1×105N/m2
The total pressure P2 of the gas inside cylinder is
P2=Patm+ΔP=1×105+1×105=2×106N/m2
Since the piston has moved outwards, there has been an increase of ΔV in the volume of the gas, i.e.,
ΔV=A×x=(8×10−3)×(0.1)
=8×10−4m3
The final volume of the gas
V2=V1+ΔV=2.4×10−3+8×10−4=3.2×10−3m3
Let T2 be the final temperature of gas. Then
(P1V1/T1)=(P2V2/T2)⇒T2(P2V2/P1V1)T1
=300×(2×105×3.2×10−3)/(105×2.4×10−3)
=800K
Let the heat supplied by the heater be Q. This is used in two parts, i.e., a part is used in doing external work W due to expansion of the gas and the other part is used in increasing h internal energy of the gas. Hence,
Q=W+ΔU
Now W=∫V2V1PdV=∫x0(Patm+kxA)Adx
[as pressure is (Patm+kx/A and dV=Adx)]
or W=PatmAx+(kx22)
=[105×(8×10−3)(0.1)+8000×(0.1)22]=120J
Further, Δ=nCvΔT
Number of moles of gas can be obtained from initial conditions and gas law is
n=(PVRT)=(1×105×2.4×108.314×300)=0.096mol
Thus, change in internal energy of gas is given as
ΔU=n(32R)ΔT (as for monatomic gas CV=34R)
or U=0.906×32×8.314×598.6J
Heat supplied by the heater = (120 + 598.6) = 718.6 J.
Topper's Solved these Questions
- KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSBook:CENGAGE PHYSICS ENGLISHChapter:KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSExercise:Exercise 2.1
Explore 20 Videos
- KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSBook:CENGAGE PHYSICS ENGLISHChapter:KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSExercise:Exercise 2.2
Explore 28 Videos
- KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSBook:CENGAGE PHYSICS ENGLISHChapter:KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSExercise:Interger
Explore 11 Videos
- KINETIC THEORY OF GASESBook:CENGAGE PHYSICS ENGLISHChapter:KINETIC THEORY OF GASESExercise:Compression
Explore 2 Videos
- LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONBook:CENGAGE PHYSICS ENGLISHChapter:LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONExercise:Single correct anwer type
Explore 14 Videos
Similar Questions
An ideal monoatomic gas is confined in a horizontal cylinder by a spring loaded piston (as shown in the figure). Initially the gas is at temperature T1, pressure P1 and volume V1and the spring is in its relaxed state. The gas is then heated very slowly to temperature T2,pressure P2and volume V2. During this process the piston moves out by a distance x. Ignoring the friction between the piston and the cylinder, the correct statement (s) is (are)
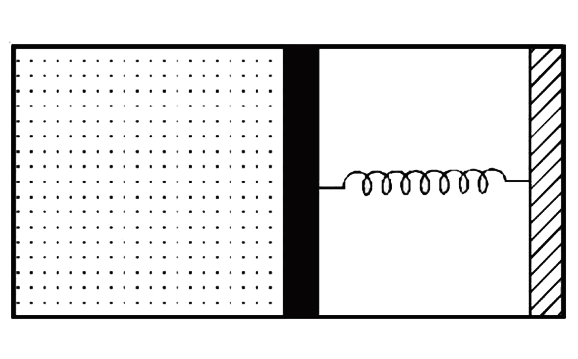
View SolutionTwo moles of an ideal monoatomic gas are confined within a cylinder by a massless and frictionless spring loaded piston of cross-sectional area 4×10−3m2. The spring is, initially in its relaxed state. Now the gas is heated by an electric heater, placed inside the cylinder, for some time. During this time, the gas expands and does 50J of work in moving the piston through a distance 0.10m. The temperature of the gas increases by 50K. Calculate the spring constant and the heat supplied by the heater. Patm=1×105N/m2R=8.314J/mol−K
View Solution
Knowledge Check
One mole of an ideal monoatomic gas at temperature T0 expands slowly according to the law pV = constant. If the final temperature is 2T0, heat supplied to the gas is
A2RT0BRToC32RT0D12RT0One mole of an ideal monatomic gas at temperature T0 expands slowly according to the law PV=constant.If the final temperature is 2T0, heat supplied to the gas is
A2RT0BRT0C32RT0D12RT0One mole of an ideal monoatomic gas at temperature T0 expands slowly according to the law pV = constant. If the final temperature is 2T0, heat supplied to the gas is
Aa.2RT0Bb.RT0Cc.32RT0Dd.12RT0
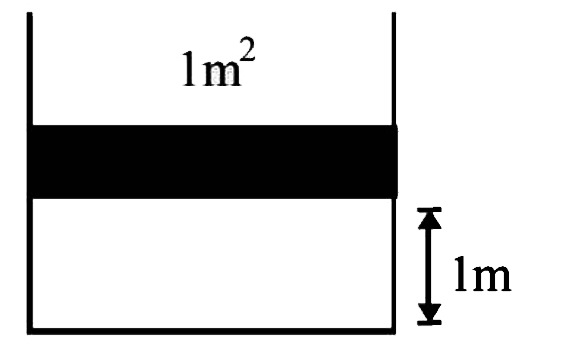
A diatomic gas is enclosed in a vessel fitted with massless movable piston. Area of cross section of vessel is 1m2. Initial height of the piston is 1m (see the figure). The initial temperature of the gas is 300K. The temperature of the gas is increased to 400K, keeping pressure constant, calculate the new height of the piston. The piston is brought to its initial position with no heat exchange. Calculate the final temperature of the gas. You can leave answer in fraction.
View SolutionOne mole of an ideal monoatomic gas at temperature T0 expands slowly according to the law pV = constant. If the final temperature is 2T0, heat supplied to the gas is
View SolutionAn ideal diatomic gas is expanded so that the amount of heat transferred to the gas is equal to the decrease in its internal energy. If in the process, the initial temperature of the gas be T0 and the final volume by 32 times the initial volume, the work done ( in Joules ) by the gas during the process will be
View SolutionIn given figure, gas is slowely heated for sometime. During the process, the increases in internal energy of the gas is 10J and the piston is found to move out by 25cm, then find the amount of heat supplied. The area of cross-section of cylinder =40cm2 and atmospheric pressure 100kPa
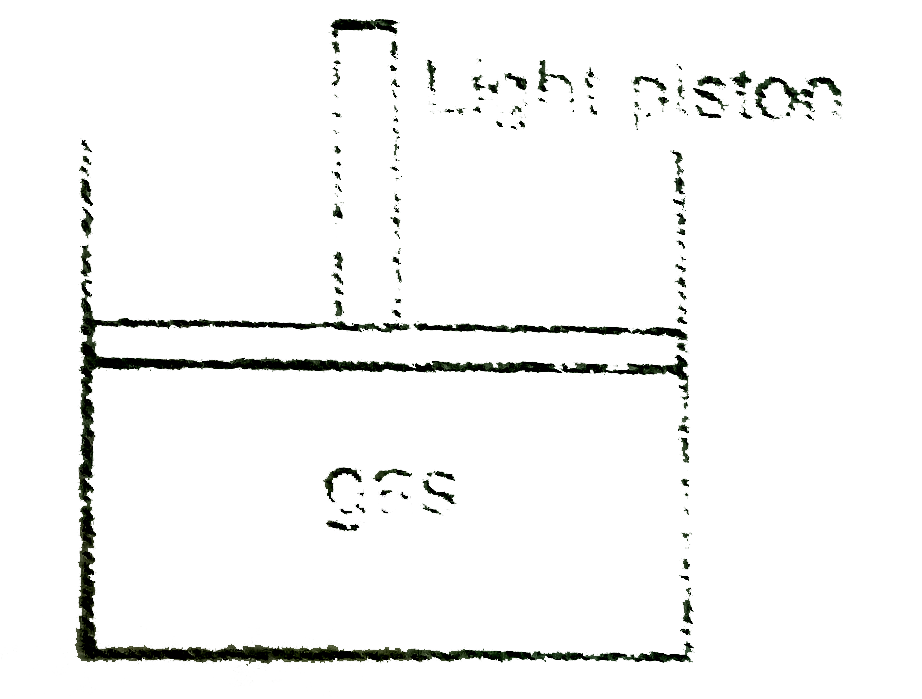
View Solution2 moles of a diatomic gas are enclosed in a cylinder piston arrangment. The area of cross section and mass of the piston are 1cm2 and 1 kg respectively. A heater is supplying heat to the gas very slowly. Find heat supplied (in joule) by the heater is the piston moves through a distance of 10 cm.
 .
.
View Solution