One end of a uniform brass rod 20 cm long and 10cm2 cross-sectional area is kept at 100∘C. The other end is in perfect thermal contact with another rod of identical cross-section and length 10 cm. The free end of this rod is kept in melting ice and when the steady state has been reached, it is found that 360 g of ice melts per hour. Calculate the thermal conductivity of the rod, given that the thermal conductivity of brass is 0.25cal/scm∘CandL=80cal/g.
Let the thermal conductivity of iron be K=cal/s−cm∘C. The thermal resistance of brass rod
R1=l1K1A=15cm0.25cals−cm∘C×20cm2=3s−∘C/cal
And that of iron rod
R2=l2K2A=8cmKcals−cm∘C×20cm2=25Ks−∘C/cal
Since, the two rods are arranged in series, their effective thermal resistance is given by
R=R1+R2=(3+25K)s−∘C/cal
Now, rate of heat flow through the rods=ΔQΔt
But =(684g)3600s(80+calg)=15.2cal/s
Since ΔQΔt=(T1−T2)R∴R=(T1−T2)ΔQ/(Δt)
(3+25K)s∘C/cal=(100−0)∘C15.2cal/s
K=111.8×10−3cal/s−cm−∘C
Topper's Solved these Questions
- CALORIMETRYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CALORIMETRYEXERCISE - Exercise 1.1
23 Videos
- CALORIMETRYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CALORIMETRYEXERCISE - Exercise 1.2
22 Videos
- BASIC MATHEMATICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - BASIC MATHEMATICSEXERCISE - Exercise 2.6
20 Videos
- CENTRE OF MASSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - INTEGER_TYPE
1 Video
Similar Questions
Two rods A and B of same area of cross-section are joined together end to end The free end of the rod A is kept in melting ice at 300 K and free end of B rod at 400 K. The rods are of the same length. The conductivity of A is 3 times that of B. Calculate the temperature of the junction of the rods.
View SolutionOne end of a metal rod is kept in a furnace. In steady state, the temperature of the rod
View Solution
Knowledge Check
One end of a 0.25 m long metal bar is in steam and the other is in contact with ice . If 12 g of ice melts per minute, what is the thermal conductivity of the metal? Given cross-section of the bar =5×10−4m2 and latent heat of ice is 80calg−1
A20cals−1m−1.∘C−1B10cals−1m−1.∘C−1C40cals−1m−1.∘C−1D80cals−1m−1.∘C−1
One end of a metal rod is kept in a furnace. In steady state, the temperature of the rod
View SolutionOne end of a metal rod is dipped in boiling water and the other is dipped in melting ice.
View SolutionA copper rod of length 60 cm long and 8 mm in radius is taken and its one end is kept in boiling water and the other end in ice at 0∘C. If 72 gm of ice melts in one hour with is the thermal conductivity of copper ? Latent of fusion of ice =336KJ/Kg.
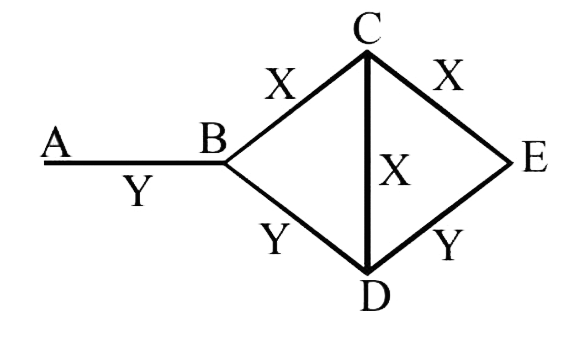
View SolutionThree rods of material X and three rods of material Y are connected as shown in the figure. All the rods are of identical length and cross-sectional area. If the end A is maintained at 60∘C and the junction E at 10∘C. Calculate the temperature of the junction B, C and D. The thermal conductivity of X is 0.92cal/sec−cm∘C and that of Y is 0.46cal/sec−cm−∘C.
View SolutionThe ends of a copper rod of length 1m and area of cross-section 1cm2 are maintained at 0∘C and 100∘C. At the centre of the rod there is a source of heat of power 25 W. Calculate the temperature gradient in the two halves of the rod in steady state. Thermal conductivity of copper is 400Wm−1K−1.
View SolutionThe ends of a copper rod of length 1m and area of cross-section 1cm2 are maintained at 0∘C and 100∘C. At the centre of the rod there is a source of heat of power 25 W. Calculate the temperature gradient in the two halves of the rod in steady state. Thermal conductivity of copper is 400Wm−1K−1.
View SolutionOne end of a brass rod of length 2.0 m and cross section 1cm2 is kept in steam at 100∘C and the other end in ice at 0∘C. The lateral surface of the rod is covered by heat insulator. Determine the amount of ice melting per minute. Thermal conductivity of brass is 110W/m−K and specific latent heat of fusion of ice is 80cal/g.
View Solution
