Question

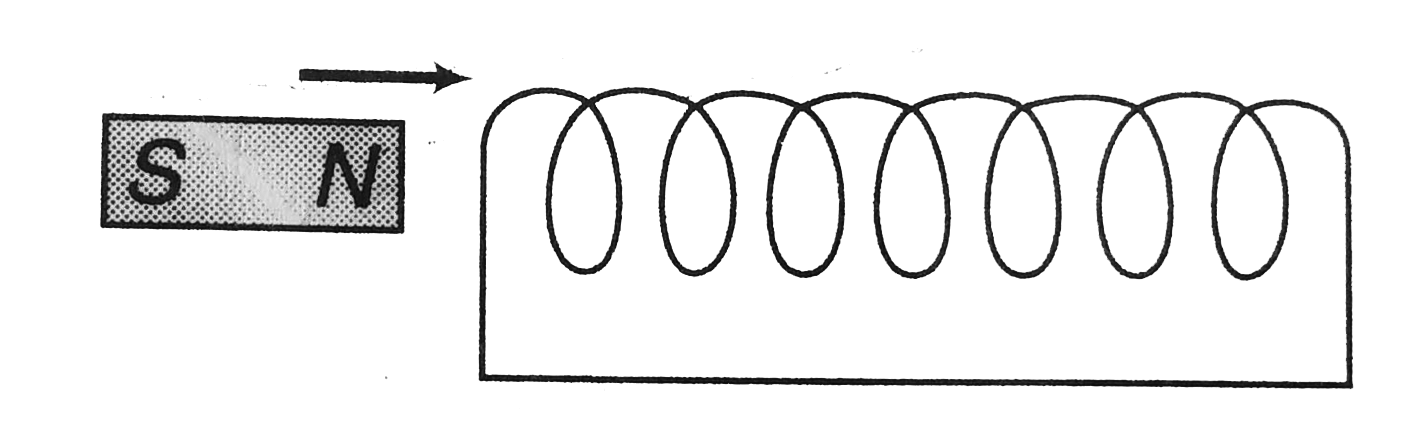
Statement I: An emf is induced ina long solenoid by a bar magnet that moves while totally inside the solenoid along axis of the solenoid.
Statement II: as the magnet moves inside the solenoid the flux through turns of the solenoid changes.

Options

A. (a) Statement I is True, Staatement II is True, Statement II is correct expaination for Statement I.
B. (b) Statement I is True, Staatement II is True, Statement II is NOT a correct expaination for Statement I.
C. ( c) statement I is True, Satement II is false.
D. ( d) statement I is False, Satement II is True.

Answer

D

Explanation

To analyze the statements provided in the question, we will break down the concepts of electromagnetic induction, magnetic flux, and how they relate to the movement of a bar magnet inside a solenoid.

1. Understanding Statement I:
- Statement I claims that an emf (electromotive force) is induced in a long solenoid by a bar magnet that moves while totally inside the solenoid along its axis.
- When a bar magnet is moved inside a solenoid, we need to consider the magnetic flux (Φ) through the solenoid.

2. Magnetic Flux Calculation:
- Magnetic flux (Φ) through the solenoid is given by the formula:
\( \Phi = B \cdot A \)
where \(B\) is the magnetic field inside the solenoid and \(A\) is the cross-sectional area of the solenoid.
- If the bar magnet is moved inside the solenoid, the magnetic field \(B\) due to the magnet changes, but the area \(A\) remains constant.

3. Induced EMF:
- According to Faraday's law of electromagnetic induction, the induced emf (ε) in a circuit is proportional to the rate of change of magnetic flux through the circuit:
\( \epsilon = -\frac{d\Phi}{dt} \)
- If the bar magnet is moved slowly and remains completely inside the solenoid, the magnetic flux through the solenoid does not change because the area is constant and the magnetic field does not change significantly.

4. Conclusion for Statement I:
- Since the magnetic flux remains constant while the bar magnet is moved inside the solenoid, there is no change in flux (dΦ/dt = 0), which means that the induced emf is zero.
- Therefore, Statement I is false.

5. Understanding Statement II:
- Statement II claims that as the magnet moves inside the solenoid, the flux through the turns of the solenoid changes.
- When the magnet is moved, the magnetic field inside the solenoid changes, which can lead to a change in the magnetic flux through the solenoid.

6. Change in Magnetic Flux:
- If the bar magnet is moved in such a way that it enters or exits the solenoid, the magnetic field experienced by the solenoid changes, thus changing the magnetic flux.
- This change in flux will induce an emf according to Faraday's law.

7. Conclusion for Statement II:
- Therefore, Statement II is true because moving the magnet changes the magnetic flux through the solenoid.

Final Conclusion:
- Statement I is false, and Statement II is true. The correct option is B.