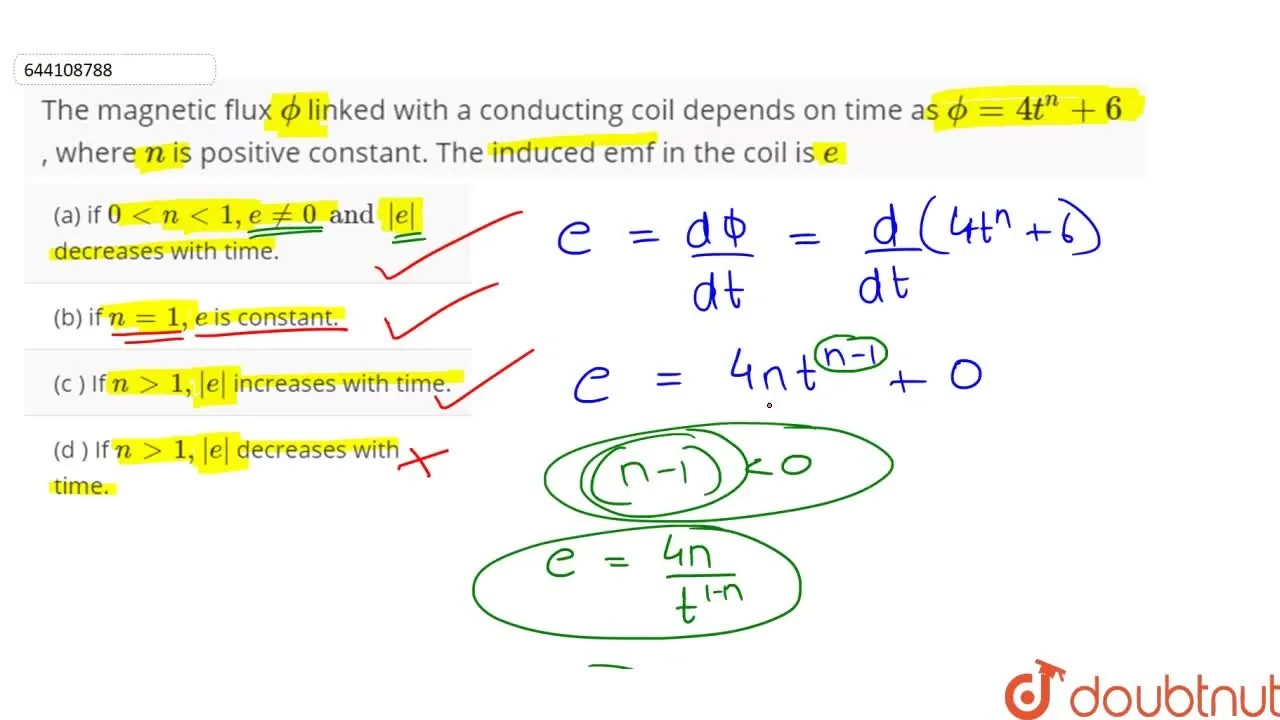
The magnetic flux ϕ linked with a conducting coil depends on time as ϕ=4tn+6, where n is positive constant. The induced emf in the coil is e
(a) if 0<n<1,e≠0and|e| decreases with time.
(b) if n=1,e is constant.
(c ) If n>1,|e| increases with time.
(d ) If n>1,|e| decreases with time.
The correct Answer is:A, B, C
(a , b, c):
ϕ=4tn+6
dϕdt=4ntn−1
|e|=4ntn−1,|e|=4ntn−1
Related Playlists
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Exercises Asserton - Reasoning8videos
ELECTROMAGNETIC INDUCTION
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Exercises Linked Comprehension36videos
ELECTROMAGNETIC INDUCTION
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Exercises Single Correct79videos
ELECTROMAGNETIC INDUCTION
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRICAL MEASURING INSTRUMENTSEXERCISE - M.C.Q2videos
ELECTRICAL MEASURING INSTRUMENTS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSEXERCISE - dpp 3.315videos
ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYS
Similar Questions
The magnetic flux (ϕ) linked with the coil depends on time t as ϕ=atn, where a and n are constants. The emf induced in the coil is e
View SolutionThe magnetic flux linked with a coil is ϕ and the emf induced in it is e.
View SolutionThe magnetic flux linked with a coil (in Wb) is given by the equation ϕ=5t2+3t+16 . The magnetic of induced emf in the coil at fourth second will be
View SolutionThe induced e.m.f. in a coil does not depend on
View SolutionThe magnetic flux linked with a coil is given by an equation ϕ (in webers ) =8t2+3t+5 . The induced e.m.f. in the coil at the fourth second will be
View SolutionThe magnetic flux linked with a coil, in webers is given by the equation ϕ=3t2+4t+9. Then, the magnitude of induced emf at t = 2 s
View SolutionThe primary and secondary coils of a transformer have 50 and 1500 turns respectively. If the magnetic flux ϕ linked with the primary coil is given by ϕ=ϕ0+4t, where ϕ is in webers, t is time in second and ϕ0 is a constant, the output voltage across the secondary coil is
View SolutionThe primary and secondary coils of a transmformer have 50 and 1500 turns respectively. If the magnetic flux ϕ linked with the primary coil is given by ϕ=ϕ0+4t, where ϕ is in weber, t is time in second and ϕ0 is a constant, the output voltage across the secondary coil is
View SolutionThe magnetic flux through each turn of a 100 turn coil is (t3–2t)×10−3Wb, where t is in second. The induced emf at t = 2 s is
View SolutionMagnetic flux passing through a coil varies with time as, ϕ=(2t2−4) weber, Resistance of the coil is 10Ω.
View Solution