A copper rod of length 0.19m is moving with uniform velocity 10ms−1 parallel to a long straight wire carrying a current of 5.0A. The rod is perpendicular to the wire with its ends at distances 0.01 and 0.2m from it. Calculate the emf induced in the rod.
To solve the problem, we need to calculate the electromotive force (emf) induced in a copper rod that is moving parallel to a long straight wire carrying a current. The steps to find the induced emf are as follows:
Step 1: Understand the setup
We have a copper rod of length L=0.19m moving with a velocity v=10m/s parallel to a long straight wire carrying a current I=5.0A. The rod is positioned such that its ends are at distances a=0.01m and b=0.2m from the wire.
Step 2: Calculate the magnetic field (B) due to the wire
The magnetic field B at a distance y from a long straight wire carrying current I is given by the formula:
B=μ0I2πy
where μ0 is the permeability of free space, approximately 4π×10−7T m/A.
Step 3: Set up the expression for induced emf (E)
The induced emf E in the rod can be calculated using the formula:
E=∫baBvdy
Substituting the expression for B:
E=∫ba(μ0I2πy)vdy
Step 4: Substitute the known values
Now, substituting the known values into the equation:
E=μ0Iv2π∫ba1ydy
where a=0.01m and b=0.2m.
Step 5: Calculate the integral
The integral ∫1ydy gives:
∫ba1ydy=ln(ba)
Thus,
E=μ0Iv2πln(ba)
Step 6: Substitute the constants
Now substituting μ0=4π×10−7T m/A, I=5.0A, v=10m/s, a=0.01m, and b=0.2m:
E=(4π×10−7)⋅5⋅102πln(0.20.01)
Step 7: Simplify the expression
The π cancels out:
E=(2×10−7)⋅10⋅ln(20)
E=2×10−6ln(20)
Step 8: Calculate ln(20)
Using a calculator, we find:
ln(20)≈2.9957
Thus,
E=2×10−6⋅2.9957≈5.9914×10−6V≈5.99μV
Final Result
The induced emf in the rod is approximately:
E≈30μV
We have a copper rod of length L=0.19m moving with a velocity v=10m/s parallel to a long straight wire carrying a current I=5.0A. The rod is positioned such that its ends are at distances a=0.01m and b=0.2m from the wire.
The magnetic field B at a distance y from a long straight wire carrying current I is given by the formula:
B=μ0I2πy
where μ0 is the permeability of free space, approximately 4π×10−7T m/A.
The induced emf E in the rod can be calculated using the formula:
E=∫baBvdy
Substituting the expression for B:
E=∫ba(μ0I2πy)vdy
Now, substituting the known values into the equation:
E=μ0Iv2π∫ba1ydy
where a=0.01m and b=0.2m.
The integral ∫1ydy gives:
∫ba1ydy=ln(ba)
Thus,
E=μ0Iv2πln(ba)
Now substituting μ0=4π×10−7T m/A, I=5.0A, v=10m/s, a=0.01m, and b=0.2m:
E=(4π×10−7)⋅5⋅102πln(0.20.01)
The π cancels out:
E=(2×10−7)⋅10⋅ln(20)
E=2×10−6ln(20)
Using a calculator, we find:
ln(20)≈2.9957
Thus,
E=2×10−6⋅2.9957≈5.9914×10−6V≈5.99μV
The induced emf in the rod is approximately:
E≈30μV
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBook:CENGAGE PHYSICS ENGLISHChapter:ELECTROMAGNETIC INDUCTIONExercise:Solved Example
Explore 5 Videos
- ELECTROMAGNETIC INDUCTIONBook:CENGAGE PHYSICS ENGLISHChapter:ELECTROMAGNETIC INDUCTIONExercise:Exercise 3.1
Explore 15 Videos
- ELECTRICAL MEASURING INSTRUMENTSBook:CENGAGE PHYSICS ENGLISHChapter:ELECTRICAL MEASURING INSTRUMENTSExercise:M.C.Q
Explore 2 Videos
- ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSBook:CENGAGE PHYSICS ENGLISHChapter:ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSExercise:dpp 3.3
Explore 15 Videos
Similar Questions
A uniform metal rod is moving with a uniform velocity v parallel to a long straight wire carrying a current I. The rod is perpendicular to the wire with its ends at distance r1andr2 (with r2>r1) form it. The E.M.F. induced in the rod at that instant is
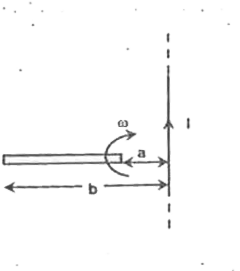
View SolutionA copper rod moves with a constant angular velocity ω about a long straight wire carrying a current I. If the ends of the rod from the wire are at distances a and b, then the e.m.f induced in the rod is
View SolutionThe figure shows a copper rod moving with velocity v parallel to a long straight wire carrying a current I = 100 A. Calculate the induced emf in the rod, assuming that v = 5 m/s, a = 1 cm and b = 100 cm.
View SolutionShows a copper rod moving with velocity v parallel to a long straight wire carrying current =100A. Calculate the induced emf in the rod, where v=5mS−1, a=1cm , b=100cm.
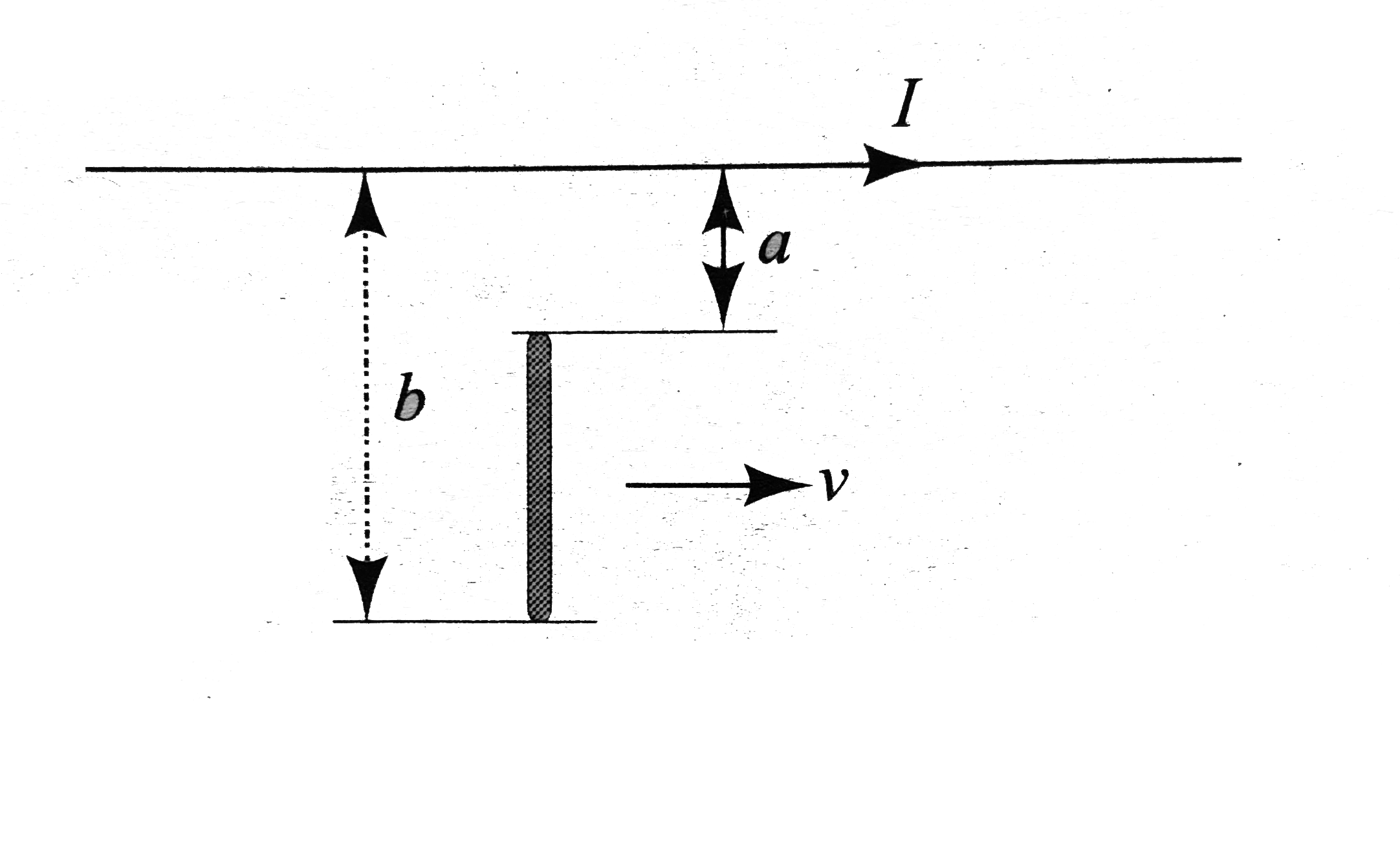
View SolutionA rod of length l is placed perpendicular to a long wire carrying current i. The rod is moved parallel to the wire with a velocity v. Find the emf induced in the rod, if its nearest end is at a distance a from the wire.
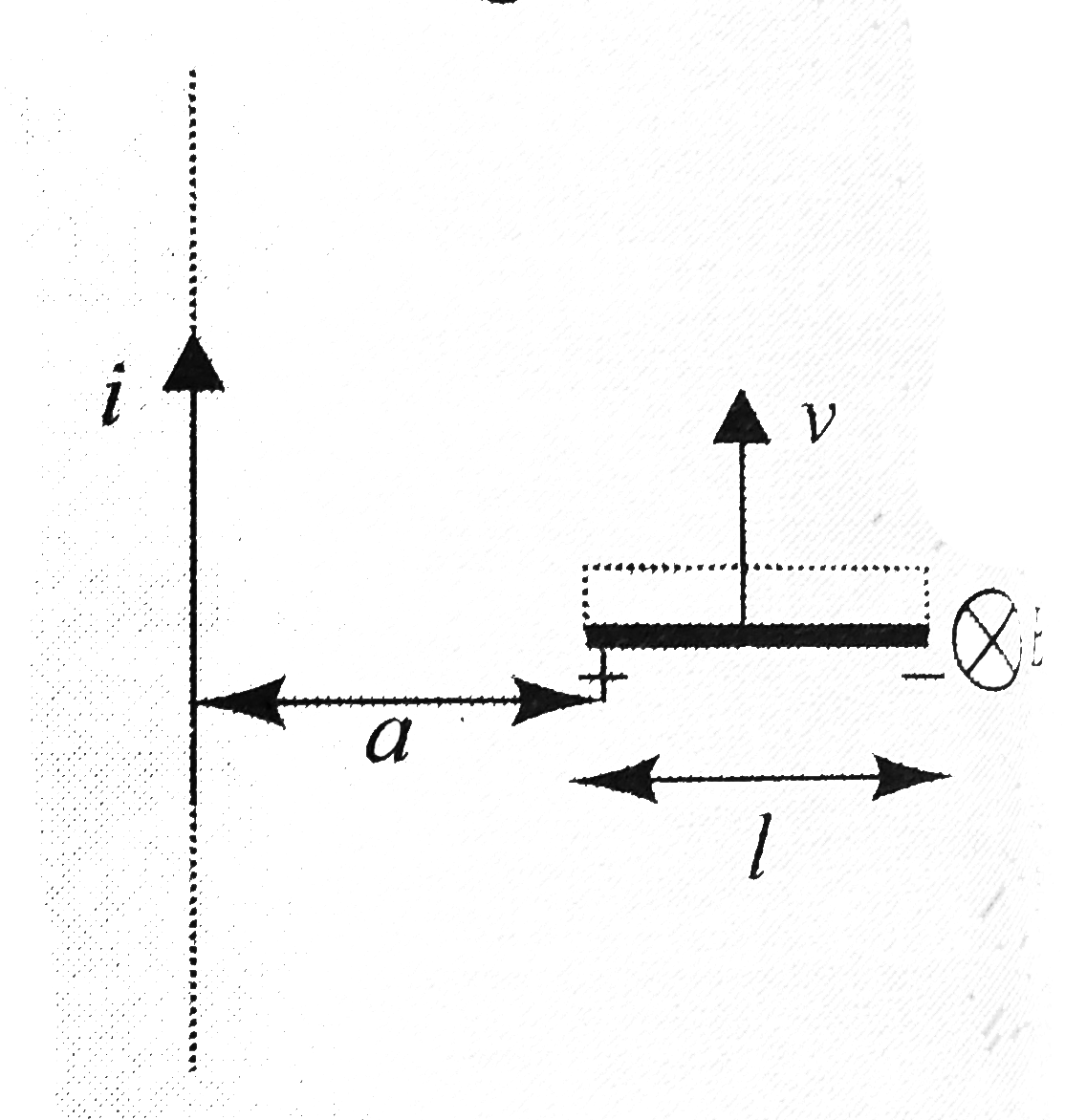
View SolutionA rod of length l is placed perpendicular to a long wire carrying current i. The rod is moved parallel to the wire with a velocity v. Find the emf induced in the rod, if its nearest end is at a distance a from the wire.
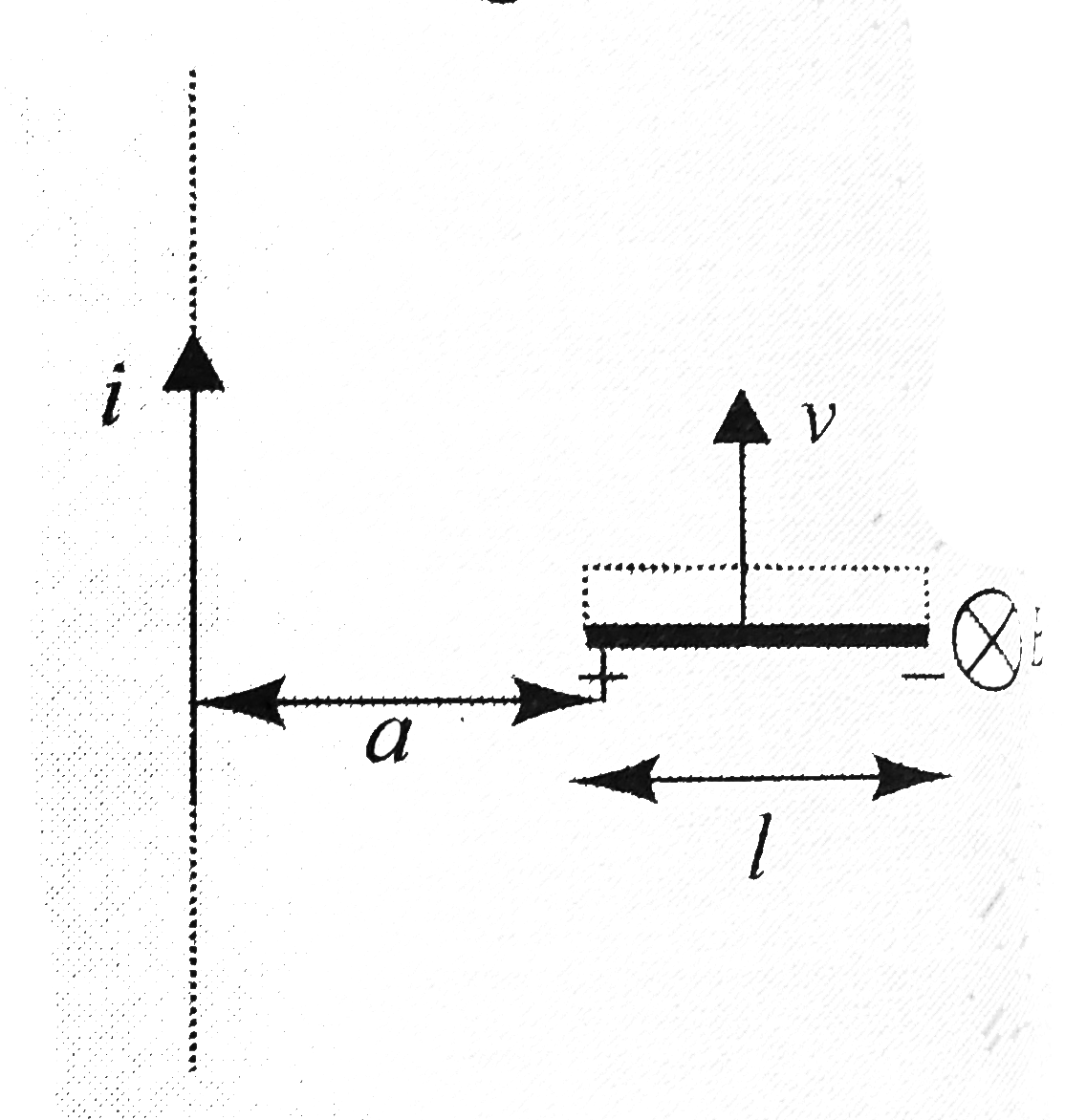
View SolutionA metal rod of length 2 m is rotating with an angualr velocity of 100rads−1 in plane perpendicular to a uniform magnetic field of 0.3 T. The potential difference between the ends of the rod is
View SolutionA rod of length l is kept parallel to a long wire carrying constant current i . It is moving away from the wire with a velocity v. Find the emf induced in the wire when its distance from the long wire is x.
View SolutionTwo long parallel copper wires carry current of 5A each in opposite directions. If the wires are separated by a distance distance of 0.5m, then the force per unit length between the two wires is
View SolutionA conducting circular loop of radius a and resistance per unit length R is moving with a constant velocity v0 , parallel to an infinite conducting wire carrying current i0. A conducting rod of length 2a is approaching the centre of the loop with a constant velocity v02 along the direction 2 of the current. At the instant t=0, the rod comes in contact with the loop at A and starts sliding on the loop with the constant velocity. Neglecting the resistance of the rod and the self-inductance of the circuit, find the following when the rod slides on the loop.
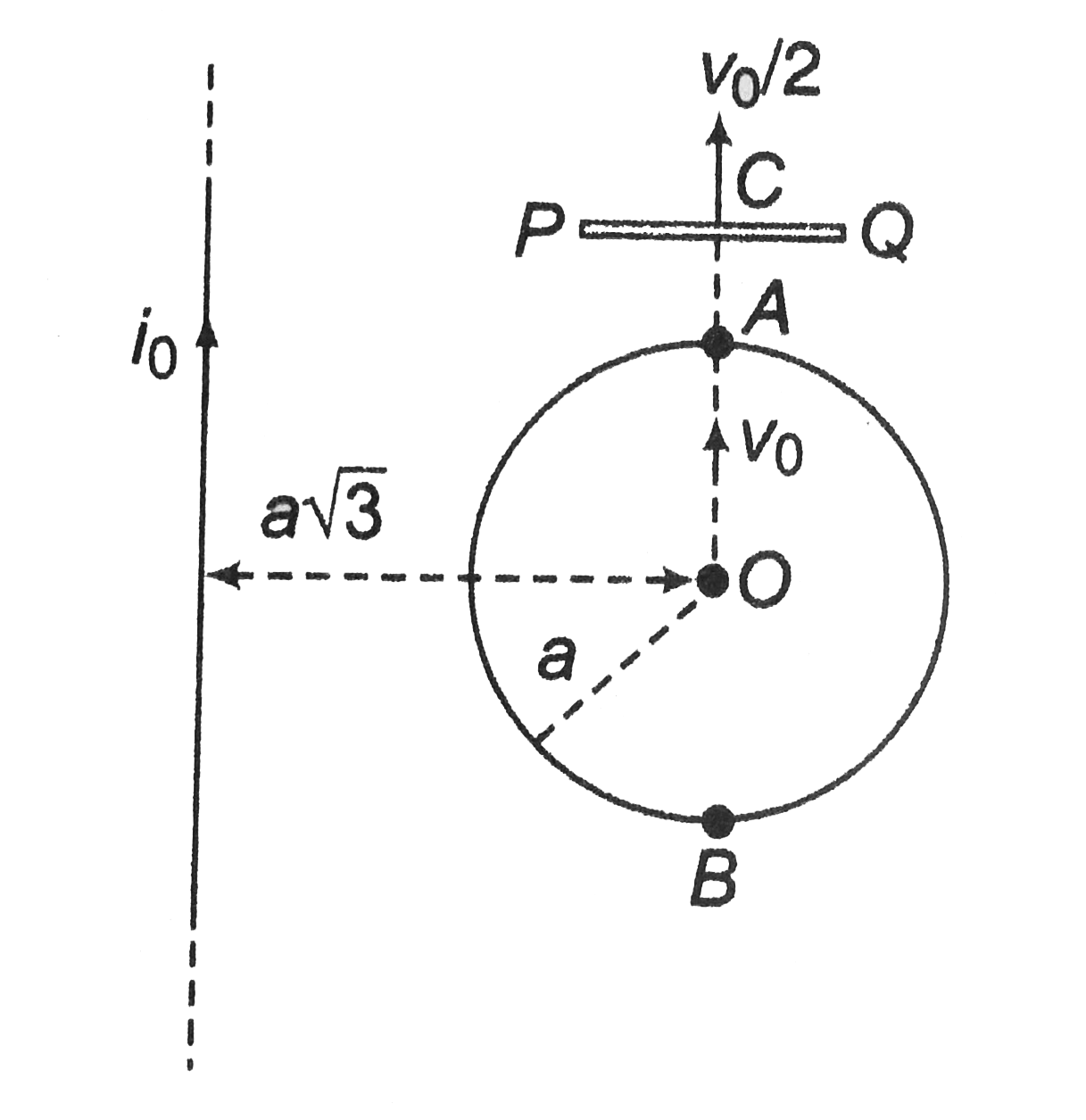
(a) The current through the rod when it is at a distance of (a2) from the point A of the loop.
(b) Force required to maintain the velocity of the rod at that instant.
View Solution
