Two hydrogen atoms are in excited state with electrons inn=2 state.First one is moving to wards left and emits a photon.' of energy E1 towards right. Second one is moving towards right with same speed and emits a photon of energy E2 towards right. Taking recoil of nucleus.into account during_emission process :
E1>E2
E1<E2
E1=E2
information insufficient
The correct Answer is:B
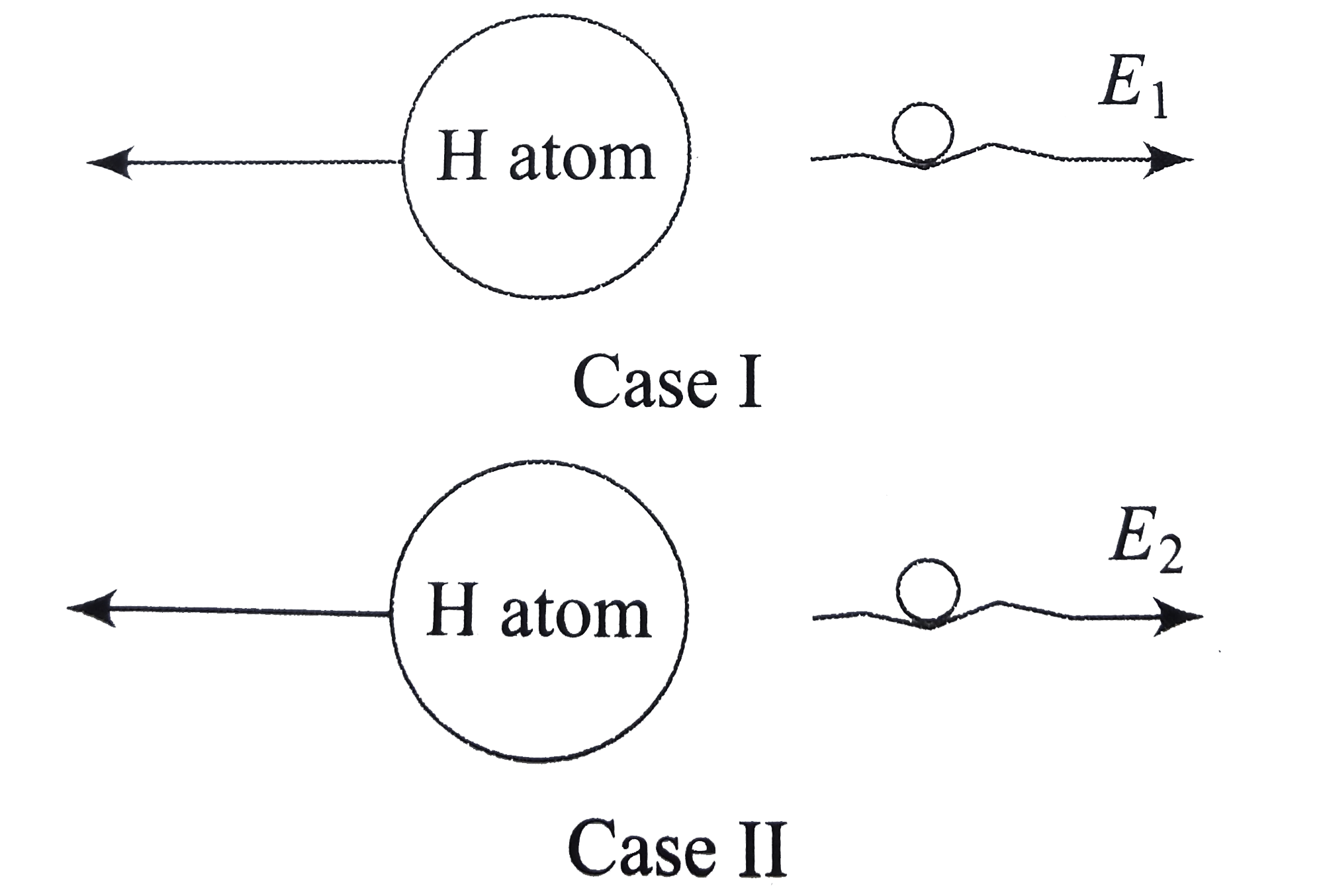
In the first case, HEofH atom increases due to recoil whereas in the second case KE decrease due to recoil.
E2>E1
Related Playlists
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ATOMIC PHYSICSEXERCISE - Multiple Correct13videos
ATOMIC PHYSICS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ATOMIC PHYSICSEXERCISE - Linked Comprehension62videos
ATOMIC PHYSICS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ATOMIC PHYSICSEXERCISE - Subject17videos
ATOMIC PHYSICS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ALTERNATING CURRENTEXERCISE - Single Correct10videos
ALTERNATING CURRENT
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CAPACITOR AND CAPACITANCEEXERCISE - Integer5videos
CAPACITOR AND CAPACITANCE
Similar Questions
Two hydrogen atoms are in excited state with electrons residing in n=2. First one is moving towards left and emits a photon of energy E1 towards right. Second one is moving twoards left with same speed and emits a photon of energy E2 towards left. Taking recoil of nucleus into account during emission process
View SolutionA stationary hydrogen atom in the first excited state emits a photon. If the mass of the hydrogen atom is m and its ionization energy is E, then the recoil velocity acquired by the atom is [speed of light = c]
View SolutionThe recoil speed of a hydrogen atom after it emits a photon is going form n=5 state to n =1 state is ….. m/s.
View SolutionGround state energy of H-atom is (−E1),t he velocity of photoelectrons emitted when photon of energy E2 strikes stationary Li2+ ion in ground state will be:
View SolutionElectron in hydrogen atom first jumps from third excited state to second excited state and then form second excited state to first excited state. The ratio of wavelength λ1:λ2 emitted in two cases is
View SolutionThe electron in a hydrogen atom jumps back from an excited state to ground state, by emitting a photon of wavelength λ0=1615R, where R is Rydbergs's constant. In place of emitting one photon, the electron could come back to ground state by
View SolutionThe electron in a hydrogen atom at rest makes a transition from n=2 energy state to the n=1 ground state.
find the energy (eV) of the emitted photon.View SolutionAn atom consists of theree energy levels given by a ground state with energy E0=0, the first excited state with energy E1=K and the second excited state with energy E2=2K where K>0. The atom is initially in the ground state. Light from a laser which emits photons with energy 1.5K is shined on the atom. Which of the following is/are correct?
View SolutionA hydrogen atom in state n=6 makes two successive transition and reaches the ground state in the first transition a photon of 1.13eV is emitted (a) Find the energy of the photon emitted in the second transition (b) what is the value of n in the intermediate state?
View SolutionSuppose frequency of emitted photon is f0 when the electron of a stationary hydrogen atom jumps from a higher state m to a lower state n. If the atom is moving with a velocity v(<<c) and emits a photon of frequency f during the same transition, then which of the following statement are possible?
View Solution


