Question

A gas of hydrogen like atoms can absorb radiation of 68 eV. Consequently, the atom emits radiations of only three different wavelengths. All the wavelengths are equal or smaller than that of the absorbed photon.
(a) Determine the initial state of the gas atoms.
(b) Identify the gas atoms. (c ) find the minimum wavelength of the emitted radiations.
(d) Find the ionization energy and the respective wavelength for the gas atoms.

Explanation

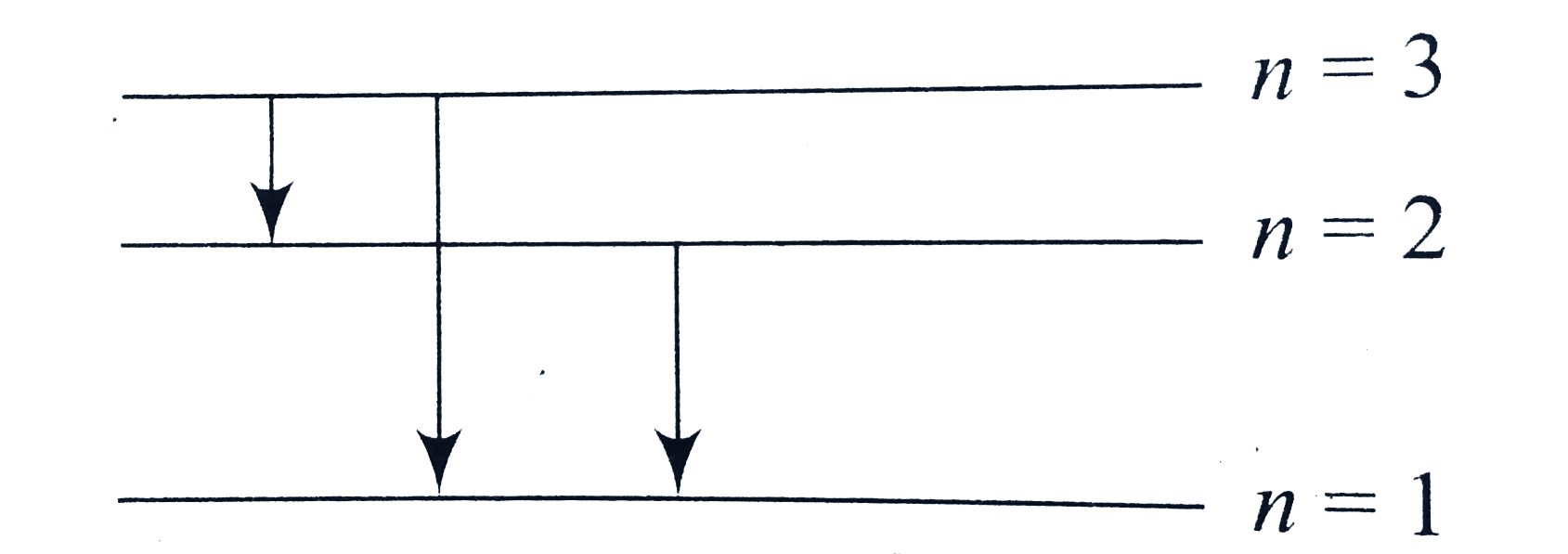
(a) Since three raditions are emitted , therefore, the final excited stateof the gas is `n = 3`.
The initial state of the gas atoms is `N = 2` as all the wevelength are smaller and the energy will be higher.
(b) `13.6Z^(2) [(1)/(2^(2)) - (1)/(3^(2))] = 68`
or `13.6Z^(2) [(5)/(36)] = 68 implies Z = 6`
( c) The minimum wevelength corresponds to the transition
`n = 3 to n = 1`
`(1)/(lambda_(min)) = RZ^(2) [(1)/(1^(2)) - (1)/(3^(2))]`
or `lambda_(min) = (9)/(8RZ^(2)) = (9)/(8 (1.097 xx 10^(7)) (6)^(2)) = 25.32 Å`