A mercury lamp is a convenient source for studying frequency dependence of photoelectric emission, since it gives a number of spectral lines ranging from the UV to the end of the red visible spectrum. In our experiment with rubidium photocell, the following lines from a mercury source were used: λ1=3650A∘,λ2=4047A∘,λ3=4358A∘,λ4=5461A∘,λ5=6907A∘ The stopping voltages, respectively were measured to be:
V01=1.28V,V02=0.95V,V03=0.74V,V04=0.16V,V05=0V.
(a) Determine the value of Planck's constant h.
(b) Estimate the threshold frequency and work function for the material.
To solve the problem, we will follow these steps:
Step 1: Convert Wavelengths to Frequencies
We start by converting the given wavelengths (in Angstroms) to frequencies using the formula:
f=cλ
where c=3×108m/s and λ is in meters.
Given:
- λ1=3650Å=3650×10−10m
- λ2=4047Å=4047×10−10m
- λ3=4358Å=4358×10−10m
- λ4=5461Å=5461×10−10m
- λ5=6907Å=6907×10−10m
Calculating frequencies:
f1=3×1083650×10−10≈8.219×1014Hz
f2=3×1084047×10−10≈7.412×1014Hz
f3=3×1084358×10−10≈6.884×1014Hz
f4=3×1085461×10−10≈5.493×1014Hz
f5=3×1086907×10−10≈4.34×1014Hz
Step 2: Use the Photoelectric Equation
The photoelectric effect is described by the equation:
eV0=hf−ϕ
where V0 is the stopping potential, h is Planck's constant, f is frequency, and ϕ is the work function.
Rearranging gives:
V0=hfe−ϕe
This can be expressed as:
V0=hef−ϕe
This is a linear equation in the form y=mx+c, where:
- y=V0
- x=f
- m=he
- c=−ϕe
Step 3: Plotting and Finding the Slope
Using the stopping voltages V0 and the corresponding frequencies f, we can plot V0 against f and find the slope.
Given stopping potentials:
- V01=1.28V
- V02=0.95V
- V03=0.74V
- V04=0.16V
- V05=0V
Step 4: Finding the Threshold Frequency
The threshold frequency f0 corresponds to the stopping potential V0=0. By extrapolating the linear graph, we can find f0.
Using the two points:
- (f4,V4)=(5.493×1014,0.16)
- (f5,V5)=(4.34×1014,0)
Setting up the equation:
0.16=m(5.493×1014)+c
0=m(4.34×1014)+c
Solving these equations will give us the slope m and the intercept c.
Step 5: Calculate Planck's Constant h
From the slope m=he, we can find h by multiplying the slope by the charge of an electron e≈1.6×10−19C.
Step 6: Calculate Work Function ϕ
Using the threshold frequency f0:
ϕ=hf0
Final Answers
(a) The value of Planck's constant h is calculated from the slope.
(b) The threshold frequency f0 and work function ϕ can be calculated using the values obtained.
We start by converting the given wavelengths (in Angstroms) to frequencies using the formula:
f=cλ
where c=3×108m/s and λ is in meters.
- λ1=3650Å=3650×10−10m
- λ2=4047Å=4047×10−10m
- λ3=4358Å=4358×10−10m
- λ4=5461Å=5461×10−10m
- λ5=6907Å=6907×10−10m
f1=3×1083650×10−10≈8.219×1014Hz
f2=3×1084047×10−10≈7.412×1014Hz
f3=3×1084358×10−10≈6.884×1014Hz
f4=3×1085461×10−10≈5.493×1014Hz
f5=3×1086907×10−10≈4.34×1014Hz
The photoelectric effect is described by the equation:
eV0=hf−ϕ
where V0 is the stopping potential, h is Planck's constant, f is frequency, and ϕ is the work function.
V0=hfe−ϕe
This can be expressed as:
V0=hef−ϕe
This is a linear equation in the form y=mx+c, where:
- y=V0
- x=f
- m=he
- c=−ϕe
Using the stopping voltages V0 and the corresponding frequencies f, we can plot V0 against f and find the slope.
- V01=1.28V
- V02=0.95V
- V03=0.74V
- V04=0.16V
- V05=0V
The threshold frequency f0 corresponds to the stopping potential V0=0. By extrapolating the linear graph, we can find f0.
- (f4,V4)=(5.493×1014,0.16)
- (f5,V5)=(4.34×1014,0)
0.16=m(5.493×1014)+c
0=m(4.34×1014)+c
From the slope m=he, we can find h by multiplying the slope by the charge of an electron e≈1.6×10−19C.
Using the threshold frequency f0:
ϕ=hf0
(a) The value of Planck's constant h is calculated from the slope.
(b) The threshold frequency f0 and work function ϕ can be calculated using the values obtained.
Topper's Solved these Questions
- PHOTOELECTRIC EFFECTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - PHOTOELECTRIC EFFECTEXERCISE - Examples
8 Videos
- PHOTOELECTRIC EFFECTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - PHOTOELECTRIC EFFECTEXERCISE - Exercise 3.1
15 Videos
- NUCLEAR PHYSICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NUCLEAR PHYSICSEXERCISE - ddp.5.5
14 Videos
- RAY OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RAY OPTICSEXERCISE - DPP 1.6
12 Videos
Similar Questions
A photon of frequency v causes photo electric emission from a surface with threshold frequency v0. The de-Broglies wavelength (λ) of the photo electron emitted is given by:
View SolutionIn photoelectric effect the slope of straight line graph between stopping potential (Vs) and frequency of incident light (v) gives
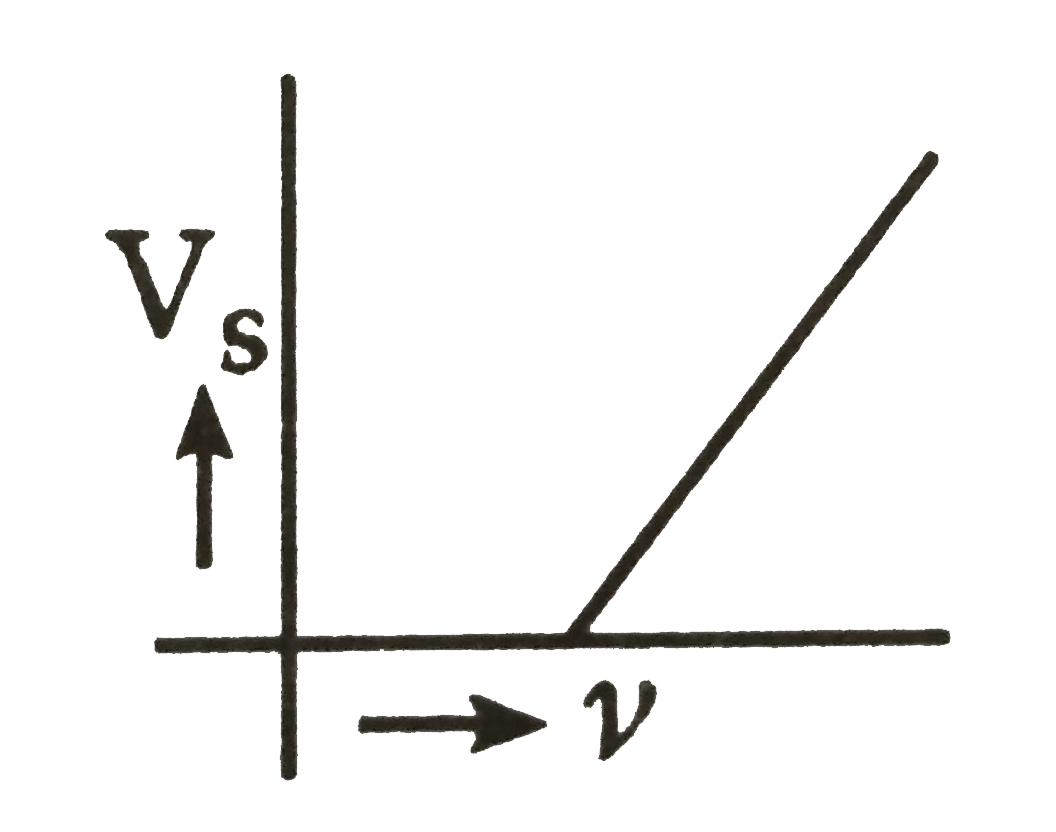
View SolutionIf the threshold frequency of a metal for photoelectric effect is v0 then which of the following will not happen ?
View SolutionA sine wave has an amplitude A and wavelength λ. Let V be the wave velocity and v be the maximum velocity of a particle in the medium. Then
View SolutionWhen a metallic surface is illuminated with radiation of wavelength λ, the stopping potential is V. If the same surface is illuminated with radiation of wavelength 2λ , the stopping potential is V4. The threshold wavelength surface is :
(a) 5λ (b) 52λ (c) 3λ (d) 4λ
View SolutionThe resistance of metal is given by V=IR, The voltage in the resistance is V=(8±0.5)V and current in the resistance is I=(2±0.2)A, the value fo resistance with its percentage error is
View Solutionwhen a monochromatic point source of light is at a distance 0.2 m from a photoelectric cell, the saturation current and cut-off voltage are 12.0 mA and 0.5 V. If the same source is placed 0.4 m away from the photoelectric cell, then the saturation current and the stopping potential respectively are
View SolutionFor a photoelectric cell the graph showing the variation of cut of voltage (V0) with frequency (v) of incident light is best represented by

View SolutionThe wavelengths and frequencies of photons in transition 1,2 and 3 for hydrogen like atom are λ1,λ2,λ3,v1,v2 and v3 respectively. Then:

View SolutionWhen radiation of wavelength λ is used to illuminate a metallic surface , the stopping potential is V. When the same suface is illuminated with radiation of wavelength 3λ the stopping is V4 . If the therehold wavelength for the metallic
View Solution
