The work function of Silver and sodium are 4.6and2.3eV, respectively . The ratio of the slope of the stopping potential versus frequency plot for silver to that of sodium is
To solve the problem, we need to analyze the stopping potential versus frequency plot for both silver and sodium. The stopping potential (Vs) is related to the frequency (ν) of the incident light and the work function (φ) of the metal.
1. Understand the Photoelectric Equation:
The energy of the incoming photon is given by:
E=hν
where h is Planck's constant and ν is the frequency of the incident light.
2. Relate Photon Energy to Work Function and Kinetic Energy:
The energy of the photon is used to overcome the work function (φ) of the metal and to give kinetic energy to the emitted electrons:
hν=ϕ+eVs
where e is the charge of the electron and Vs is the stopping potential.
3. Rearranging the Equation:
Rearranging the equation gives:
Vs=heν−ϕe
This equation resembles the equation of a straight line y=mx+c, where:
- y is Vs
- x is ν
- m (slope) is he
- c (y-intercept) is −ϕe
4. Determine the Slope for Both Metals:
The slope m for both metals (Silver and Sodium) is the same:
Slope=he
Therefore, the slope for Silver (Ag) is he and for Sodium (Na) is also he.
5. Calculate the Ratio of the Slopes:
The ratio of the slope of the stopping potential versus frequency plot for silver to that of sodium is:
Ratio=SlopeAgSlopeNa=hehe=1
Final Answer:
The ratio of the slope of the stopping potential versus frequency plot for silver to that of sodium is 1.
The energy of the incoming photon is given by:
E=hν
where h is Planck's constant and ν is the frequency of the incident light.
The energy of the photon is used to overcome the work function (φ) of the metal and to give kinetic energy to the emitted electrons:
hν=ϕ+eVs
where e is the charge of the electron and Vs is the stopping potential.
Rearranging the equation gives:
Vs=heν−ϕe
This equation resembles the equation of a straight line y=mx+c, where:
- y is Vs
- x is ν
- m (slope) is he
- c (y-intercept) is −ϕe
The slope m for both metals (Silver and Sodium) is the same:
Slope=he
Therefore, the slope for Silver (Ag) is he and for Sodium (Na) is also he.
The ratio of the slope of the stopping potential versus frequency plot for silver to that of sodium is:
Ratio=SlopeAgSlopeNa=hehe=1
The ratio of the slope of the stopping potential versus frequency plot for silver to that of sodium is 1.
Topper's Solved these Questions
- PHOTOELECTRIC EFFECTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - PHOTOELECTRIC EFFECTEXERCISE - Linked Comprehension Type
3 Videos
- NUCLEAR PHYSICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NUCLEAR PHYSICSEXERCISE - ddp.5.5
14 Videos
- RAY OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RAY OPTICSEXERCISE - DPP 1.6
12 Videos
Similar Questions
Knowledge Check
In photoelectric effect experiment, the slope of the graph of the stopping potential versus frequency gives the value of :
AheBhCehDhceThe work function for Al, K and Pt is 4.28 eV, 2.30 eV and 5.65 eV respectively. Their respective threshold frequencies would be
APt>Al>KBAl>Pt>KCK>Al>PtDAl>K>Pt
Sodium and copper have work functions 2.3eVand4.5eV respectively . Then the ratio of the wavelength is nearest
View SolutionThe work function for tungsten and sodium are 4.5eV and 2.3eV respectively . If the threshold wavelength λ for sodium is 5460Å, the value of λ for tungsten is
View SolutionThe work functions of tungsten and sodium are 5.06eV and 2.53eV respectively. If the threshold wavelenth for sodium is 5896Å, then the threshold wavelength for the tungsten will be
View Solution(i) Plot a labelled graph of |Vs| where Vs is stopping potential versus frequency f of the incident radiation.
(ii) State how will you use this graph to determine the value of Planck.s constant.
View SolutionIf the work functions of three photosensitive materials are 1 eV, 2 eV and 3 eV respectively, then the ratio of the respective frequencies of light that produce photoelectrons of the maximum kinetic energy of 1 eV from each of them is
View SolutionGraph between stopping potential and frequency of light as shown find the work function
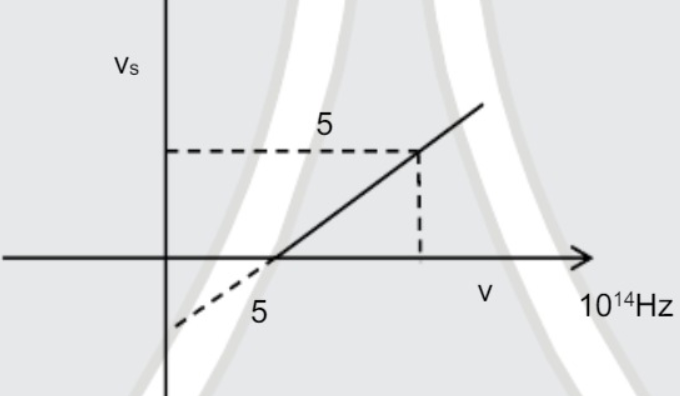
View Solution
