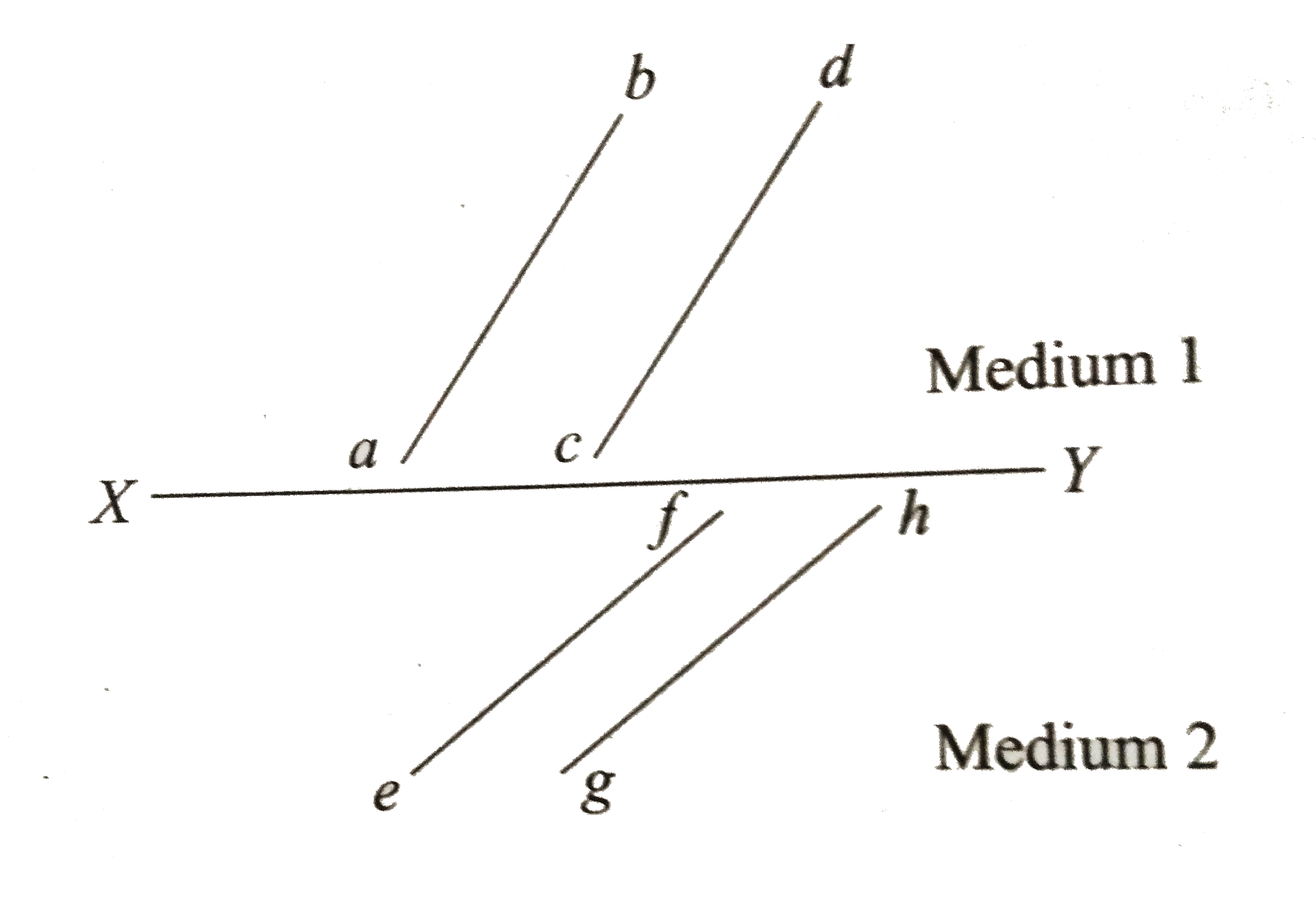
Fig. shows a surface XY separating two transparent media, medium 1 and medium 2. Lines ab and cd represent wavefronts of a light wave travelling in medium 1 and incident on XY. Line ef and gh represent wavefront of the light wave in medium 2 after rafraciton.

The phase of the ligth wave at c, d, e, and f are ϕc, phi_(d), ϕe and ϕf, respectively. It is given that ϕc≠ϕf. Then
ϕc cannot be equal to ϕd
ϕd can be equal to ϕd
ϕd−ϕf is equal to ϕc−ϕe
ϕd−ϕc is not equal to ϕf−ϕe
The correct Answer is:c
All points on a wavefront are at the same phase
ϕd=ϕc and ϕf=ϕe
ϕd−ϕf=ϕc−ϕe
Hence, the correct option is (c).
Topper's Solved these Questions
- WAVE OPTICSBook:CENGAGE PHYSICS ENGLISHChapter:WAVE OPTICSExercise:Matching Column Type
Explore 2 Videos
- WAVE OPTICSBook:CENGAGE PHYSICS ENGLISHChapter:WAVE OPTICSExercise:Comprehension Type
Explore 14 Videos
- WAVE OPTICSBook:CENGAGE PHYSICS ENGLISHChapter:WAVE OPTICSExercise:MCQ
Explore 2 Videos
- SOURCES OF MAGNETIC FIELDBook:CENGAGE PHYSICS ENGLISHChapter:SOURCES OF MAGNETIC FIELDExercise:single correct Ansewer type
Explore 12 Videos
Similar Questions
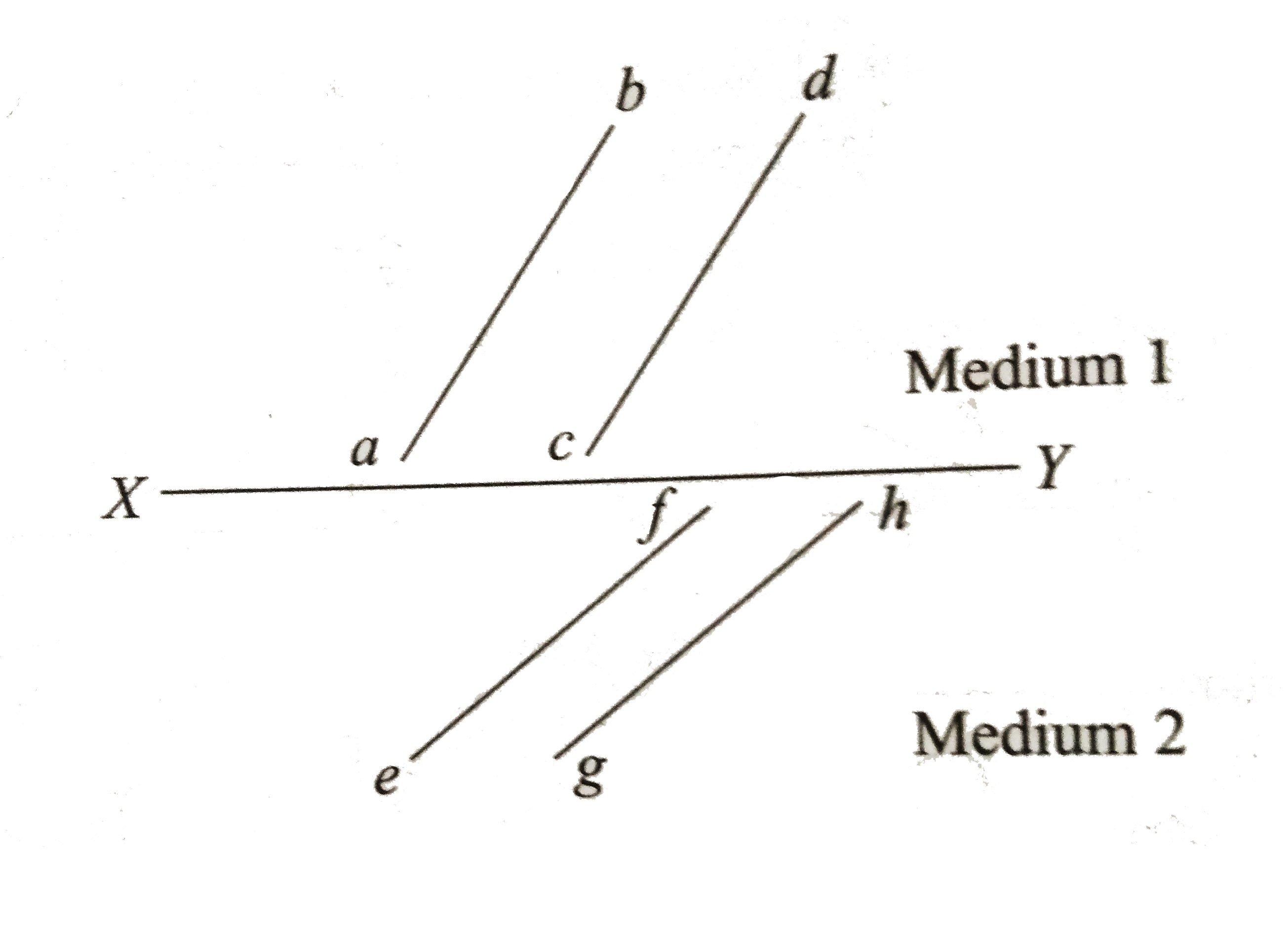
Fig. shows a surface XY separating two transparent media, medium 1 and medium 2. Lines ab and cd represent wavefronts of a light wave travelling in medium 1 and incident on XY. Line ef and gh represent wavefront of the light wave in medium 2 after rafraciton.
Light travel as a
View SolutionFig. shows a surface XY separating two transparent media, medium 1 and medium 2. Lines ab and cd represent wavefronts of a light wave travelling in medium 1 and incident on XY. Line ef and gh represent wavefront of the light wave in medium 2 after rafraciton. Speed of light is
View SolutionLight waves travels in vacuum along the X-axis. Which of the following may represent the wavefronts?
View SolutionDuring the propagation of e.m. wave in a medium
View SolutionThe waves which cannot travel without medium are
View SolutionA wave has velocity u in medium P and velocity 2 u in medium Q . If the wave is incident in medium P at an angle of 30∘ then the angle of refraction will be
View SolutionWavelength of given light waves in air and in a medium are 6000 Å and 4000 Å respectively . The critical angle for the medium is given by
View SolutionIf ϕ(x)=ϕ'(x) and ϕ(1)=2, then ϕ(3) equals
View SolutionThe critical angle between a certain transparent medium and air is ϕ. A ray of light travelling through air enters the medium at an angle of incidence equal to its Brewster angle θ. Therefore, the angle of refraction is
View SolutionThe critical angle between a certain transparent medium and air is ϕ. A ray of light travelling through air enters the medium at an angle of incidence equal to its Brewster angle θ. Therefore, the angle of refraction is
View Solution
