In a Young's double-slit experiment set up, source S of wavelength 6000Å illuminates two slits S1 and S2 which act two coherent sources. The sources S oscillates about its shown position according to the eqation y=1+cosπt, where y is in millimeter and t in second.
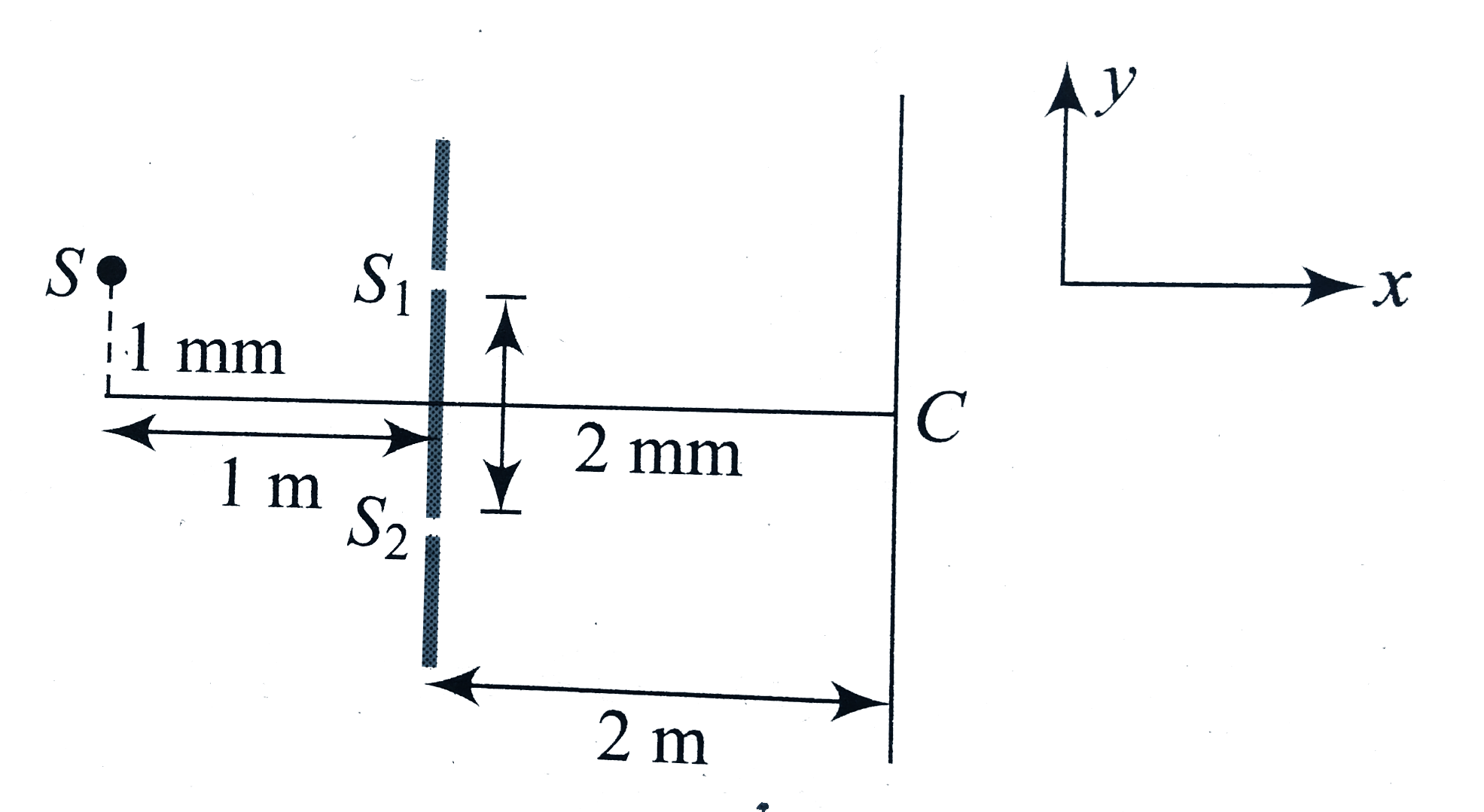
At t=0, fringe width is β1, and at t=2 s, width of figure is β2. Then
β1>β2
β2>β1
β1=β2
data is insufficient
The correct Answer is:b
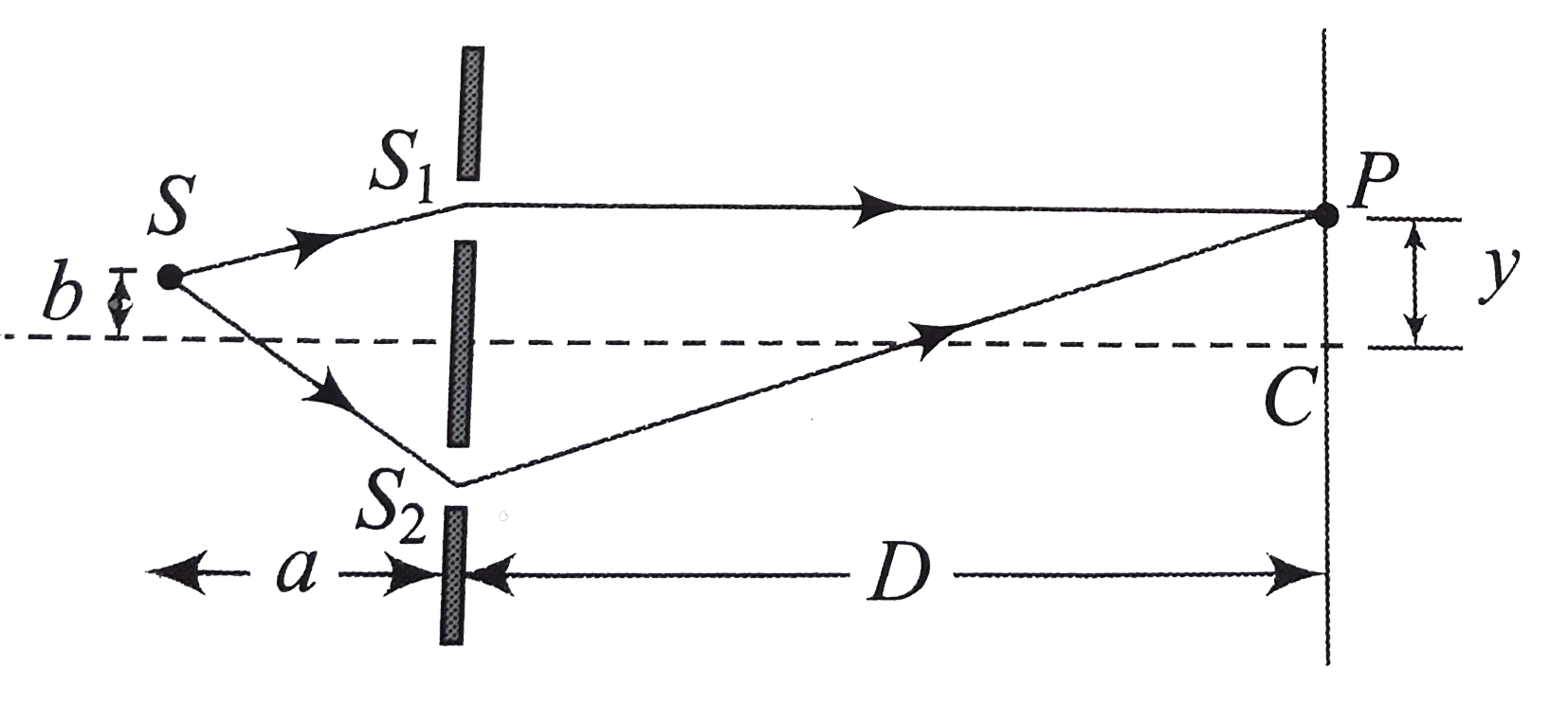
Path difference at any point on the screen Δx=bda+y'dD
For central maixma. Δx=0
⇒y'=−bda=−(1+cosπt)21
y'=−2(1+cosπt)
position of central maxima at any time is
y'=−2(1+conπt) mm
At t=2s,y,'=−4mm
At t=1 s, central maxima is at y'=0.
But when a plate is inserted central maxima is at
y1=Dd(μ−1)t=1mm
Topper's Solved these Questions
- WAVE OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WAVE OPTICSEXERCISE - Integer
3 Videos
- WAVE OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WAVE OPTICSEXERCISE - Archives
4 Videos
- WAVE OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WAVE OPTICSEXERCISE - Assertion- Reasoning
13 Videos
- SOURCES OF MAGNETIC FIELDBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOURCES OF MAGNETIC FIELDEXERCISE - single correct Ansewer type
12 Videos
Similar Questions
In a Young's double-slit experiment set up, source S of wavelength 6000Å illuminates two slits S1 and S2 which act two coherent sources. The sources S oscillates about its shown position according to the eqation y=1+cosπt, where y is in millimeter and t in second.
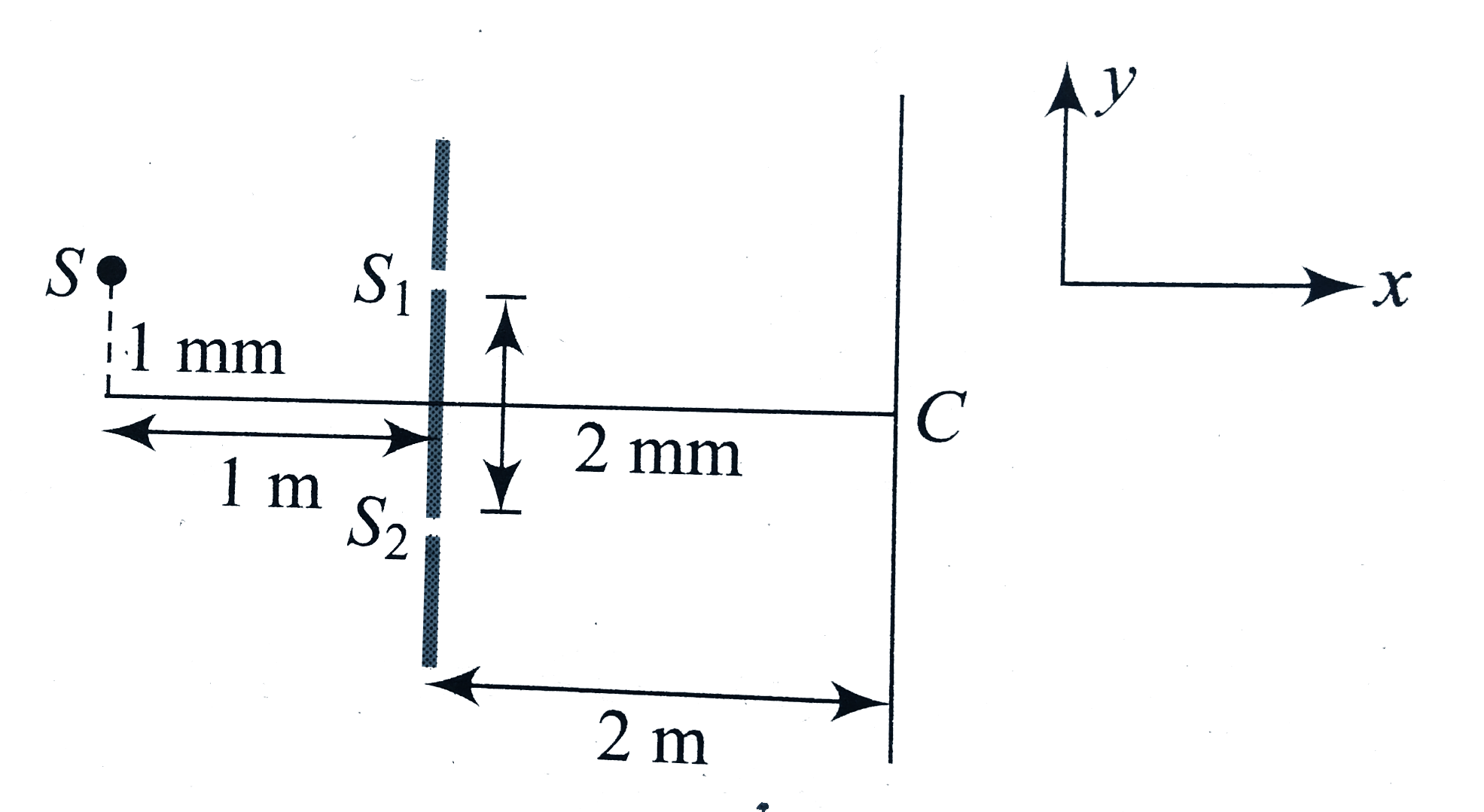
At t=2 s, the position of central maxima is
View SolutionIn young's double-slit experiment set up, sources S of wavelength 50 nm illumiantes two slits S1 and S2 which act as two coherent sources. The sources S oscillates about its own position according to the equation y=0.5sinπt, where y is in nm and t in seconds. The minimum value of time t for which the intensity at point P on the screen exaclty in front of the upper slit becomes minimum is
View Solution
Knowledge Check
In Young's double slit experiment, the fringe width with light of wavelength 6000 Ã… is 3 mm. The fringe width, when the wavelength of light is changed to 4000 Ã… is
A3 mmB1 mmC2 mmD4 mm
In the Young’s double slit experiment, for which colour the fringe width is least
View SolutionIn Young.s double slit experiment the band width is minimum for the colour
View SolutionIn a Young’s double slit experiment, the source illuminating the slits is changed from blue to violet. The width of the fringes
View SolutionIn Young.s double slit experiment both the slits are similar. If width of one of the slits is doubled then
View SolutionIn Young.s double slit experiment sodium light is replaced by blue lamp, then the fringe width
View SolutionIn Young's double slit experiment, the ratio of maximum and minimum intensities in the fringe system is 9:1 the ratio of amplitudes of coherent sources is
View SolutionIn Young.s double slit experiment, blue-green light of wavelength 500nm is used. The slits are 1.20mm apart, and the viewing screen is 5.40m away from the slits. What is the fringe width.
View Solution
