A point sources $S$ emitting light of wavelength $600 n m$ is placed at a very small height $h$ above the flat reflecting surface $A B$ (see figure).The intensity of the reflected light is $36 %$ of the intensity.interference firnges are observed on a screen placed parallel to the reflecting surface a very large distance $D$ from it.
(A)What is the shape of the interference fringes on the screen?

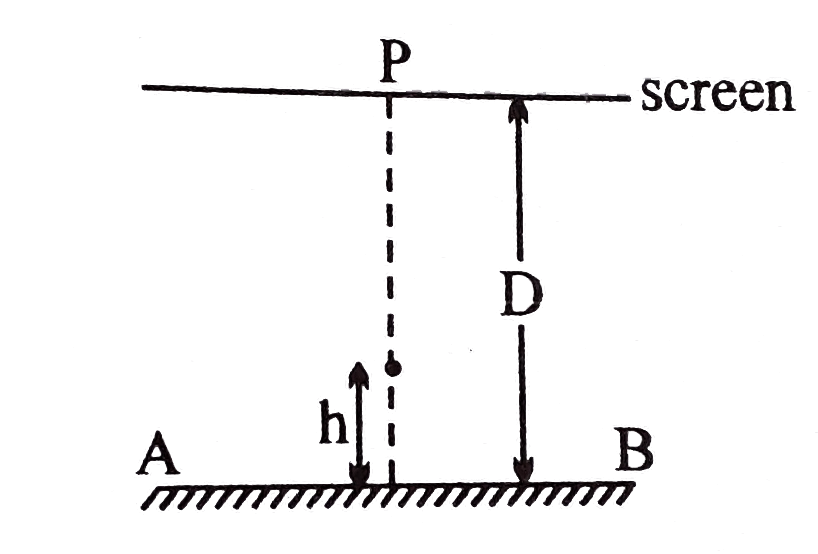
(B)Calculate the ratio of the minimum to the maximum to the maximum intensities in the interference fringes fromed near the point $P$ (shown in the figure) (c) if the intenstities at point $P$ corresponds to a maximum,calculate the minimum distance through which the reflecting surface $A B$ should be shifted so that the intensity at $P$ again becomes maximum.

Video Solution

Text Solution

Verified by Experts

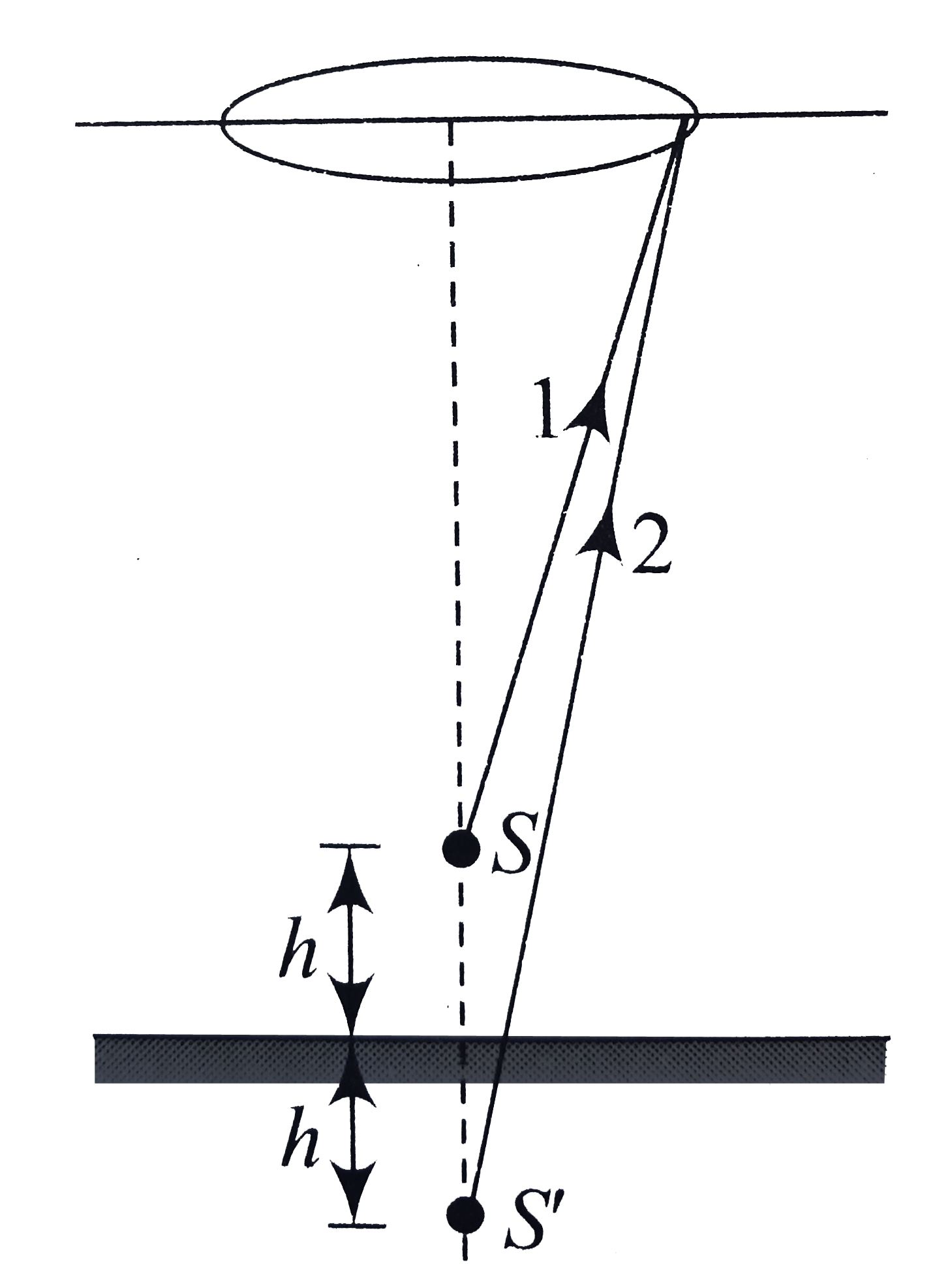
a. The path difference between the rays 1 and 2 coming from S and S' will be equal on the circular path on the screen, hence fringes will be circular.
Let the intensity of light coming from S be $I_{1} = I$ , then as per the problem, the velocity of the reflected light will be $I_{2} = 0.36 I$ .
As we know
$I_{max} = {({\sqrt{I}}_{1} + {\sqrt{I}}_{2})}_{1}^{2}$
$I_{min} = {({\sqrt{I}}_{1} - {\sqrt{I}}_{2})}_{1}^{2}$
Hence, $\frac{I_{max}}{I_{min}} = \frac{{({\sqrt{I}}_{1} + {\sqrt{I}}_{2})}_{1}^{2}}{{({\sqrt{I}}_{1} - {\sqrt{I}}_{2})}_{1}^{2}}$
$= \frac{{(\sqrt{I} + \sqrt{0.36} I)}^{2}}{{(\sqrt{I} - \sqrt{0.36} I)}^{2}} = \frac{1}{16}$
To have the next maximum at P, the path difference between the interfering waves must change by $λ$ . If AB is moved by a distance x, it will cause an additional path difference $2 x$
$2 x = λ$ (value minimum value of x)
$\Rightarrow x = \frac{λ}{2} = 300 m m$

Updated on:21/07/2023

The intensity of the light coming from one of the slits in a Young's double slit experiment is double the intensity from the other slit. Find the ratio of the maximum intensity to the minimum intensity in the interference fringe pattern observed.
A $2 : 1$
B $34 : 1$
C $9 : 1$
D $8 : 1$