A spherical mirror forms an image of magnification3. The object distance, if focal length of mirror is 24cm, may be
32cm, 24cm
32cm, 16cm
32cm only
16cm only
The correct Answer is:b.
To solve the problem, we need to find the object distance (u) given the magnification (m) and the focal length (f) of a spherical mirror. The magnification is given as 3, and the focal length is -24 cm (negative for a concave mirror).
1. Understand the Magnification Formula:
The magnification (m) is defined as the ratio of the image distance (v) to the object distance (u):
m=vu
We also know that for mirrors, magnification can be expressed in terms of focal length (f) and object distance (u):
m=ff−u
2. Set Up the Equation:
Since we know that m=3 and f=−24 cm, we can set up the equation:
3=−24−24−u
3. Cross Multiply:
To eliminate the fraction, we can cross-multiply:
3(−24−u)=−24
4. Distribute the 3:
Distributing the 3 gives:
−72−3u=−24
5. Rearrange the Equation:
Now, we can rearrange the equation to isolate u:
−3u=−24+72
−3u=48
6. Solve for u:
Dividing both sides by -3 gives:
u=−16 cm
7. Check for the Second Possible Value:
Since magnification can also be negative, we check for m=−3:
−3=−24−24−u
Cross-multiplying again:
−3(−24−u)=−24
This simplifies to:
72+3u=−24
Rearranging gives:
3u=−24−72
3u=−96
Dividing by 3:
u=−32 cm
Final Answers:
The possible object distances are:
- u=−16 cm
- u=−32 cm
The magnification (m) is defined as the ratio of the image distance (v) to the object distance (u):
m=vu
We also know that for mirrors, magnification can be expressed in terms of focal length (f) and object distance (u):
m=ff−u
Since we know that m=3 and f=−24 cm, we can set up the equation:
3=−24−24−u
To eliminate the fraction, we can cross-multiply:
3(−24−u)=−24
Distributing the 3 gives:
−72−3u=−24
Now, we can rearrange the equation to isolate u:
−3u=−24+72
−3u=48
Dividing both sides by -3 gives:
u=−16 cm
Since magnification can also be negative, we check for m=−3:
−3=−24−24−u
Cross-multiplying again:
−3(−24−u)=−24
This simplifies to:
72+3u=−24
Rearranging gives:
3u=−24−72
3u=−96
Dividing by 3:
u=−32 cm
The possible object distances are:
- u=−16 cm
- u=−32 cm
Topper's Solved these Questions
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Assertion-Reasoninig
2 Videos
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Linked Comprehension
51 Videos
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Subjective
26 Videos
- ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSEXERCISE - dpp 3.3
15 Videos
- HEATING EFFECT OF CURRENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - HEATING EFFECT OF CURRENTEXERCISE - Thermal Power in Resistance Connected in Circuit
27 Videos
Similar Questions
A spherical mirror produces an image of magnification -1, when an object is placed on the principal axis of the mirror at a distance of 40 cm form its pole.
(a) Identify the nature of image.
(b) Identify the nature of spherical mirror.
(c) Find the distance between the object and its image.
(d) How will the nature of the image change, if the object is shifted 10 cm towards the mirror ? Draw a ray diagram to justify your answer.
[Hint. (a) Image is real, inverted/same size as the object, (b) Concave mirror, (c) Zero, (d) Image will be formed beyond C, It will be larger in size.]
View SolutionA convex mirror forms the image of an object placed at a distance 40 cm in front of mirror, at distance 10 cm Find the focal length of mirror.
View SolutionA spherical mirror from image at distance 10 cm of an object at distance 30 cm from mirror. If object start moving with velocity 9cm/sec. Find velocity of image
View SolutionThe image formed by a concave mirror is twice the size of the object. The focal length of the mirror is 20 cm. The distance of the object from the mirror is/are
View SolutionAn object is present on the principal axis of a concave mirror at a distance 30cm from it. Focal length of mirror is 20cm.
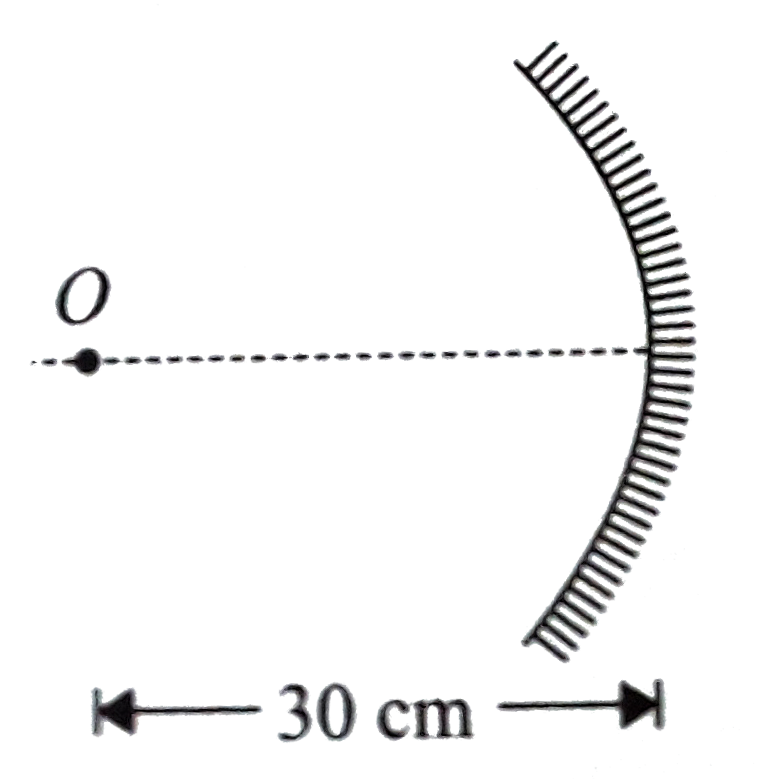
Q. Image formed by mirror is
View SolutionA spherrical mirror forms an erect image three times the size of the object, If the distance between the object and the inage is 80 cm. The nature and the focal length of the mirror are
View SolutionFor a concave mirror of focal length f, image is 2 times larger. Then the object distance from the mirror can be
View SolutionA convex mirror forms an image one-fourth the size of the object. If object is at a distance of 0.5 m from the mirror the focal length of the mirror is
View SolutionA concave mirror forms a virtual image of size twice that of the object placed at a distance 5 cm from it . Find the focal length of the mirror.
View SolutionThe image of an object formed by a mirror is real, inverted and its of magnification -1. If the image is at a distance of 40 cm from the mirror, where is the object placed ? Where would the image be if the object is moved 20 cm towards the mirror? State reason and also draw ray diagram for the new position of the object to justify your answer.
View Solution
