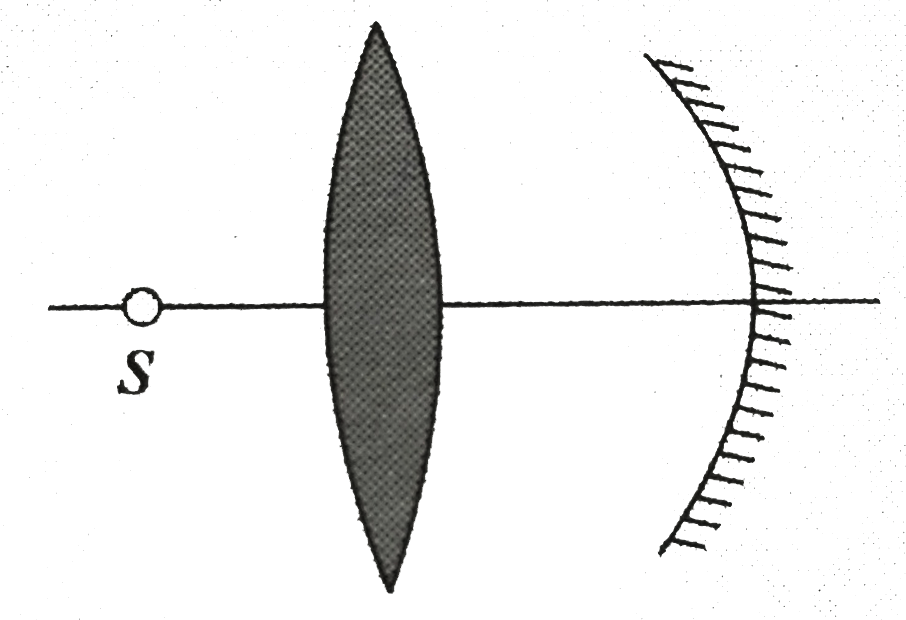
A beam of light converges to a point on a screen S. A mirror is placed in front of the screen at a distance of 10cm form the screen. It is found that the beam now converges at a point 20cm in front of the mirror. Find the focal length of the mirror.
To solve the problem, we will use the mirror formula and the information given in the question. Let's break it down step by step.
Step 1: Understand the Setup
We have a screen at the origin where light converges at point S. A mirror is placed 10 cm in front of the screen. The light beam, after reflecting off the mirror, converges at a point 20 cm in front of the mirror.
Step 2: Define the Variables
- Let the distance from the mirror to the screen be d=10 cm.
- The distance from the mirror to the point where the light converges after reflection is v=−20 cm (negative because it is in front of the mirror).
- The distance from the screen (where the light originally converged) to the mirror is the object distance for the mirror, which is u=+10 cm (positive because it is in the direction of the incoming light).
Step 3: Use the Mirror Formula
The mirror formula is given by:
1f=1v+1u
where:
- f is the focal length of the mirror,
- v is the image distance,
- u is the object distance.
Step 4: Substitute the Values
Substituting the values into the mirror formula:
1f=1−20+110
Step 5: Calculate Each Term
Calculating each term:
- 1−20=−0.05
- 110=0.1
Now, substituting these values:
1f=−0.05+0.1=0.05
Step 6: Solve for Focal Length
Now, we can find f:
f=10.05=20 cm
Step 7: Determine the Nature of the Mirror
Since the focal length is positive, the mirror is a convex mirror.
Final Answer
The focal length of the mirror is 20 cm.
---
We have a screen at the origin where light converges at point S. A mirror is placed 10 cm in front of the screen. The light beam, after reflecting off the mirror, converges at a point 20 cm in front of the mirror.
- Let the distance from the mirror to the screen be d=10 cm.
- The distance from the mirror to the point where the light converges after reflection is v=−20 cm (negative because it is in front of the mirror).
- The distance from the screen (where the light originally converged) to the mirror is the object distance for the mirror, which is u=+10 cm (positive because it is in the direction of the incoming light).
The mirror formula is given by:
1f=1v+1u
where:
- f is the focal length of the mirror,
- v is the image distance,
- u is the object distance.
Substituting the values into the mirror formula:
1f=1−20+110
Calculating each term:
- 1−20=−0.05
- 110=0.1
1f=−0.05+0.1=0.05
Now, we can find f:
f=10.05=20 cm
Since the focal length is positive, the mirror is a convex mirror.
The focal length of the mirror is 20 cm.
Topper's Solved these Questions
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Exercise1.3
25 Videos
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Exercise1.4
12 Videos
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Exercise1.1
14 Videos
- ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSEXERCISE - dpp 3.3
15 Videos
- HEATING EFFECT OF CURRENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - HEATING EFFECT OF CURRENTEXERCISE - Thermal Power in Resistance Connected in Circuit
27 Videos
Similar Questions
An object is placed at a distance of 30 cm from a concave mirror and its real image is formed at a distance of 30 cm from the mirror. The focal length of the mirror is
View SolutionA convex mirror forms the image of an object placed at a distance 40 cm in front of mirror, at distance 10 cm Find the focal length of mirror.
View SolutionA converging lens and a converging mirror are placed with their principal axis coinciding. Their separation equals 40cm. A point source S is placed on the principal axis at a distance of 12 cm from the lens as shown in Fig. It is found that the final beam comes out parallel to the principal axis. Focal length of the lens equals 15 cm. Find the focal length of the mirror.
View SolutionA beam of light converges at a point P. Now a convex lens is placed in the part of the converngent beam at 15 cm from P. At what point does a beam converge if the convex lens has a focad length 10 cm ?
View SolutionA small status has a height of 1cm and is placed in front of a spherical mirror. The image of the statue is inverted and is 0.5cm tall and located to 10cm in front of the mirror. Find the focal length and nature of the mirror.
View SolutionA point source of light is placed in front of a plane mirror.
View SolutionA beam of light converges to a point P. A lens is placed in the path of the covergent beam 12cm from P. At what point does the beam converge if the lens is
(a) a convex lens of focal length 20cm
(b) a concave lens of focal length 16cm ?
View SolutionA convergent beam of light is incident on a convex mirror so as to converge to a distance 12 cm from the pole of the mirror. An inverted image of the same size is formed coincident with the virtual object. What is the focal length of the mirror ?
View SolutionA beam of light converges to a point P.A lens is placed in the path of the convergent beam 12cm from P. At what point does the beam converge if the lens is
(a) a convex lens of focal length 20cm. (b) a concave lens of focal length 16cm ?
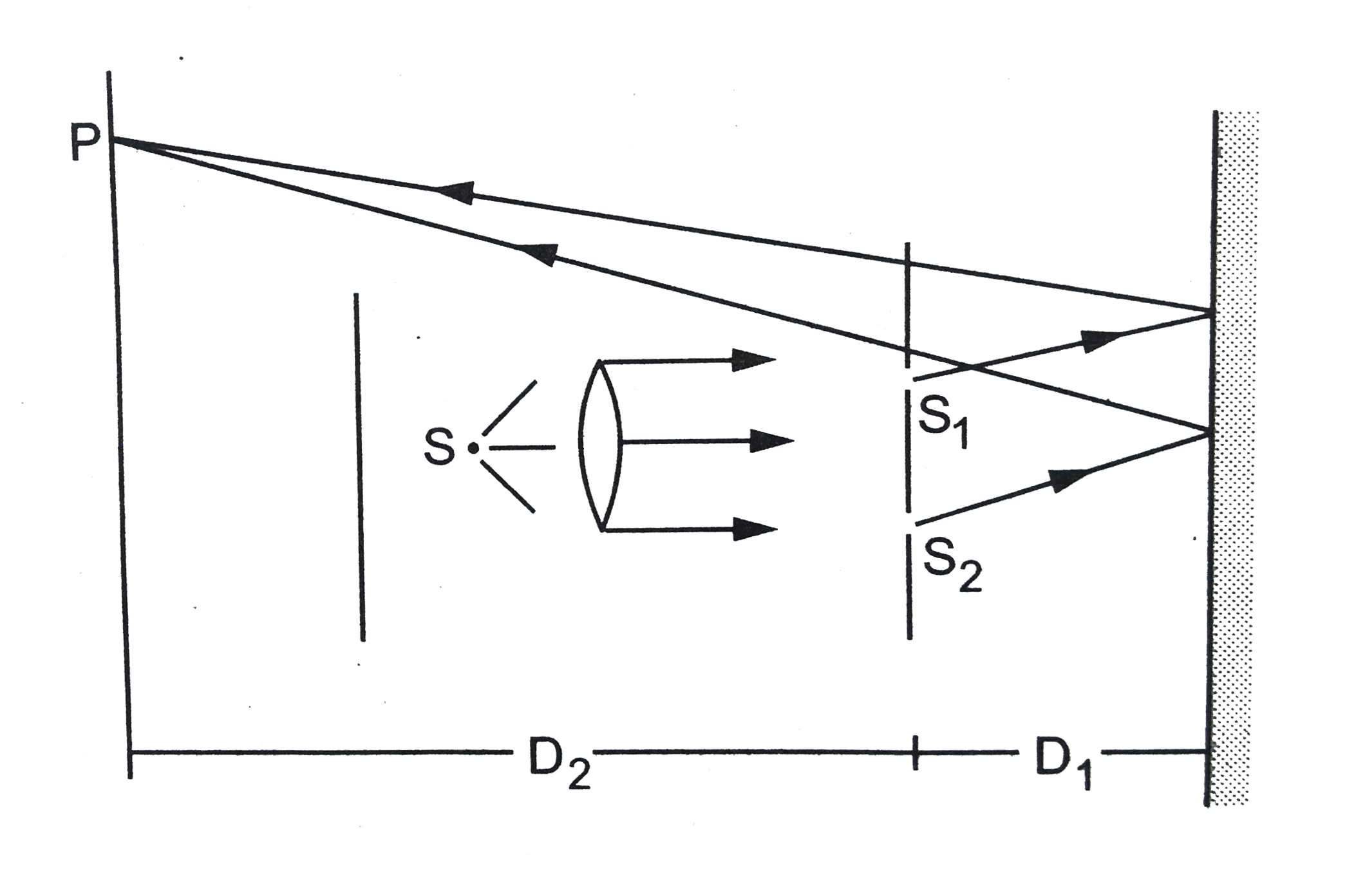
View SolutionA double slit S1−S2 is illuminated by a coherent light of wavelength λ. The slits are separated by a distance d. A plane mirror is placed in front of the double slit at a distance D1, from it and a screen ∑ is placed behind the double slit at a distance D2, from it . The screen ∑ receives only the light reflected by the mirror. Find the fringe-width of the interference pattern on the screen.
View Solution
