Question

Two small spheres are attached to the ends of a long light nonconducting rod at a distance 40 mm from each other. All three spheres are nonconducting, have identical masses $m=1g$, and a positive charge $q=1C$ is distributed evenly on the surface of each sphere. The whole system is placed on a horizontal frictionless nonconducting surface. Initially, all three spheres are at rest and the middle sphere is located a distance 30 mm from one of the ends of the rod and a distance 10 mm from the other. Find the maximum speed v $(\in m{s}^{-1})$ of the middle sphere after the system is released.

Explanation

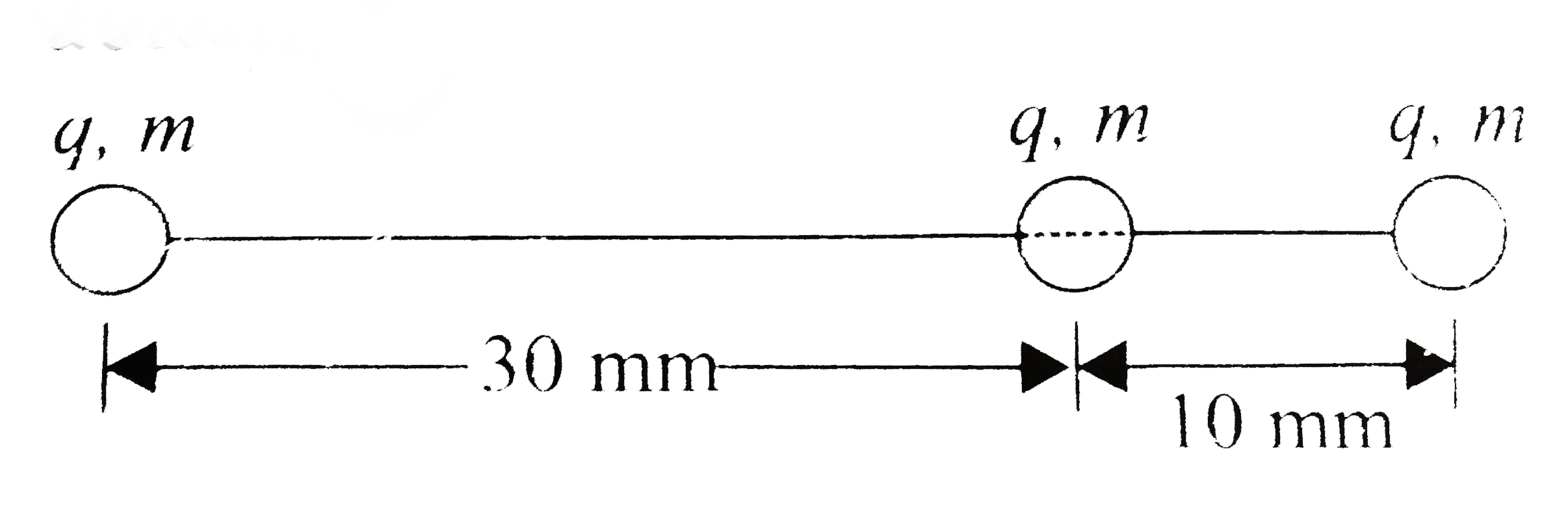
Since the charge is evely distributed, the charges can be treated as point charges. Looking at the "believe" and "after" pictures and taking right as positive and left as negative, conservation of momentum gives `sumvecP_(b)=sumvecP_(a)`
`0=2mv_(2)-mv_(1)`,which gives `v_(2)=V_(1)//2`
The maximum velocity of the middle charge will occur when the middle charge is at the middle. Beyond the middle point, there will be a net force to the right that will tend to slow the charge down. The situation will be the same for the middle charge even if the two end charges are moving. Applying the law of conservation of energy to the system and using the middle charge position of maximum velocity as the "after" position, one gets `E_(b)=E_(a)`
`PE_(b)=KE+PE_(a)`
or `(kq^(2))/(3d)+(kq^(2))/(d)=(1)/(2)mv_(1)^(2)+(1)/(2)(2m)v_(2)^(2)+(2kq^(2))/(2d)`
Now using the conservation of momentum result above for `v_(2)` and simplifying, we get
or `(kq^(2))/(3d)=(1)/(2)mv_(1)^(2)+m(v_(1)^(2))/(4)=(3mv_(1)^(2))/(4)`
Finally solving for the maximum velocity
`v_(1)=(2q)/(3) sqrt((k)/(md))=20 ms^(-1)`