The resistance of hot tungsten filament is about 10 times the cold resistance. What will be the resistance of 100 W and 200 V lamp when not in use?
14,000Ω
400Ω
40Ω
4Ω
The correct Answer is:C
To find the resistance of the 100 W and 200 V lamp when not in use, we can follow these steps:
Step 1: Understand the relationship between hot and cold resistance
The problem states that the resistance of the hot tungsten filament (R_hot) is about 10 times the cold resistance (R_cold). This can be expressed as:
Rhot=10×Rcold
Step 2: Calculate the hot resistance using the power formula
When the lamp is in use, we can calculate the hot resistance (R_hot) using the power formula. The power (P) of the lamp is given as 100 W, and the voltage (V) is given as 200 V. The relationship between power, voltage, and resistance is given by:
P=V2R
Rearranging this formula to solve for resistance gives us:
R=V2P
Step 3: Substitute the values into the formula
Now we can substitute the given values into the formula:
Rhot=(200V)2100W
Calculating this gives:
Rhot=40000V2100W=400Ω
Step 4: Calculate the cold resistance
Using the relationship established in Step 1, we can find the cold resistance (R_cold):
Rcold=Rhot10=400Ω10=40Ω
Conclusion
Thus, the resistance of the lamp when not in use (cold resistance) is:
Rcold=40Ω
---
The problem states that the resistance of the hot tungsten filament (R_hot) is about 10 times the cold resistance (R_cold). This can be expressed as:
Rhot=10×Rcold
When the lamp is in use, we can calculate the hot resistance (R_hot) using the power formula. The power (P) of the lamp is given as 100 W, and the voltage (V) is given as 200 V. The relationship between power, voltage, and resistance is given by:
P=V2R
Rearranging this formula to solve for resistance gives us:
R=V2P
Now we can substitute the given values into the formula:
Rhot=(200V)2100W
Calculating this gives:
Rhot=40000V2100W=400Ω
Using the relationship established in Step 1, we can find the cold resistance (R_cold):
Rcold=Rhot10=400Ω10=40Ω
Thus, the resistance of the lamp when not in use (cold resistance) is:
Rcold=40Ω
Topper's Solved these Questions
- HEATING EFFECT OF CURRENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - HEATING EFFECT OF CURRENTEXERCISE - Multiple Correct
5 Videos
- HEATING EFFECT OF CURRENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - HEATING EFFECT OF CURRENTEXERCISE - Assertion - Reasoning
6 Videos
- HEATING EFFECT OF CURRENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - HEATING EFFECT OF CURRENTEXERCISE - Subjective
8 Videos
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Integer Type
4 Videos
- INDUCTANCEBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - INDUCTANCEEXERCISE - Concept Based
8 Videos
Similar Questions
If resistance of the filament increases with temperature, what will be power dissipated in a 220V−100W lamp when connected to 110V power supply
View SolutionAn electric kettle is rated at 230 V, 1000 W.
What is the resistance of its element when in use ?
View SolutionWhat resistance must be connected to a 15 Ω resistance to provide an effective resistance of 6Ω ?
View SolutionThe resistance of the filament of a lamp increases with the increase in temperature. A lamp rated 100W and 220V is connected across 220V power supply. If the voltage drops by 10%, then the power of the lamp will be
View SolutionThe operating temperature of the filament of lamp is 2000∘C. The temperature coefficient of the material of the filament is 0.005∘C−1. If the atmospheric temperature is 0∘C, then the current in the 100W−200V lamp when it is switched on is nearest to
View SolutionIncandescent bulbs are designed by keeping in mind that the resistance of their filament increases with the increase in temperature. If at room temperature, 100W,60Wand40W bulbs have filament resistances R100,R60andR40, respectively, the relation between these resistances is
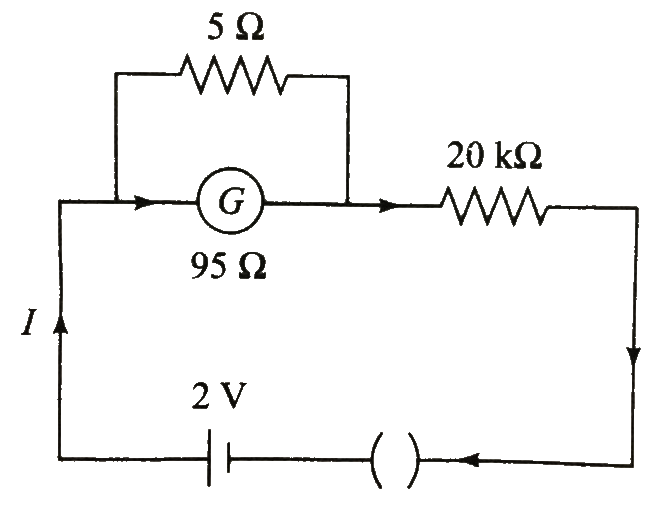
View SolutionA galvanometer of resistance 95Ω, shunted resistance of 5Ω, gives a deflection of 50 divisions when joined in series with a resistance of 20kΩ and a 2V accumulator. What is the current sensitivity of the galvanomter (in div/μA)?
View SolutionIn a wheatstone bridge resistance of each of the four sides is 10Ω. If the resistance of the galvanometer is also 10Ω , then effective resistance of the bridge will be
View SolutionFind the resistance of a copper coil of total wire-length 10m and area of cross section 1.0mm^(2). What would be the resistance of a similar coil of aluminium? The resistivity of copper =1.7×10−8(Ω)m and that of aluminium2.6×10−8(Ω).
View SolutionThe current through tungsten filament lamp connected to a 12 V accumulator of negligible resistance is 3.0 A. Calculate :
the resistance of the filament,
View Solution
