Three charges each of value q are placed at the corners of an equilateral triangle. A fourth charge Q is placed at the center of the triangle.
a. Find the net force on charge q.
b. If Q=−q, will the charges at the corners move toward the center or fly away from it ?
c. For what value of Q at O will the charges remain stationary ?
d. In situation (c), how much work is done in removing the charges to infinity?
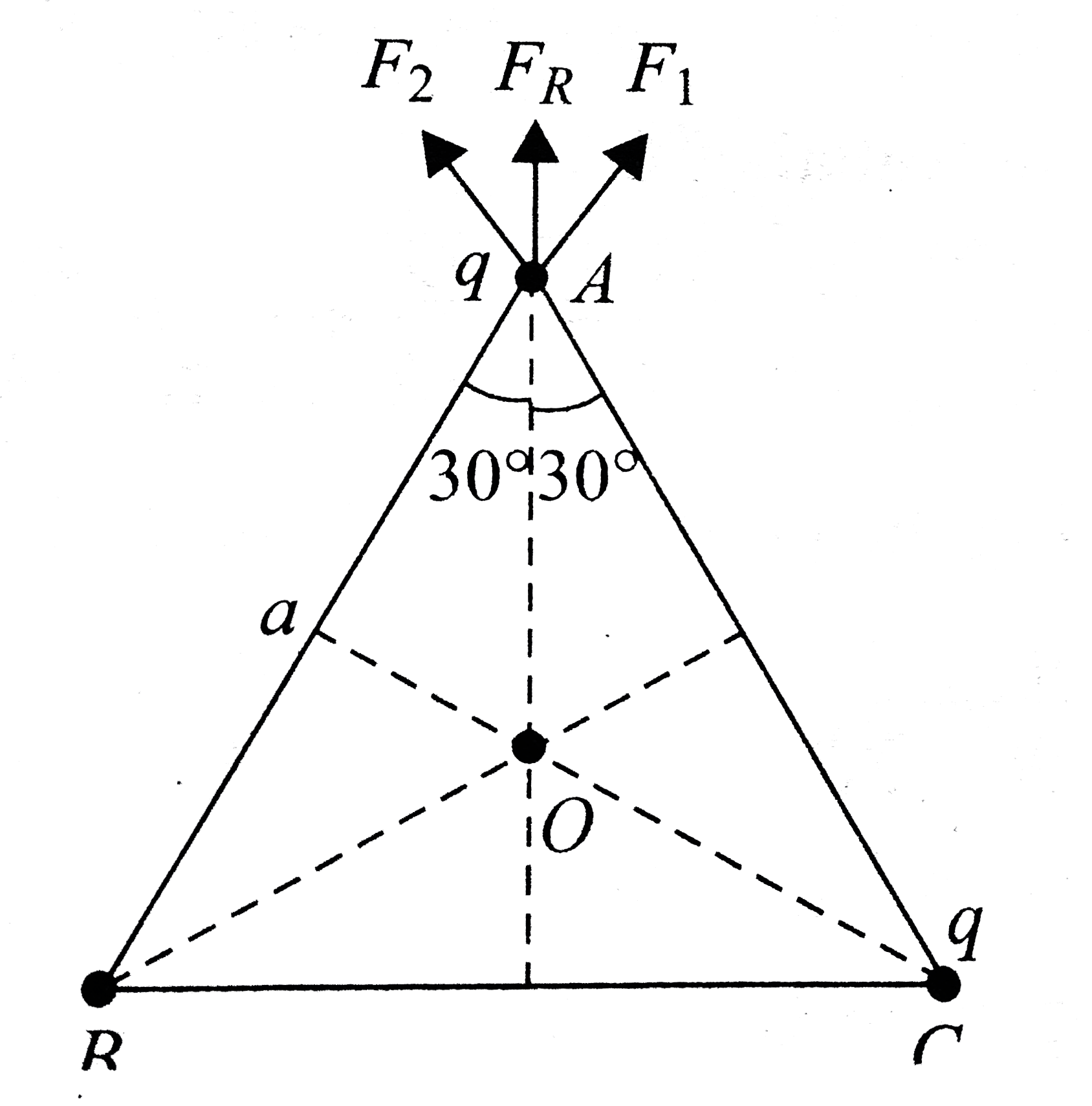
The correct Answer is:a. FR=q4πε0a2[√3q+3Q] along −−→OA
b. the charges q at corners A,B and C will move towards the center O.
c. Q=−(q√3)
d. zero
A If Q is a positive charge, the resultant force on q at A will be
→FR=→F1+→F2+→F3
Here, F1=F2=14πε0q2a2
So, (→F1+→F2)=2×14πε0q2a2cos30∘=√3q24πε0a2 along −−→OA
while →F3=14πε0qQ(a/√3)2=3qQ4πε0a2 along −−→OA
 .
.
So, FR=q4πε0a2[√3q+3Q] along −−→OA .... (i)
b. For Q=−q,
FR=q4πε0a2[√3q−3a] along −−→OA
=√3q4πε0a2[√3−1] along −−→AO
i.e., the charges q at corners A, B and C will move toward the center O.
c. Charges will remain stationary if FR=0, which Eq. (i) is possible only if
[(√3)q+3Q]=0, i,e., Q=−(q√3)
d. Potential energy of the system
U=12×14πε0∑i≠jqiqjrij=14πε0⎡⎢
⎢⎣3q×qa+3q(−q/√3)(a/√3)⎤⎥
⎥⎦=0
And for finite charges, distribution potential energy at infinity is always zero. So
W=UF−UI=0−0=0.
Topper's Solved these Questions
- ELECTRIC POTENTIALBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC POTENTIALEXERCISE - Single Correct
56 Videos
- ELECTRIC POTENTIALBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC POTENTIALEXERCISE - Multiple Correct
10 Videos
- ELECTRIC POTENTIALBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC POTENTIALEXERCISE - Exercise 3.3
9 Videos
- ELECTRIC FLUX AND GAUSS LAWBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC FLUX AND GAUSS LAWEXERCISE - MCQ s
38 Videos
- ELECTRICAL MEASURING INSTRUMENTSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRICAL MEASURING INSTRUMENTSEXERCISE - M.C.Q
2 Videos
Similar Questions
Three equal charges, each +q are placed on the corners of an equilatreal triangel . The electric field intensity at the centroid of the triangle is
View SolutionFive equal charges each of value q are placed at the corners of a regular pentagon of side a. The electric field at the centre of the pentagon is

View Solution
Knowledge Check
Electric charges q,q,–2q are placed at the corners of an equilateral triangle ABC of side l. The magnitude of electric dipole moment of the system is
AqlB√3qlCzeroD4qlConsider the charges q,q and -q placed at the vertices of an equilateral triangle of each side l. What is the force on each charge ?
Aq24√2πε0l2B−q24πε0l2Cq24πε0l2Dzero
Three charges 2q,−q,and−q are located at the vertices of an equilateral triangle. At the center of the triangle,
View SolutionThree charges +q, -q and +q are kept at the corners of an equilateral triangle of side d. Find the resultant electric force on a charge +q placed at the centroid O of the triangle.
View SolutionThree equal charge q0 each are placed at three corners of an equilateral triangle of side 'a'. Find out force acting on one of the charge due to other two charges ?
View SolutionThree poistive charges of equal value q are placed at the vertices of an equilateral triangle. The resulting lines of force should be sketched as in
View SolutionElectric charges q,q,–2q are placed at the corners of an equilateral triangle ABC of side l. The magnitude of electric dipole moment of the system is
View SolutionThree charges, each equal to q, are placed at the three. corners of a square of side a . Find the electric field at. the fourth corner.
View Solution