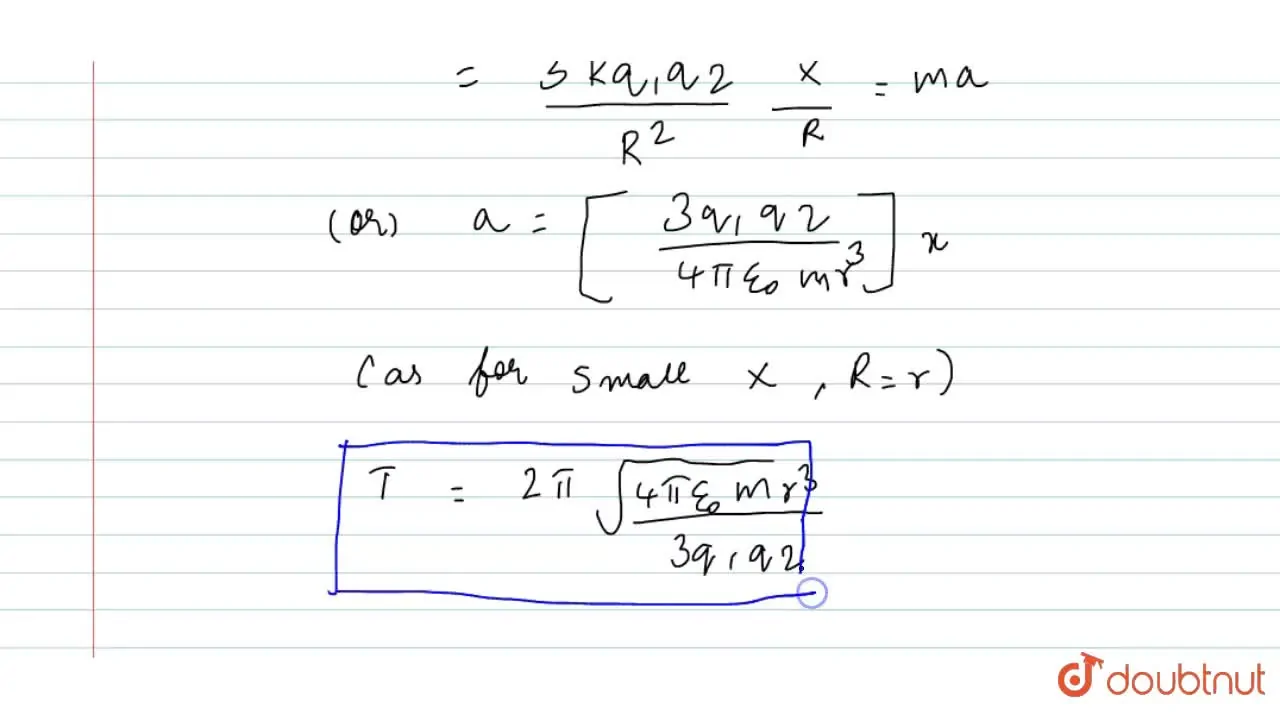
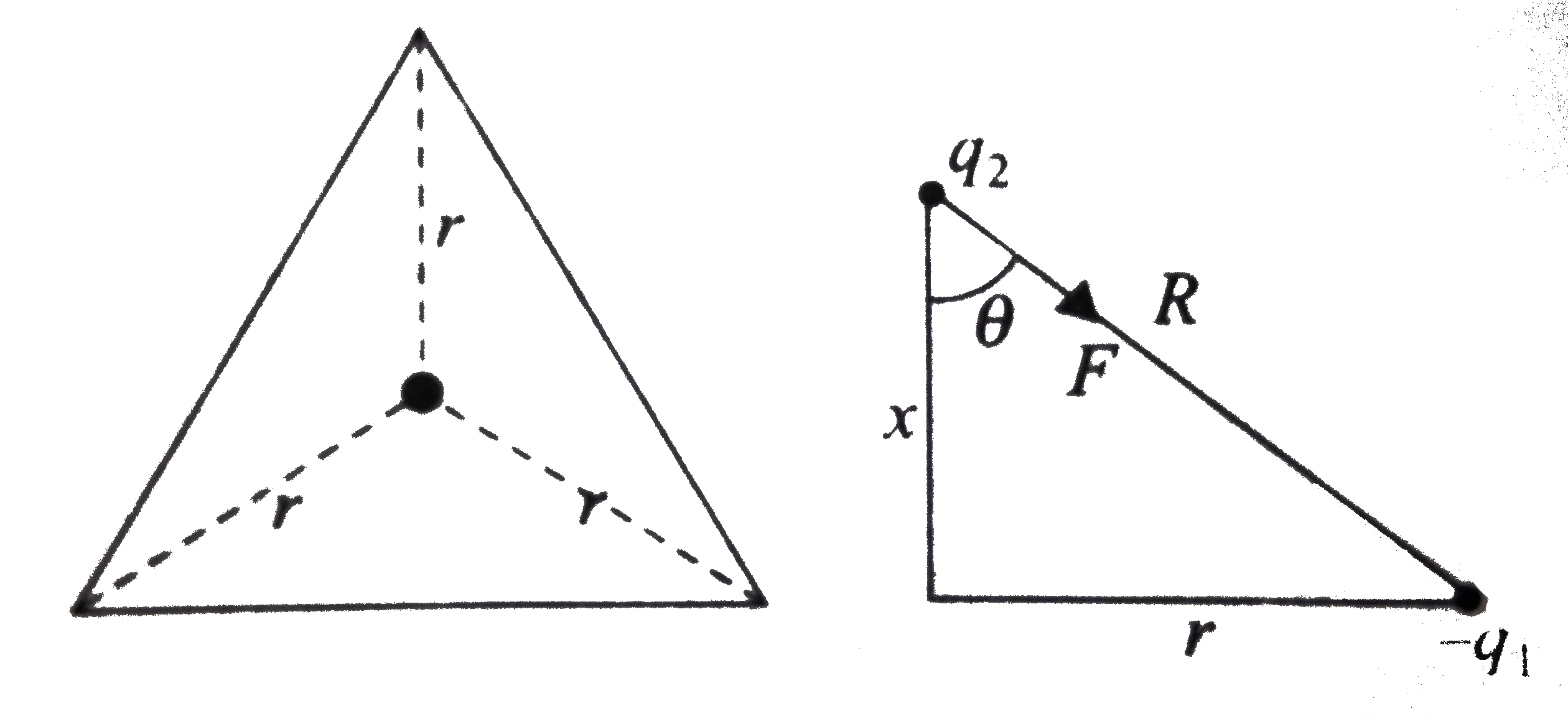
Three equal negative charges, $- q_{1}$ each form the vertices of and equilateral triangle . A particle of mass m and a positive charge $q_{2}$ is constrained to move along a line perpendicular to the plane of triangle and through its center, which at a distance r from each of the negative charges as shown in fig. The whole systen is kept in gravity-free space, Find the time period of vibration of the particle for small displacement from the equilibrium position.

Video Solution

Text Solution

Verified by Experts

The correct Answer is: $2 π \sqrt{\frac{4 π ε_{0} m r^{3}}{3 q_{1} q_{2}}}$

$(F_{net}) = 3 F cos θ = \frac{3 k q_{1} q_{2}}{R^{2}} \frac{x}{R} = m a$
or, $a = [\frac{3 k q_{1} q_{2}}{4 π ε_{0} m r^{3}}] x$ (as for small x, R=r)
$T = 2 π \sqrt{\frac{4 π ε_{0} m r^{3}}{3 k q_{1} q_{2}}}$
,

Updated on:21/07/2023

|

Class 12 PHYSICS ELECTROSTATICS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Consider the charges q,q and -q placed at the vertices of an equilateral triangle of each side l. What is the force on each charge ?
A $\frac{q^{2}}{4 \sqrt{2} π ε_{0} l^{2}}$
B $\frac{- q^{2}}{4 π ε_{0} l^{2}}$
C $\frac{q^{2}}{4 π ε_{0} l^{2}}$
Dzero

CENGAGE PHYSICS ENGLISH-COULOMB LAW AND ELECTRIC FIELD-Subjective

Home
Ask Doubt
Profile