The bar shown in the figure is made of a single piece of material. It is fixed at one end. It consists of two segments of equal lengh L/2 each but different cross sectional area A and 2A. Find the ratio of total elongation in the bar to the elongation produced in thicker segment under the action of an axial force F. Consider the shape of the joint to remain circular. (Given :Y is Young's modulus.
The correct Answer is:3
To solve the problem, we need to find the ratio of the total elongation in the bar to the elongation produced in the thicker segment under the action of an axial force F. The bar consists of two segments of equal length L2 but different cross-sectional areas A (thinner segment) and 2A (thicker segment).
1. Understanding the Setup:
- The bar is fixed at one end and has two segments of equal length L2.
- The first segment has a cross-sectional area A and the second segment has a cross-sectional area 2A.
2. Using the Formula for Elongation:
- The elongation ΔL in a segment can be calculated using the formula:
ΔL=F⋅LA⋅Y
- Where F is the axial force, L is the length of the segment, A is the cross-sectional area, and Y is Young's modulus.
3. Calculating Elongation in Each Segment:
- For the first segment (cross-sectional area A):
- Length of the segment = L2
- Elongation ΔL1:
ΔL1=F⋅L2A⋅Y=F⋅L2A⋅Y
- For the second segment (cross-sectional area 2A):
- Length of the segment = L2
- Elongation ΔL2:
ΔL2=F⋅L22A⋅Y=F⋅L4A⋅Y
4. Total Elongation in the Bar:
- The total elongation ΔLtotal in the bar is the sum of the elongations in both segments:
ΔLtotal=ΔL1+ΔL2=F⋅L2A⋅Y+F⋅L4A⋅Y
- To add these fractions, we need a common denominator:
ΔLtotal=2F⋅L4A⋅Y+F⋅L4A⋅Y=3F⋅L4A⋅Y
5. Finding the Ratio of Total Elongation to Elongation in Thicker Segment:
- We need to find the ratio ΔLtotalΔL2:
ΔLtotalΔL2=3F⋅L4A⋅YF⋅L4A⋅Y=3F⋅L4A⋅Y⋅4A⋅YF⋅L=3
Final Answer:
The ratio of the total elongation in the bar to the elongation produced in the thicker segment is 3.
- The bar is fixed at one end and has two segments of equal length L2.
- The first segment has a cross-sectional area A and the second segment has a cross-sectional area 2A.
- The elongation ΔL in a segment can be calculated using the formula:
ΔL=F⋅LA⋅Y
- Where F is the axial force, L is the length of the segment, A is the cross-sectional area, and Y is Young's modulus.
- For the first segment (cross-sectional area A):
- Length of the segment = L2
- Elongation ΔL1:
ΔL1=F⋅L2A⋅Y=F⋅L2A⋅Y
- Length of the segment = L2
- Elongation ΔL2:
ΔL2=F⋅L22A⋅Y=F⋅L4A⋅Y
- The total elongation ΔLtotal in the bar is the sum of the elongations in both segments:
ΔLtotal=ΔL1+ΔL2=F⋅L2A⋅Y+F⋅L4A⋅Y
- To add these fractions, we need a common denominator:
ΔLtotal=2F⋅L4A⋅Y+F⋅L4A⋅Y=3F⋅L4A⋅Y
- We need to find the ratio ΔLtotalΔL2:
ΔLtotalΔL2=3F⋅L4A⋅YF⋅L4A⋅Y=3F⋅L4A⋅Y⋅4A⋅YF⋅L=3
The ratio of the total elongation in the bar to the elongation produced in the thicker segment is 3.
Topper's Solved these Questions
- MISCELLANEOUS VOLUME 2Book:CENGAGE PHYSICS ENGLISHChapter:MISCELLANEOUS VOLUME 2Exercise:LC_TYPE
Explore 34 Videos
- MISCELLANEOUS KINEMATICSBook:CENGAGE PHYSICS ENGLISHChapter:MISCELLANEOUS KINEMATICSExercise:Interger type
Explore 3 Videos
- NEWTON'S LAWS OF MOTION 1Book:CENGAGE PHYSICS ENGLISHChapter:NEWTON'S LAWS OF MOTION 1Exercise:Integer
Explore 5 Videos
Similar Questions
The bar shown in the figure is made of a single piece of material. It is fixed at one end and consists of two segments of equal length L2 but different cross-section area A and 2A. What is the change in length of the system under the action of an axial force F. [ consider the shape of joint to remain circular, Y is young's modulus]

View SolutionA uniform cylindrical rod of length L and cross-sectional area by forces as shown in figure.
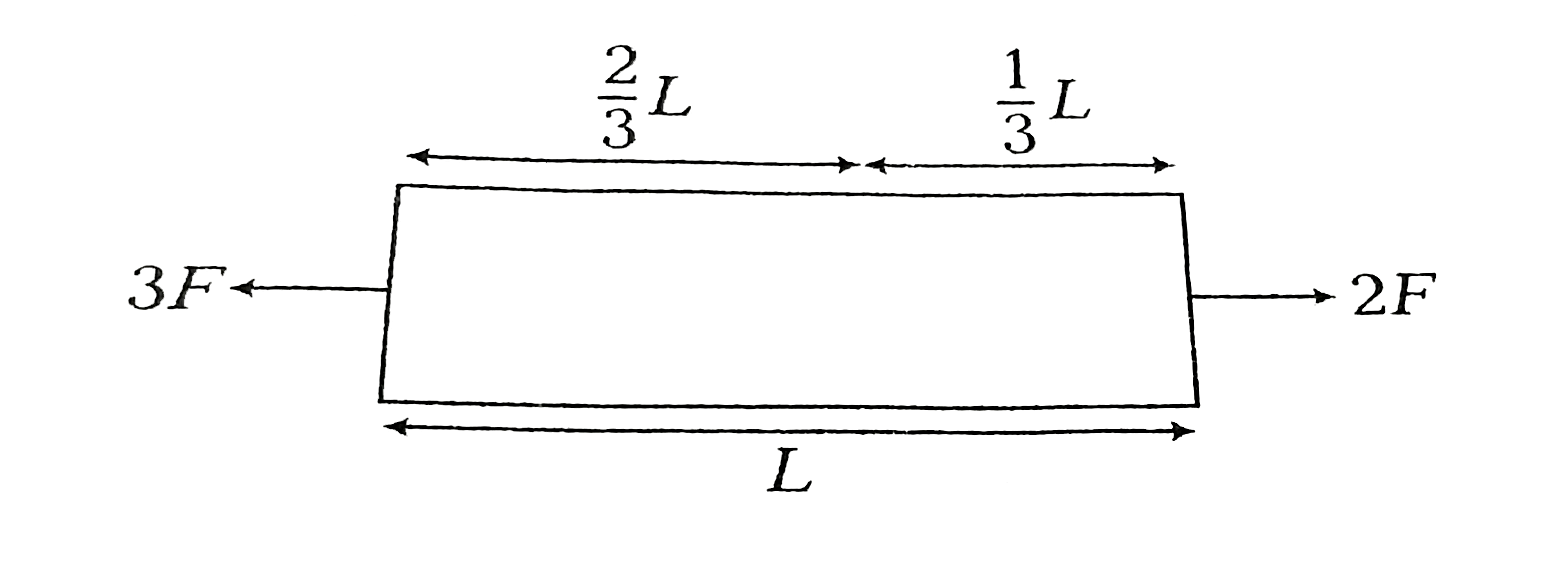
The elongation produced in the rod is
View Solution
Knowledge Check
A wire of length L and area of cross-section A, is stretched by a load. The elongation produced in the wire is I. If Y is the Young's modulus of the material of the wire, then the force constant of the wire is
AYLABYlACYALDYAl
A uniform heavy rod of weight W, cross sectional area a and length L is hanging from fixed support. Young modulus of the material of the rod is Y. Neglect the lateral contraction. Find the elongation of the rod.
View SolutionTwo wires of the same material and length are stretched by the same force. Their masses are in the ratio 3:2. Their elongations are in the ratio
View SolutionA steel bar of cross-sectional area A and length l is magnetised to develop an intensity of magnetisation l . The pole strength of the bar is
View SolutionTwo wires of the same length and same material but radii in the ratio of 1:2 are stretched by unequal forces to produce equal elongation. The ratio of the two forces is
View SolutionA metallic rod of length l and cross-sectional area A is made of a material of Young's modulus Y. If the rod is elongated by an amount y,then the work done is proportional to
View SolutionTwo wires of the same material and same mass are stretched by the same force. Their lengths are in the ratio 2:3. Their elongation are in the ratio
View SolutionTwo wires A and B are made of same material. The wire A has a length l and diameter r while the wire B has a length 2l and diameter r/2. If the two wires are stretched by the same force the elongation in A divided by the elongation in B is
View Solution