A paper disc of radius R from which a hole of radius r is cut out is floating in a liquid of the surface tension S. The force on the disc due to the surface tension is
S×2πR
S×2πr
S×2π(R−r)
S×2π(R+r)
The correct Answer is:D
To find the force on a paper disc with a hole cut out of it, floating in a liquid with surface tension S, we will follow these steps:
Step 1: Understand the Geometry of the Problem
The paper disc has a radius R and a hole with a radius r cut out from it. The effective area of the disc that is in contact with the liquid is the circumference of the outer circle minus the circumference of the inner circle (the hole).
Step 2: Calculate the Length in Contact with the Liquid
The length of the circumference of the outer circle (the disc) is given by:
Louter=2πR
The length of the circumference of the inner circle (the hole) is given by:
Linner=2πr
The effective length in contact with the liquid is:
Leffective=Louter−Linner=2πR−2πr=2π(R−r)
Step 3: Apply the Formula for Surface Tension Force
The force due to surface tension F is given by the product of surface tension S and the length in contact with the liquid:
F=S⋅Leffective
Substituting the effective length we found:
F=S⋅2π(R−r)
Step 4: Final Expression for the Force
Thus, the force on the disc due to the surface tension is:
F=2πS(R−r)
The paper disc has a radius R and a hole with a radius r cut out from it. The effective area of the disc that is in contact with the liquid is the circumference of the outer circle minus the circumference of the inner circle (the hole).
The length of the circumference of the outer circle (the disc) is given by:
Louter=2πR
The length of the circumference of the inner circle (the hole) is given by:
Linner=2πr
The effective length in contact with the liquid is:
Leffective=Louter−Linner=2πR−2πr=2π(R−r)
The force due to surface tension F is given by the product of surface tension S and the length in contact with the liquid:
F=S⋅Leffective
Substituting the effective length we found:
F=S⋅2π(R−r)
Thus, the force on the disc due to the surface tension is:
F=2πS(R−r)
Topper's Solved these Questions
- PROPERTIES OF SOLIDS AND FLUIDSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - PROPERTIES OF SOLIDS AND FLUIDSEXERCISE - Multiple Correct
17 Videos
- PROPERTIES OF SOLIDS AND FLUIDSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - PROPERTIES OF SOLIDS AND FLUIDSEXERCISE - Assertion- Reasoning
13 Videos
- PROPERTIES OF SOLIDS AND FLUIDSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - PROPERTIES OF SOLIDS AND FLUIDSEXERCISE - Subjective
16 Videos
- NEWTON'S LAWS OF MOTION 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Integer type
1 Video
- RIGID BODY DYNAMICS 1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 1EXERCISE - Integer
11 Videos
Similar Questions
Which of the fact is not due to surface tension
View SolutionFigure shows a uniform disc of radius R, from which a hole of radius R2 has been cut out from left of the centre and is placed on the right of the centre of the disc. Find the CM of the resulting disc.

View SolutionWhat is the force of surface tension on a light ring of radius r floating on a liquid of surface tension γ ?
View SolutionA square wire frame of side / is floating on the surface of liquid of surface tension T. The force required to pull out the frame from the liquid is
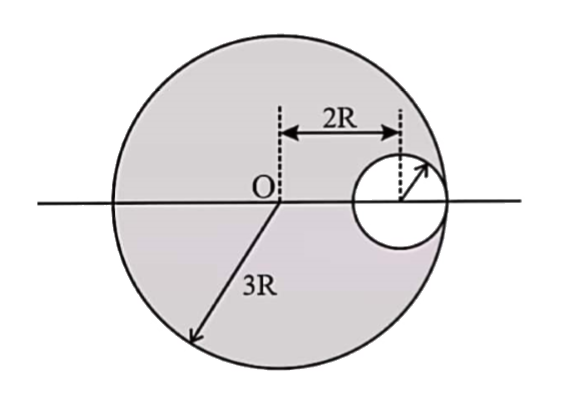
View SolutionThe figure shows a disc of radius 3R from which a circular hole of radius R is cut as shown in the figure. The distance of the centre of mass of the remaining object from the point O is
View SolutionName the force responsible for surface tension in liquids.
View SolutionAn annular disc of radius r1=10cm and r2=5cm is placed on a water surface. Find the surface tension force on the disc if we want to pull it from water surface. Take coefficient of surface tension as σ=7×10−3N/m,g=10ms−12.

View SolutionAn annular disc of radius r1=10cm and r2=5cm is placed on a water surface. Find the surface tension force on the disc if we want to pull it from water surface. Take coefficient of surface tension as σ=72dy≠/cm,g=10ms−2.

View SolutionAir is pushed into a soap bubble of radius r to double its radius. If the surface tension of the soap solution is S, the work done in the process is
View SolutionA needle of length l and density ρ will float on a liquid of surface tension σ if its radius r is less than or equal to:
View Solution
