Two glass plates are separated by water. If surface tension of water is 75dyn/cm and the area of each plate wetted by water is 8cm2 and the distance between the plates is 0.12mm, then the force applied to separate the two plates is
102dyn
104dyn
105dyn
106dyn
The correct Answer is:C
To find the force applied to separate the two glass plates that are separated by water, we can use the concept of surface tension. The force due to surface tension can be calculated using the formula:
F=2×γ×L
Where:
- F is the force,
- γ is the surface tension of the liquid (water in this case),
- L is the length of the wetted perimeter in contact with the liquid.
1. Identify the given values:
- Surface tension of water, γ=75dyn/cm
- Area of each plate, A=8cm2
- Distance between the plates, d=0.12mm=0.012cm (conversion from mm to cm)
2. Calculate the wetted length L:
- Since the area A is given, we can find the wetted length L using the formula:
A=L×d
Rearranging gives:
L=Ad
Substituting the values:
L=8cm20.012cm=80.012≈666.67cm
3. Calculate the force F:
- Now we can substitute L and γ into the force formula:
F=2×γ×L=2×75dyn/cm×666.67cm
F=150×666.67≈100000dyn
4. Convert the force to appropriate units if necessary:
- The force calculated is already in dynes, which is suitable for this context.
5. Final Answer:
- The force applied to separate the two plates is approximately 100000dyn.
- F is the force,
- γ is the surface tension of the liquid (water in this case),
- L is the length of the wetted perimeter in contact with the liquid.
- Surface tension of water, γ=75dyn/cm
- Area of each plate, A=8cm2
- Distance between the plates, d=0.12mm=0.012cm (conversion from mm to cm)
- Since the area A is given, we can find the wetted length L using the formula:
A=L×d
Rearranging gives:
L=Ad
Substituting the values:
L=8cm20.012cm=80.012≈666.67cm
- Now we can substitute L and γ into the force formula:
F=2×γ×L=2×75dyn/cm×666.67cm
F=150×666.67≈100000dyn
- The force calculated is already in dynes, which is suitable for this context.
- The force applied to separate the two plates is approximately 100000dyn.
Topper's Solved these Questions
- PROPERTIES OF SOLIDS AND FLUIDSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - PROPERTIES OF SOLIDS AND FLUIDSEXERCISE - Multiple Correct
17 Videos
- PROPERTIES OF SOLIDS AND FLUIDSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - PROPERTIES OF SOLIDS AND FLUIDSEXERCISE - Assertion- Reasoning
13 Videos
- PROPERTIES OF SOLIDS AND FLUIDSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - PROPERTIES OF SOLIDS AND FLUIDSEXERCISE - Subjective
16 Videos
- NEWTON'S LAWS OF MOTION 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Integer type
1 Videos
- RIGID BODY DYNAMICS 1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 1EXERCISE - Integer
11 Videos
Similar Questions
Find the charge on each plate given area of each plate is A and separation between two consecutive plates is d.

View SolutionWhat is the area of the plates of a 2 farad parallel plate air capacitor, given that the separation between the plates is 0.5 cm?
View SolutionWhat is the area of the plates of a 2 farad parallel plate air capacitor, given that the separation between the plates is 0.5 cm?
View SolutionIf a water drop is kept between two glass plates, then its shape is
View SolutionTwo metal plates of area 2×10−4m2 each, are kept in water and one plate is moved over the other with a certain velocity. The distance between the plates is 2×10−4m. If the horizontal force applied to move the plate is 10−3N, calculate the velocity of the plate. Given η of water is10−3 decapoise.
View SolutionTwo vertical parallel glass plates are partially submerged in water. The distance between the plates is d and the length is l. Assume that the water between the plates does not reach the upper edges of the plates and the plates and the wetting is complete. The water will rise to height (ρ= density of water and α= surface tension of water)
View SolutionTwo plates are at potentials −10V and +30V. If the separation between the plates be 2cm. The electric filed between them is
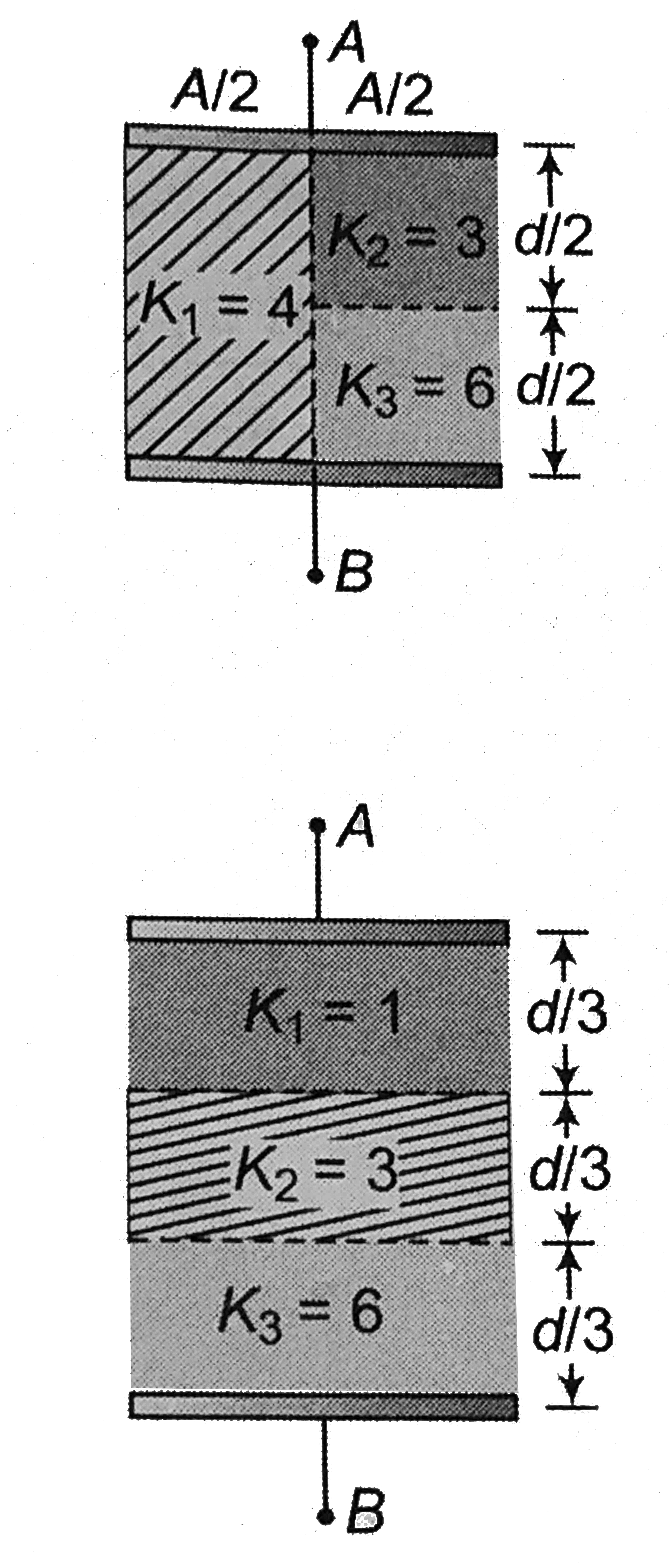
View SolutionFind the equivalent capacitance between A and B.A: area of each plate, d: separation between the plates.
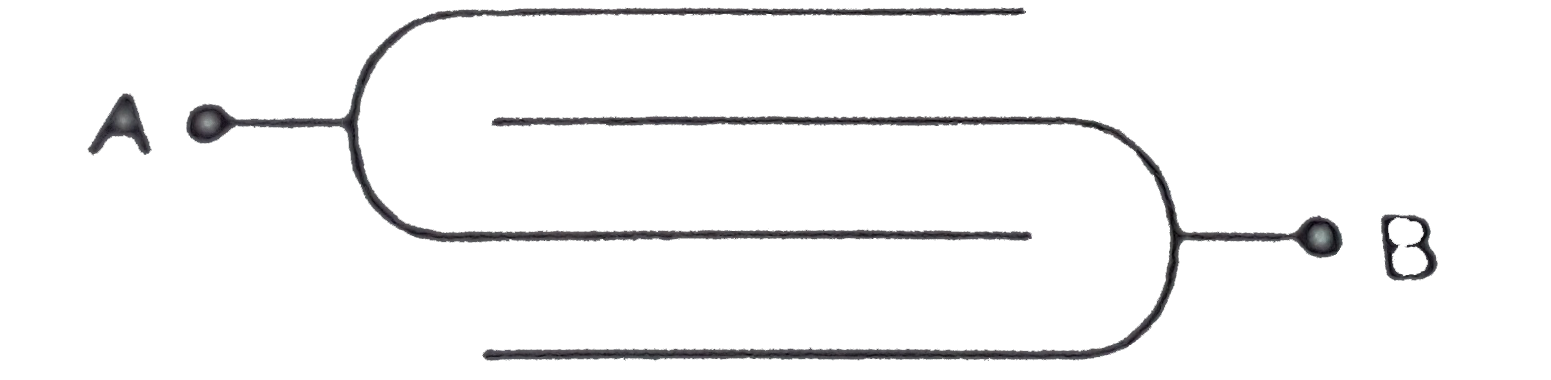
View SolutionFind out equivalent capacitance between A and B. (take each plate Area = A and distance between two conjugative plates is d)
View SolutionA small drop of water kept pressed between two glass plates spreads as a circle of diameter 10cm. If the distance between the plates is 0.005 mm, and surface tension of water is 0.072Nm−1 , what force will be required to separate the plates ?
View Solution
