A horizontal aluminum rod of diameter projects from a wall. A object is suspended from the end of the rod. The shear modulus of aluminum is . Neglecting the mass of the rod find a shearing stress on the rod and b the vertical deflection of the end of the rod.
To solve the problem step by step, we will calculate the shearing stress on the rod and then find the vertical deflection of the end of the rod.
Step 1: Calculate the Force Acting on the Rod
The force F acting on the rod due to the suspended mass can be calculated using the formula:
F=m⋅g
where:
- m=1200kg (mass of the object)
- g=10m/s2 (acceleration due to gravity)
Substituting the values:
F=1200kg⋅10m/s2=12000N
Step 2: Calculate the Area of the Rod
The area A of the rod's cross-section can be calculated using the formula for the area of a circle:
A=π4d2
where:
- d=4.8cm=0.048m (diameter of the rod)
Substituting the value:
A=π4(0.048)2≈3.144(0.002304)≈0.00018096m2
Step 3: Calculate the Shearing Stress
Shearing stress τ can be calculated using the formula:
τ=FA
Substituting the values:
τ=12000N0.00018096m2≈6.63×106N/m2
Step 4: Calculate the Shear Strain
The shear strain γ can be calculated using the relationship:
γ=τG
where G is the shear modulus. Given G=3.0×1010N/m2:
γ=6.63×1063.0×1010≈2.21×10−4
Step 5: Calculate the Vertical Deflection
The vertical deflection ΔL can be calculated using the formula:
ΔL=γ⋅L
where L=5.3cm=0.053m:
ΔL=2.21×10−4⋅0.053≈1.17×10−5m
Final Results
- Shearing Stress: τ≈6.63×106N/m2
- Vertical Deflection: ΔL≈1.17×10−5m
The force F acting on the rod due to the suspended mass can be calculated using the formula:
F=m⋅g
where:
- m=1200kg (mass of the object)
- g=10m/s2 (acceleration due to gravity)
F=1200kg⋅10m/s2=12000N
The area A of the rod's cross-section can be calculated using the formula for the area of a circle:
A=π4d2
where:
- d=4.8cm=0.048m (diameter of the rod)
A=π4(0.048)2≈3.144(0.002304)≈0.00018096m2
Shearing stress τ can be calculated using the formula:
τ=FA
Substituting the values:
τ=12000N0.00018096m2≈6.63×106N/m2
The shear strain γ can be calculated using the relationship:
γ=τG
where G is the shear modulus. Given G=3.0×1010N/m2:
γ=6.63×1063.0×1010≈2.21×10−4
The vertical deflection ΔL can be calculated using the formula:
ΔL=γ⋅L
where L=5.3cm=0.053m:
ΔL=2.21×10−4⋅0.053≈1.17×10−5m
- Shearing Stress: τ≈6.63×106N/m2
- Vertical Deflection: ΔL≈1.17×10−5m
Topper's Solved these Questions
- PROPERTIES OF SOLIDS AND FLUIDSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - PROPERTIES OF SOLIDS AND FLUIDSEXERCISE - Solved Examples
11 Videos
- PROPERTIES OF SOLIDS AND FLUIDSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - PROPERTIES OF SOLIDS AND FLUIDSEXERCISE - Exercise 5.1
12 Videos
- NEWTON'S LAWS OF MOTION 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Integer type
1 Videos
- RIGID BODY DYNAMICS 1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 1EXERCISE - Integer
11 Videos
Similar Questions
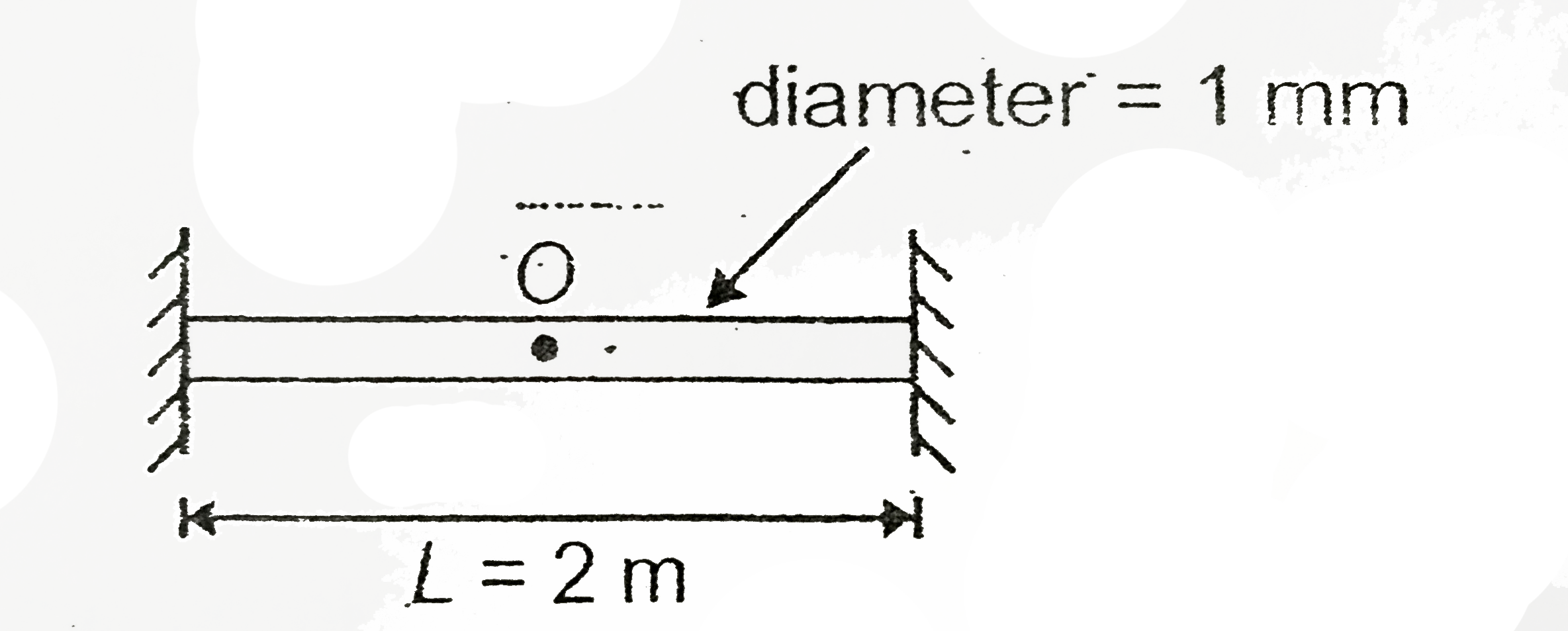
Find the net vertical displacement of point O, when a mass of 2.5 kg is suspended from the mid-point O of the rod shown (Y=2×1011N/m2).
View SolutionThe edges of an aluminum cube are 10cm long. One face of the cube is firmly fixed to a vertical wall. A mass of 100kg is then attached to the opposite face of the cube. Shear modulus of aluminum is 25×109Pa, the vertical deflection in the face to which mass is attached is
View Solution
Knowledge Check
The edges of an aluminum cube are 10cm long. One face of the cube is firmly fixed to a vertical wall. A mass of 100kg is then attached to the opposite face of the cube. Shear modulus of aluminum is 25×109Pa, the vertical deflection in the face to which mass is attached is
A4×10−5mB4×10−6mC4×10−7mD4×10−8m
A metal rod of length 50cm having mass 2kg is supported on two edges placed 10cm from each end. A 3kg load is suspended at 20cm from one end. Find the reactions at the edges (take g=10m/s2)
View SolutionA metal rod of length 50cm having mass 2kg is supported on two edges placed 10cm from each end. A 3kg load is suspended at 20cm from one end. Find the reactions at the edges (take g=10m/s2)
View SolutionThe moment of inertia of a uniform rod of mass 0.50 kg and length 1 m is 0.10 kg m^2 about a line perpendicular to the rod. Find the distance of this line from the middle point of the rod.
View SolutionThe length of a rod is 20cm and area of cross-section 2cm2. The Young's modulus of the material of wire is 1.4×1011N/m2. If the rod is compressed by 5kg−wt along its length, then increase in the energy of the rod in joules will be
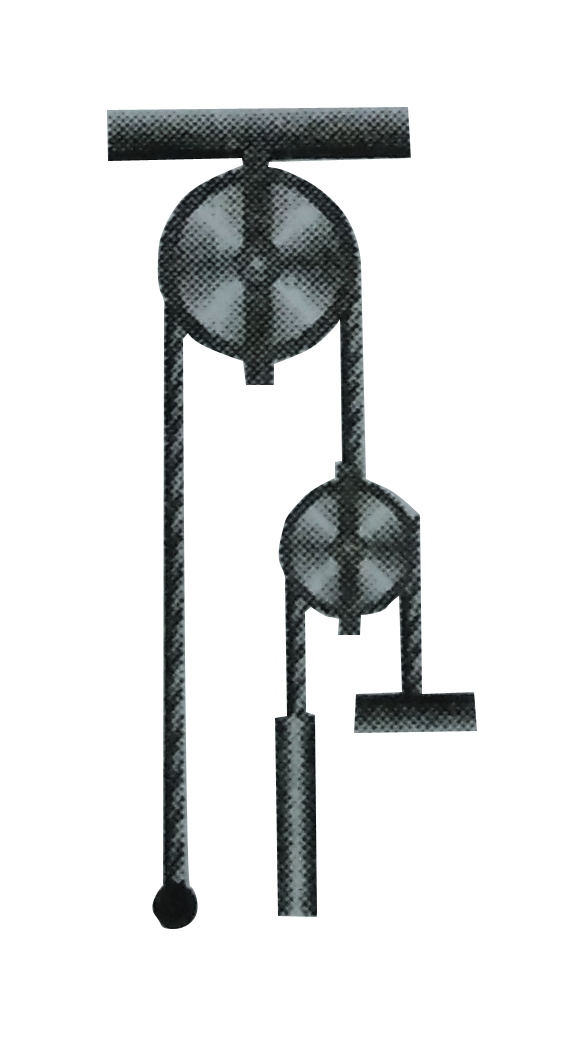
View SolutionIn the arrangement shown in figure the mass of the ball is η times as that of the rod. The length of the rod is L the masses of the pulleys and the threads as well as the friction, are negligible. The ball is set on the same level as the lower end of the rod and then released. How soon will the ball be opposite the upper end of the rod?
View SolutionA uniform rod of mass m=2kg and length l is kept on a smooth horizontal plane. If the ends A and B of the rod move with speeds v and 2v respectively perpendicular to the rod, find the:
a. angulr velocity of CM of the rod.
b. Kinetic energy of the rod (in Joule).

View SolutionIn a steady state of thermal conduction, temperature of the ends A and B of a 20 cm long rod are 100∘C and 0∘C respectively. What will be the temperature of the rod at a point at a distance of 9 cm from the end A of the rod
View Solution
