A slender rod of mass M and length L rests on a horizontal frictionless surface. The rod is pivoted about one of ends. The impulse of the force exerted on the rod by the pivot when the rod is struck by a blow of impulse J perpendicular to the rod at other end is
J
J2
J3
Information is insufficient
The correct Answer is:B
To solve the problem, we will analyze the situation step by step, focusing on the impulse applied to the rod and the resulting motion.
1. Understanding the System:
- We have a slender rod of mass M and length L resting on a frictionless horizontal surface.
- The rod is pivoted at one end, and an impulse J is applied perpendicular to the rod at the other end.
2. Impulse and Momentum:
- When the impulse J is applied, it will cause a change in momentum of the rod.
- The initial momentum of the rod is zero (since it is at rest). After the impulse is applied, the final momentum will be J.
3. Linear Momentum Equation:
- The change in linear momentum can be expressed as:
J=Δp=pfinal−pinitial=mvcm−0
- Therefore, the velocity of the center of mass vcm can be expressed as:
vcm=JM
4. Angular Momentum Consideration:
- The impulse J also creates an angular momentum about the pivot point.
- The torque τ due to the impulse J is given by:
τ=J⋅L
- The angular impulse is equal to the change in angular momentum:
J⋅L=I⋅ω
- The moment of inertia I of the rod about the pivot point is:
I=13ML2
5. Relating Angular Velocity and Linear Velocity:
- The angular velocity ω can be related to the linear velocity of the center of mass:
vcm=ω⋅L2
- Substituting vcm from step 3:
JM=ω⋅L2
- Rearranging gives:
ω=2JML
6. Using Angular Momentum Equation:
- Substitute ω back into the angular momentum equation:
J⋅L=13ML2⋅2JML
- Simplifying this gives:
J⋅L=23JL
- This implies:
J=23J
7. Impulse by the Pivot:
- The impulse J0 exerted by the pivot can be found by considering the net impulse:
J−J0=0⇒J0=12J
- Thus, the impulse of the force exerted on the rod by the pivot is:
J0=−J2
- The negative sign indicates that the direction of the impulse by the pivot is opposite to the direction of the applied impulse J.
Final Answer:
The impulse of the force exerted on the rod by the pivot when the rod is struck by a blow of impulse J is −J2.
- We have a slender rod of mass M and length L resting on a frictionless horizontal surface.
- The rod is pivoted at one end, and an impulse J is applied perpendicular to the rod at the other end.
- When the impulse J is applied, it will cause a change in momentum of the rod.
- The initial momentum of the rod is zero (since it is at rest). After the impulse is applied, the final momentum will be J.
- The change in linear momentum can be expressed as:
J=Δp=pfinal−pinitial=mvcm−0
- Therefore, the velocity of the center of mass vcm can be expressed as:
vcm=JM
- The impulse J also creates an angular momentum about the pivot point.
- The torque τ due to the impulse J is given by:
τ=J⋅L
- The angular impulse is equal to the change in angular momentum:
J⋅L=I⋅ω
- The moment of inertia I of the rod about the pivot point is:
I=13ML2
- The angular velocity ω can be related to the linear velocity of the center of mass:
vcm=ω⋅L2
- Substituting vcm from step 3:
JM=ω⋅L2
- Rearranging gives:
ω=2JML
- Substitute ω back into the angular momentum equation:
J⋅L=13ML2⋅2JML
- Simplifying this gives:
J⋅L=23JL
- This implies:
J=23J
- The impulse J0 exerted by the pivot can be found by considering the net impulse:
J−J0=0⇒J0=12J
- Thus, the impulse of the force exerted on the rod by the pivot is:
J0=−J2
- The negative sign indicates that the direction of the impulse by the pivot is opposite to the direction of the applied impulse J.
The impulse of the force exerted on the rod by the pivot when the rod is struck by a blow of impulse J is −J2.
Topper's Solved these Questions
- RIGID BODY DYNAMICS 2Book:CENGAGE PHYSICS ENGLISHChapter:RIGID BODY DYNAMICS 2Exercise:Multiple Correct
Explore 26 Videos
- RIGID BODY DYNAMICS 2Book:CENGAGE PHYSICS ENGLISHChapter:RIGID BODY DYNAMICS 2Exercise:Linked Comprehension
Explore 71 Videos
- RIGID BODY DYNAMICS 2Book:CENGAGE PHYSICS ENGLISHChapter:RIGID BODY DYNAMICS 2Exercise:Subjective
Explore 19 Videos
- RIGID BODY DYNAMICS 1Book:CENGAGE PHYSICS ENGLISHChapter:RIGID BODY DYNAMICS 1Exercise:Integer
Explore 11 Videos
- SOUND WAVES AND DOPPLER EFFECTBook:CENGAGE PHYSICS ENGLISHChapter:SOUND WAVES AND DOPPLER EFFECTExercise:Integer
Explore 16 Videos
Similar Questions
A uniform rod AB of mass m and length l at rest on a smooth horizontal surface . An impulse P is applied to the end B. The time taken by the rod to turn through a right angle is :

View SolutionA uniform rod AB of mass m and length l at rest on a smooth horizontal surface . An impulse P is applied to the end B. The time taken by the rod to turn through a right angle is :

View SolutionA uniform rod of length L rests on a frictionless horizontal surface. The rod is pivoted about a fixed frictionless axis at one end. The rod is initially at rest. A bulled travelling parallel to the horizontal surface and perpendicular to the rod with speed v strikes the rod at its centre and becomes embedded in it. the mass of the bullet is one-sixth the mass of the rod.
(a). What is the final angular velocity of the rod?
(b). What is the ratio of the kinetic energy of the system after the collision to the kinetic energy of te bullet the o collision?
View SolutionA uniform rod of mass M and length L lies flat on a frictionless horizantal surface. Two forces F and 2F are applied along the length of the rod as shown. The tension in the rod at point P is

View SolutionA slender rod of mass m and length L is pivoted about a horizontal axis through one end and released from rest at an angle of 30∘ above the horizontal. The force exerted by the pivot on the rod at the instant when the rod passes through a horizontal position is

View SolutionA rod of mass m and length L is kept on a horizontal smooth surface. The rod is hinged at its one end A. There are two springs, perpendicular to the rod, kept in the same horizontal plane. The spring of spring constant k is rigidly attached to the lower end of rod and spring of spring constant 12k just touches the rod at its midpoint C, as shown in the figure. If the rod is slightly displaced leftward and released then, the time period for small osciallation of the rod will be

View SolutionThe adjacent figure shows a non-uniform metre stick AB having the linear mass density variation λ=λ0x , where λ0 is a constant and x is the distance from end A, placed on a smooth horizontal surface. In the first experiment, the rod is pivoted at A and force F is applied perpendicular to the rod at the other end B and in the second experiment, the rod is pivoted at B and the force is applied perpendicular to the rod at the end A. If in the first case angular acceleration is αA and in the second case it is αB then
View SolutionA rod AB of mass m and length L is rotating in horizontal plane about one of its ends which is pivoted. The constant angular speed with which the rod is rotating is ω. The force applied on the rod by the pivot is
View SolutionA uniform rod of mass m, length l rests on a smooth horizontal surface. Rod is given a sharp horizontal impulse p perpendicular to the rod at a distance l/4 from the centre. The angular velocity of the rod will be
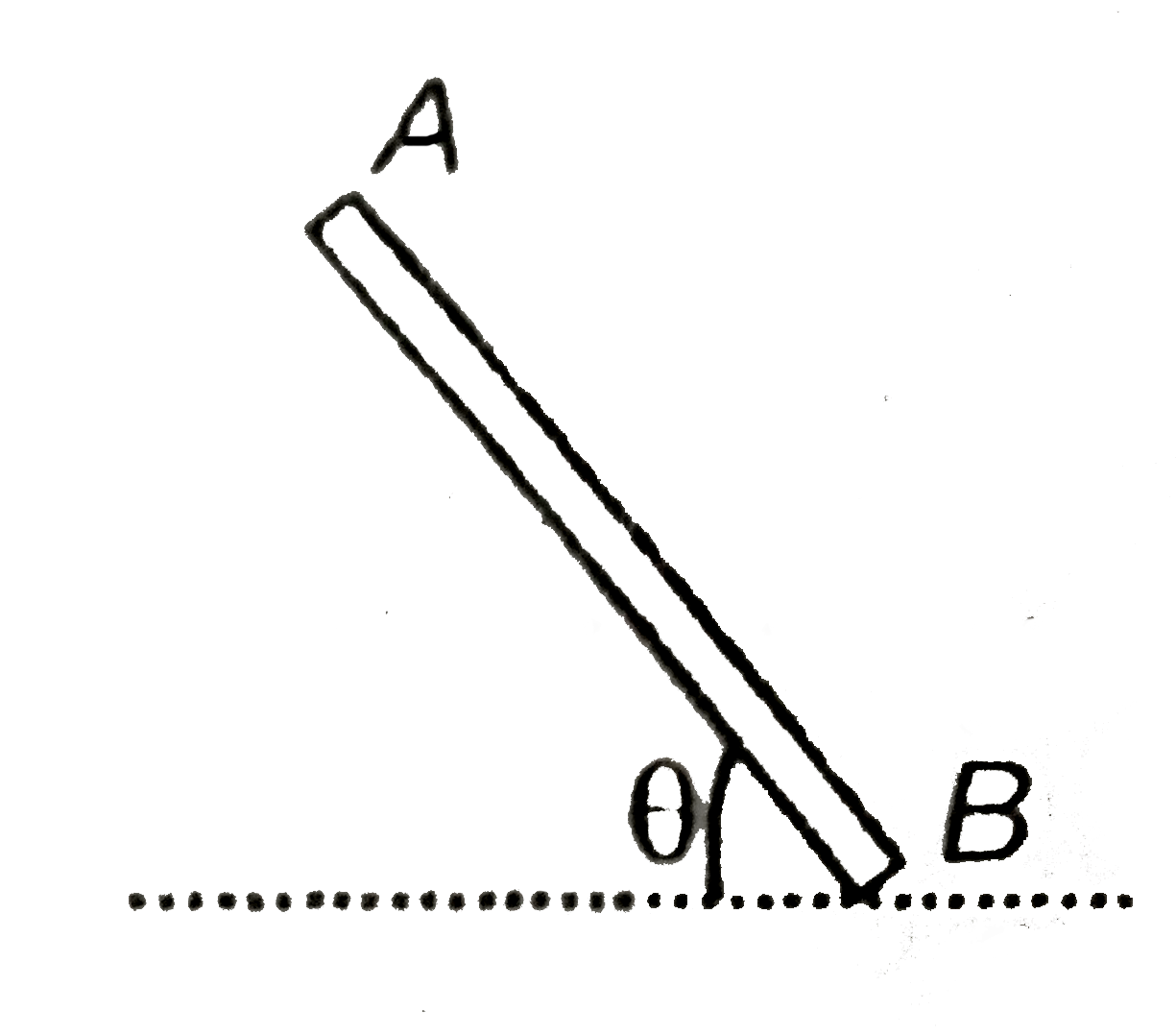
View SolutionA rod AB of mass M and length L is shown in figure. End A of rod is hinged and end B is lying on the ground. Find the Normal reaction by ground on the rod
View Solution