In Fig. the velocities are in ground frame and the cylinder is performing pure rolling on the plank, velocity of point 'A' would be
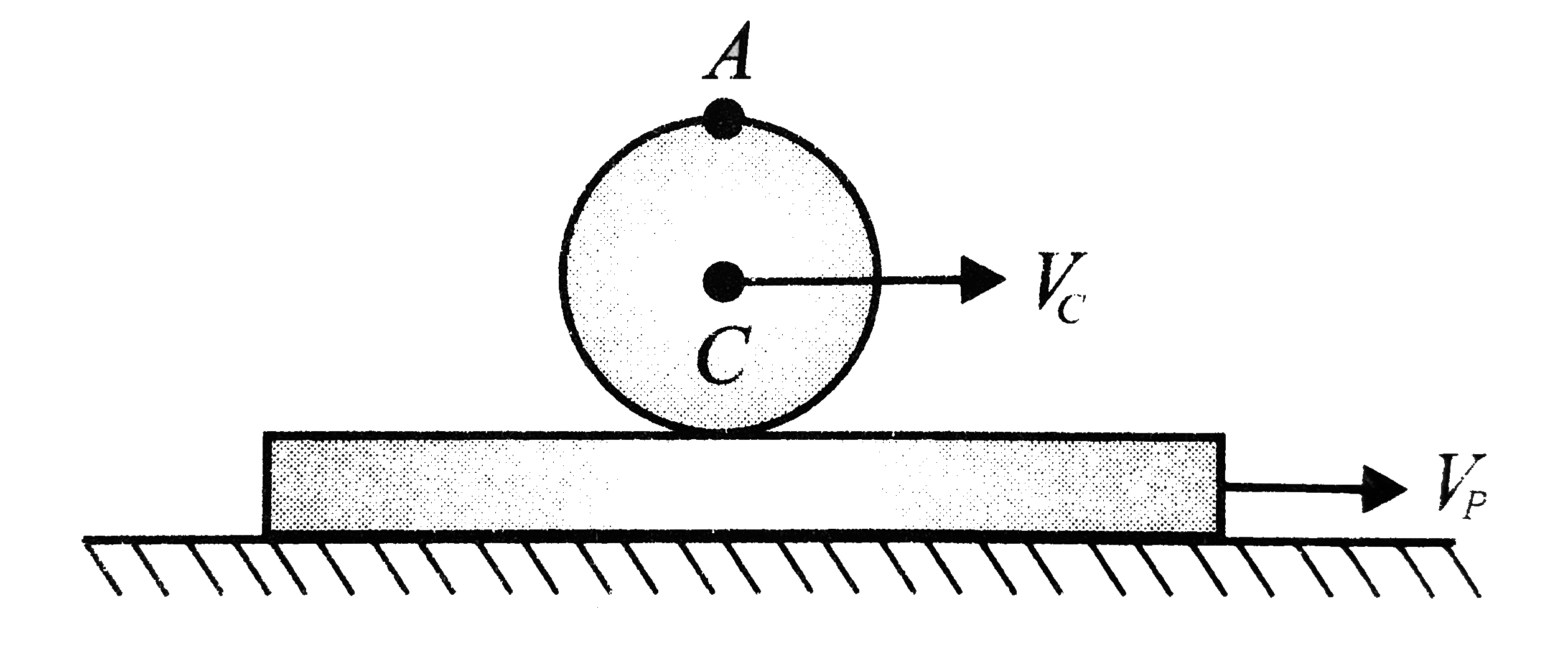
2VC
2VC+VP
2VC+VP
2(VC−VP)
The correct Answer is:C
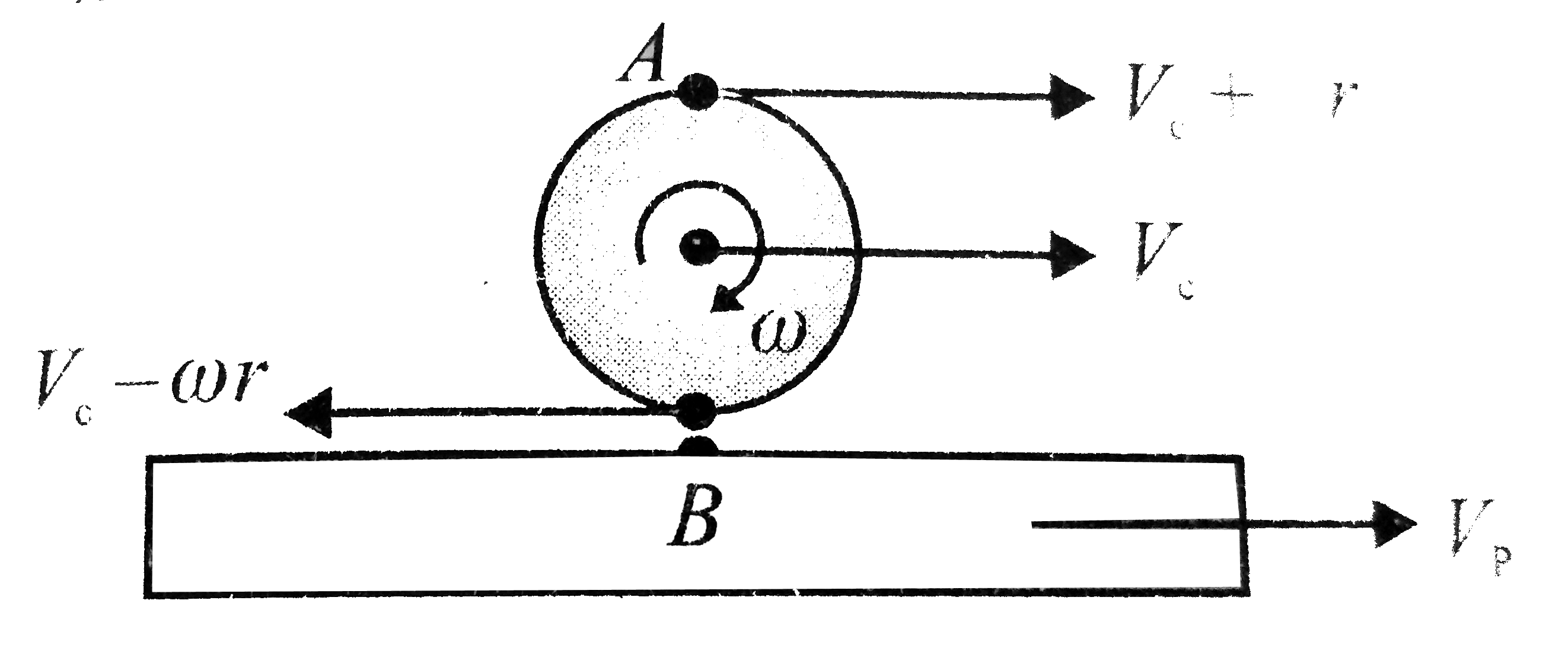
For pure rolling velocity of te point of contact as to be equal to the velocity of the surface.
Let us say cylinder rolls with angular velocity ω.
At point B,
VC−ωr=VP⇒ωr=VC−VP
At point A
VA=VC+ωr=2VC−VP
Topper's Solved these Questions
- RIGID BODY DYNAMICS 2Book:CENGAGE PHYSICS ENGLISHChapter:RIGID BODY DYNAMICS 2Exercise:Multiple Correct
Explore 26 Videos
- RIGID BODY DYNAMICS 2Book:CENGAGE PHYSICS ENGLISHChapter:RIGID BODY DYNAMICS 2Exercise:Linked Comprehension
Explore 71 Videos
- RIGID BODY DYNAMICS 2Book:CENGAGE PHYSICS ENGLISHChapter:RIGID BODY DYNAMICS 2Exercise:Subjective
Explore 19 Videos
- RIGID BODY DYNAMICS 1Book:CENGAGE PHYSICS ENGLISHChapter:RIGID BODY DYNAMICS 1Exercise:Integer
Explore 11 Videos
- SOUND WAVES AND DOPPLER EFFECTBook:CENGAGE PHYSICS ENGLISHChapter:SOUND WAVES AND DOPPLER EFFECTExercise:Integer
Explore 16 Videos
Similar Questions
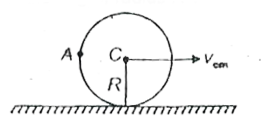
In case of pure rolling what will be velocity of point A of the ring of radius R ?
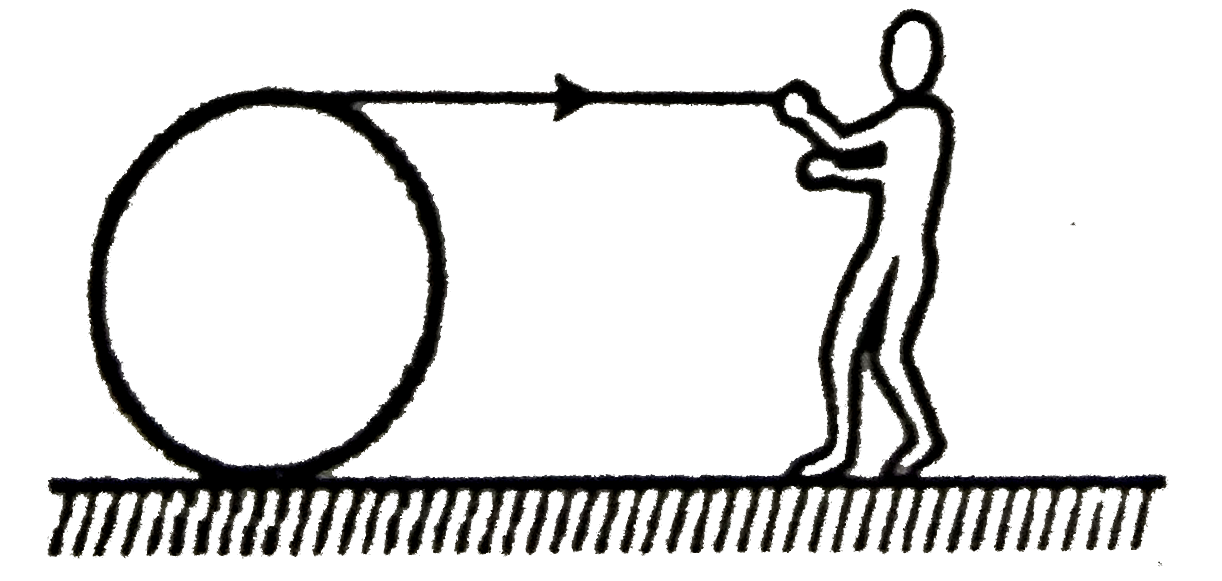
View SolutionA man pulls a solid cylinder ( initially at rest ) horizontal by a massless string. The string is wrapped on the cylinder and the cylinder performs pure rolling. Mass of the cylinder is 100kg, radius is π metre & tension in string is 100N. Then the angular speed of the cylinder after one revolution will be :
View Solution
Knowledge Check
A system of identical cylinders and plates is shown in Fig. All the cylinders are identical and there is no slipping at any contact. The velocity of lower and upper plates are V and 2V, respectively, as shown in Fig. Then the ratio of angular speeds of the upper cylinders to lower cylinders is
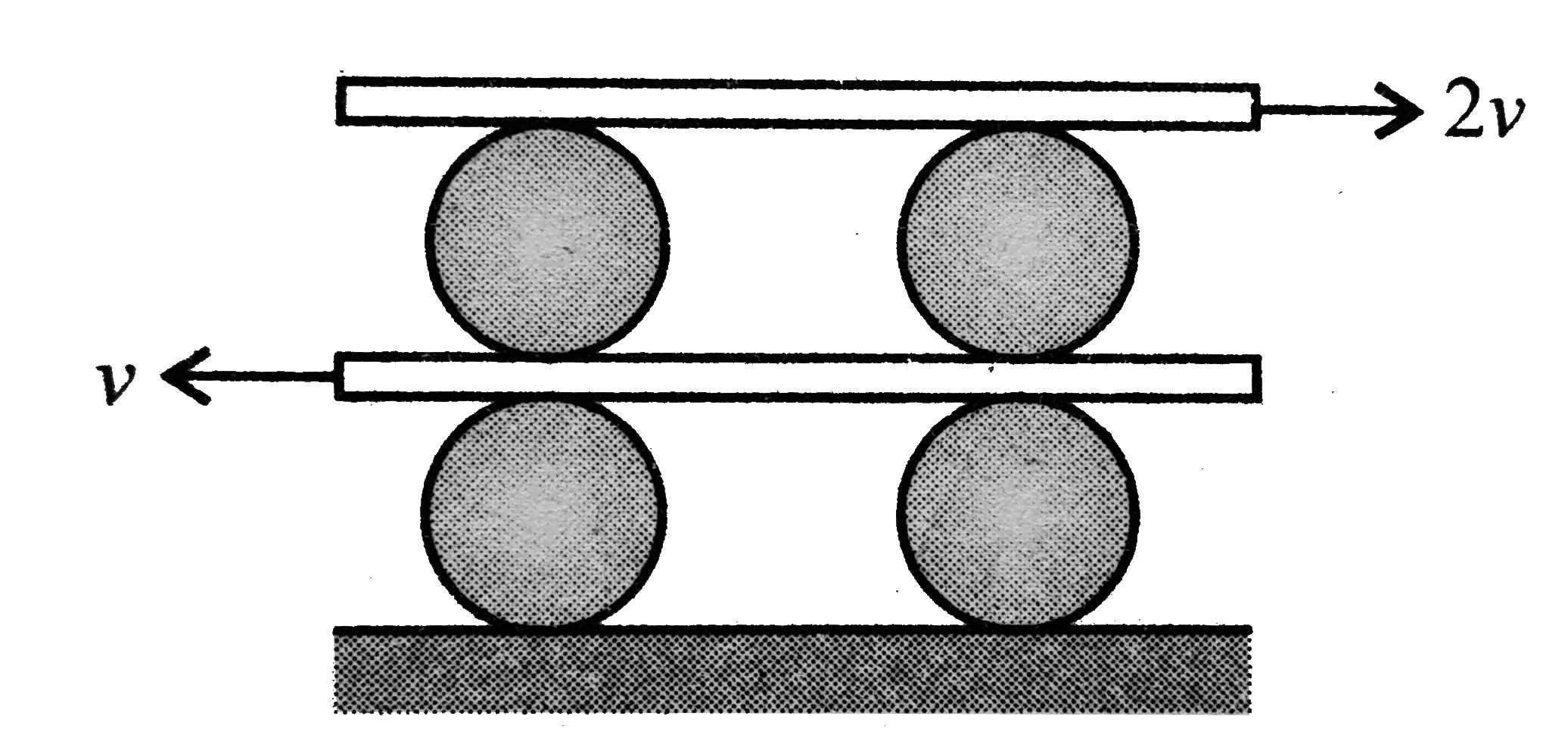 A1:3B3:1C1:2D2:1
A1:3B3:1C1:2D2:1
If v2,v2, and v3 are the velocities of points P,C and Q respectively lying on body doing pure rolling motion, then correct order of velocities will be
View SolutionA very large plank P of some unknown mass is being moved with velocity v0ˆi under application of an external force (not shown in figure). Simultaneously a block B of mass m placed on the plank is also moving with velocity v0ˆk. All these velocities are with respect to ground frame and at t=0. Coefficient of frictioin between the plank and the block μ. Choose the correct option(s).

View SolutionIn the figure shown, a solid sphere of mass 'm' and radius r is released from a height 6r to slide down a smooth surface. A plank of same mass 'm' touches the horizontal portion of the surface at the ground. The co-efficient of friction between the plank and the sphere is μ and that between the plank and the ground is μ/4. Find the work done by the friction force between the plank and the ground till the sphere starts pure rolling on the plank. Neglect the height of the plank.
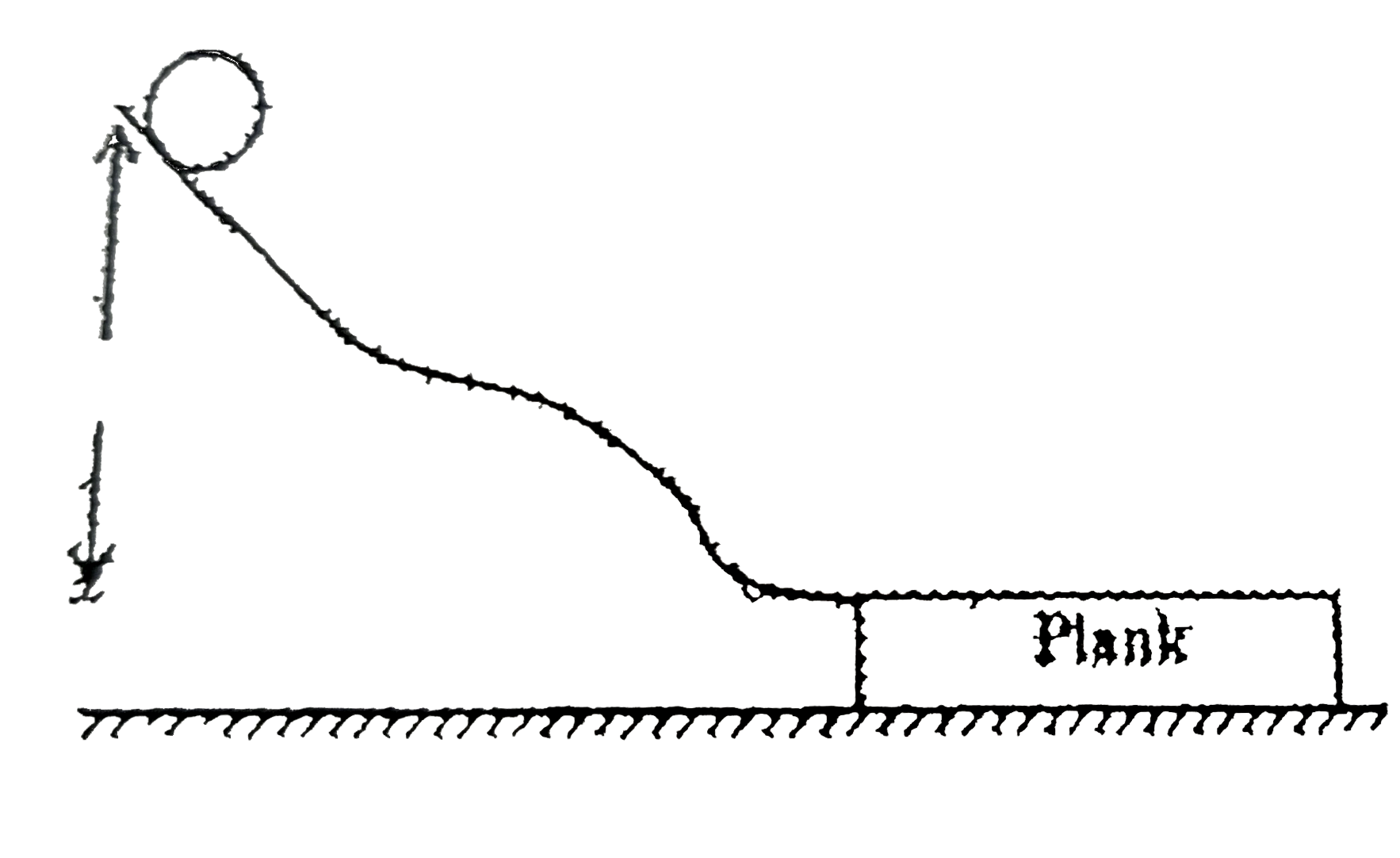
View SolutionA bullet of mass m moving with a velocity of u just grazes the top of a solid cylinder of mass M and radius R resting on a rough horizontal surface as shown and is embedded in the cylinder after impact. Assuming that the cylinder rolls without slipping, find the angular velocity of the cylinder and the final velocity of the bullet.

View SolutionA system of identical cylinders and plates is shown in Fig. All the cylinders are identical and there is no slipping at any contact. The velocity of lower and upper plates are V and 2V, respectively, as shown in Fig. Then the ratio of angular speeds of the upper cylinders to lower cylinders is

View SolutionConsider a cylinder rolling on a horizontal plane. Its linear velocity is v and rotational angular velocity is ω. Find its angular momentum about point P on the ground as shown in Fig. What happens to this angular momentum if the cylinder is rotating in opposite direction but moving translationally in the same direction.

View SolutionA system is shown in fig. Assume that the cylinder remains in contact with the two wedge. Then the velocity of cylinder is
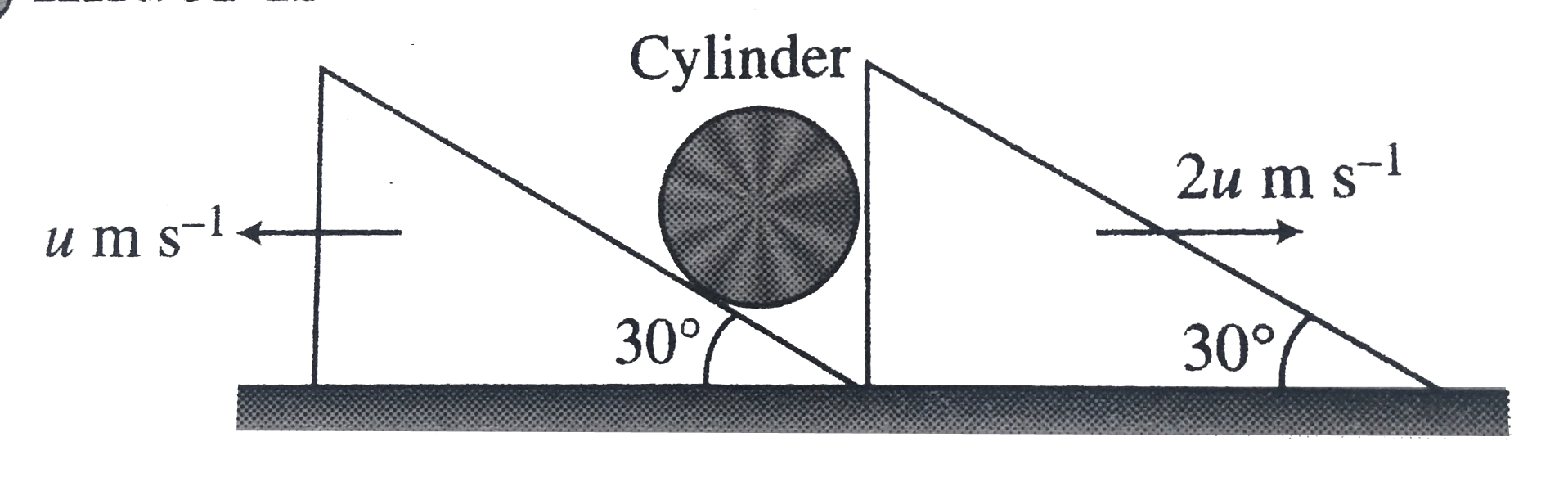
View Solution