A tall block of mass M=50kg and base width b=1m and height h=3m is kept on rough inclined surface with coefficient of friction μ=0.8 as shown in figure. The angle of inclination with the horizontal is 37∘. Determine whether the block slides down or topples over.
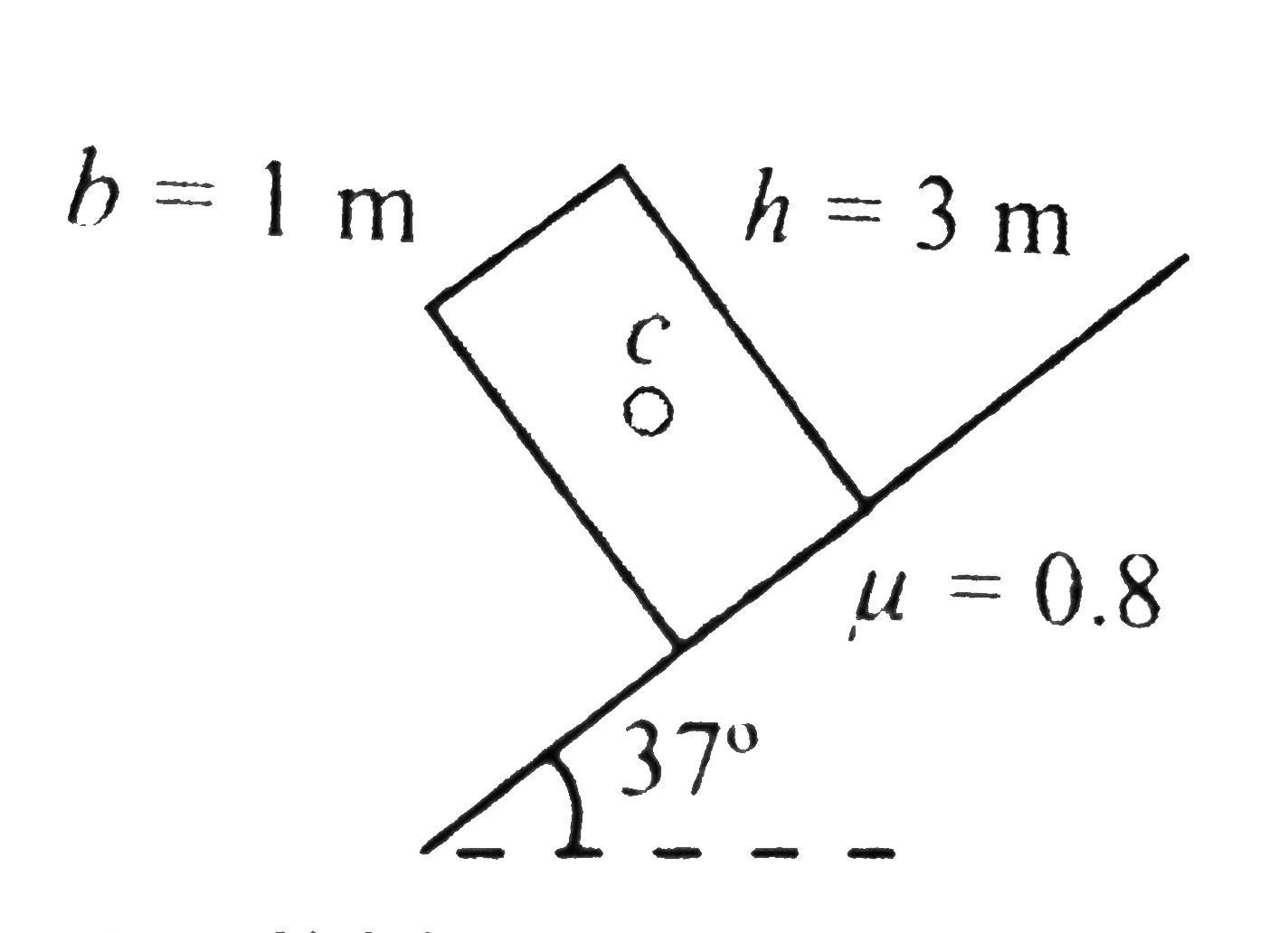
Here μ=0.8,bh=13,=0.33tanθ=34=0.75

Since μ>b/h and μ>tanθ, therefore the block has a greater tendency to topple over. From the condition of translation equilibrium , we have
N=Mgcos37∘=(50)(10)(45) or N=400N
f=Mgcos37∘=(50)(10)(35)
=300N<fmass=μN=0.8(400)=320N
For rotational equilibrium Στc=0
Nx=fh2
Thus, x=fNh2=(300400)(32)=98<b2
Since the value of x is more than b/2 which is not possible, therefore the block topples over.
Topper's Solved these Questions
- RIGID BODY DYNAMICS 1Book:CENGAGE PHYSICS ENGLISHChapter:RIGID BODY DYNAMICS 1Exercise:Solved Examples
Explore 9 Videos
- RIGID BODY DYNAMICS 1Book:CENGAGE PHYSICS ENGLISHChapter:RIGID BODY DYNAMICS 1Exercise:Exercise 2.1
Explore 6 Videos
- PROPERTIES OF SOLIDS AND FLUIDSBook:CENGAGE PHYSICS ENGLISHChapter:PROPERTIES OF SOLIDS AND FLUIDSExercise:INTEGER_TYPE
Explore 2 Videos
- RIGID BODY DYNAMICS 2Book:CENGAGE PHYSICS ENGLISHChapter:RIGID BODY DYNAMICS 2Exercise:Interger
Explore 2 Videos
Similar Questions
A block with a square base measuring a×a and height h, is placed on an inclined plane. The coefficient of friction is μ. The angle of inclination (θ) of the plane is gradually increased. The block will
View SolutionA block of mass m is pulled on an incline surface having coefficient of friction μ=1& angle of inclination θ=30∘, with the horizontal, such that required external force is minimum. The angle made by this force with the incline is :
View Solution
Knowledge Check
A block of mass m rests on a rough inclined plane. The coefficient of friction between the surface and the block is µ. At what angle of inclination θ of the plane to the horizontal will the block just start to slide down the plane?
Aθ=tan−1μBθ=cos−1μCθ=sin−1μDθ=sec−1μA block of mass m is at rest on an inclined plane. The coefficient of static friction is μ. The maximum angle of incline before the block begins to slide down is :
Atan−1(μ/m)Btan−1(μ)Ctan−1(μ/2)Dcos−1(μ)A track has two inclined surface AB and DC each of length 3 m and angle of inclination of 30∘ with the horizontal and a central horizontal part of length 4m shown in figure. A block of mass 0.2 kg slides from rest from point A. The inclined surfaces are frictionless. If the coefficient of friction between the block and the horizontal flat surface is 0.2, where will the block finally come to rest ? [in 10−1m]
 A0.5 m away from point BB3.5 m away from point BC0.5 m away from point CD1.5 m away from point C
A0.5 m away from point BB3.5 m away from point BC0.5 m away from point CD1.5 m away from point C
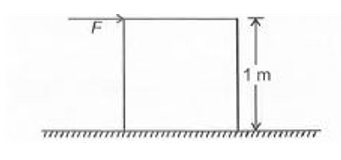
A cubical block of edge length 1 m and density 800 kg m−3 rests on a rough horizontal surface with coefficient of friction μ. A horizontal force F is applied on the block as shown in figure. If the coefficient of friction is sufficiently large so that the block does not slide bofore toppling, then the minimum value of F to topple the block is [Take g = 10 ms−2]
View SolutionA block of mass m moves with constant speed down the inclined plane of inclination θ. Find the coefficient of kinetic friction.

View SolutionA block of mass m=3kg slides on a rough inclined plane of cofficient of friction 0.2 Find the resultant force offered by the plane on the block

View SolutionA block is kept on a rough horizontal surface as shown. Its mass is 2 kg and coefficient of friction between block and surface (μ)=0.5. A horizontal force F is acting on the block. When

View SolutionA block of mass 10 kg is kept on a fixed rough (μ=0.8) inclined plane of angle of inclination 30∘. The frictional force acting on the block is

View Solution