A cube of side a is placed on an inclined plane of inclination $θ$ . What is the maximum value of $θ$ for which the cube will not topple?

Video Solution

Text Solution

Verified by Experts

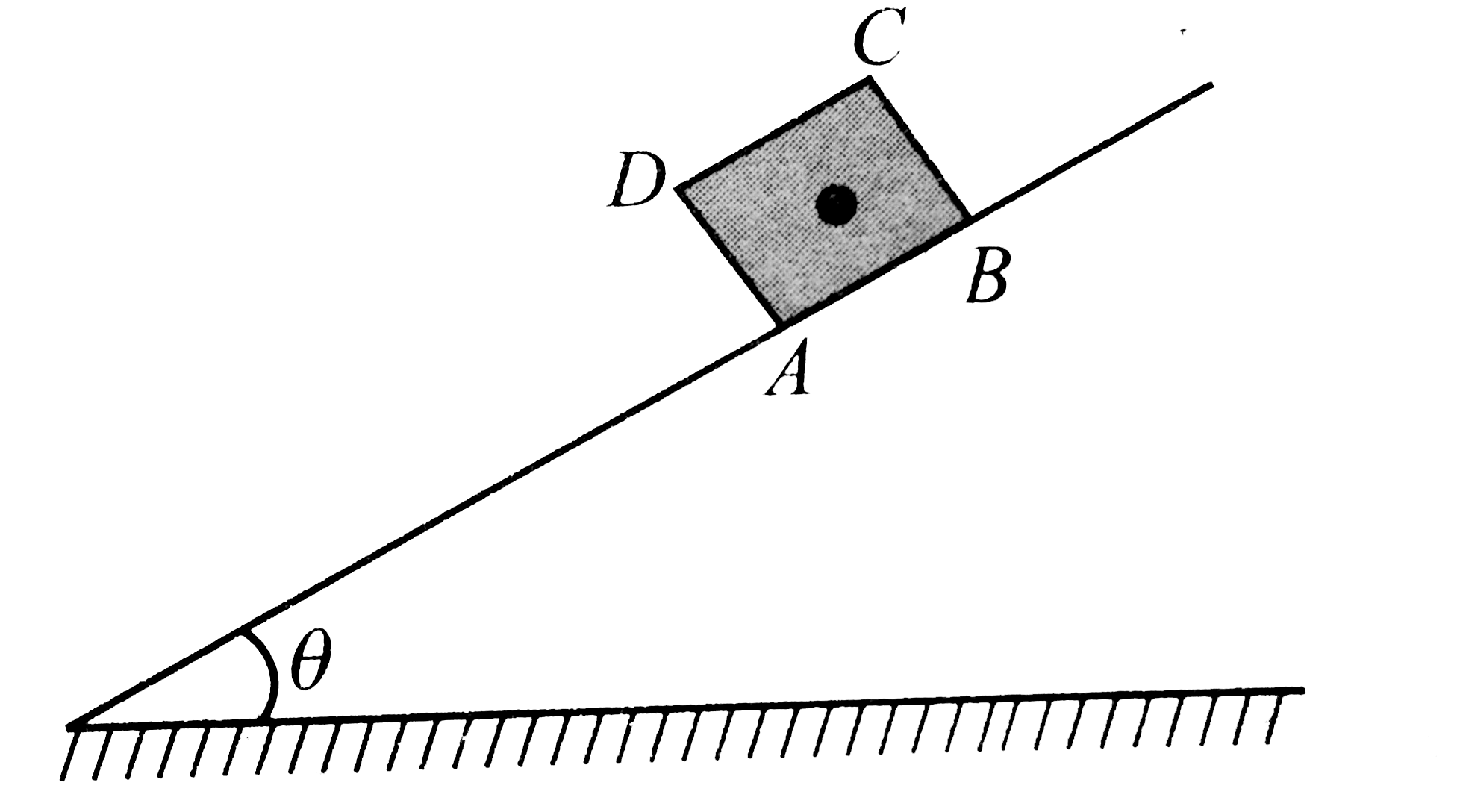
a. The free body diagram of the block is shown in figure.

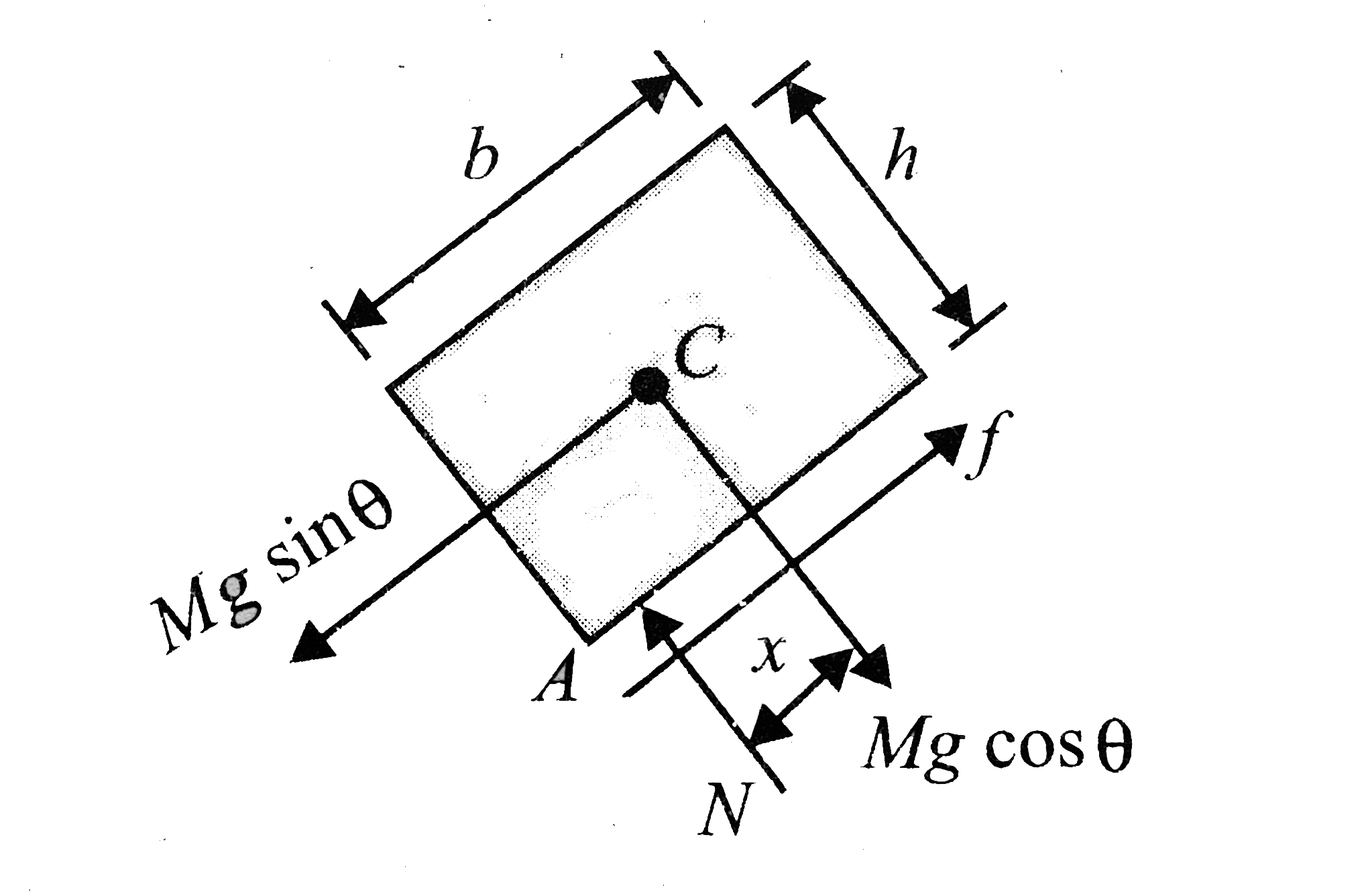
Note that the point of application of the normal reaction $N$ is displaced through $x$ in the downward direction. It has to porduce a clockwise torque about the centre of mass that may balance the anticlockwise torque produced by the friction force.
There are two tendencies of the block: to slide down
to rotate (or topple) about the point $A$
For translation equation $\sum \to F = 0$
$f = M g sin θ$ .......i
$N = m g cos θ$ ...........ii
For rotational equilibrium $\sum {\to τ}_{C} = 0$
$N \times x = f \times \frac{h}{2} \Rightarrow M g cos θ \times x = \frac{f h}{2}$
$\Rightarrow f = \frac{2 M g cos θ \times x}{h}$
If the block topples over about $A, x = \frac{b}{2}$
Hence, $\frac{b M g cos θ}{h}$ ......iii
From eqn if block do not slide down $f < μ N$
$M g sin θ, μ y \times M g cos θ \Rightarrow tan θ \leq μ$
or we can say if the block $μ < tan θ$
from eqn iii if the block topples before sliding,
$f \leq μ (M g cos θ)$
$\frac{b M g cos θ}{h} \leq μ M g cos θ \Rightarrow \frac{b}{h} \leq μ$
Hence if the block topples before sliding $μ \geq \frac{b}{h}$

|

Updated on:21/07/2023

Class 11 PHYSICS RIGID BODY DYNAMICS

Topper's Solved these Questions

Similar Questions

CENGAGE PHYSICS ENGLISH-RIGID BODY DYNAMICS 1-Integer

Home
Ask Doubt
Profile