Find the out the moment of inertia of a ring having uniform mass distribution of mass M and radius R about an axis which is tangent ot the ring and a in the plane of the ring b. perpendicular to the plane of the ring.
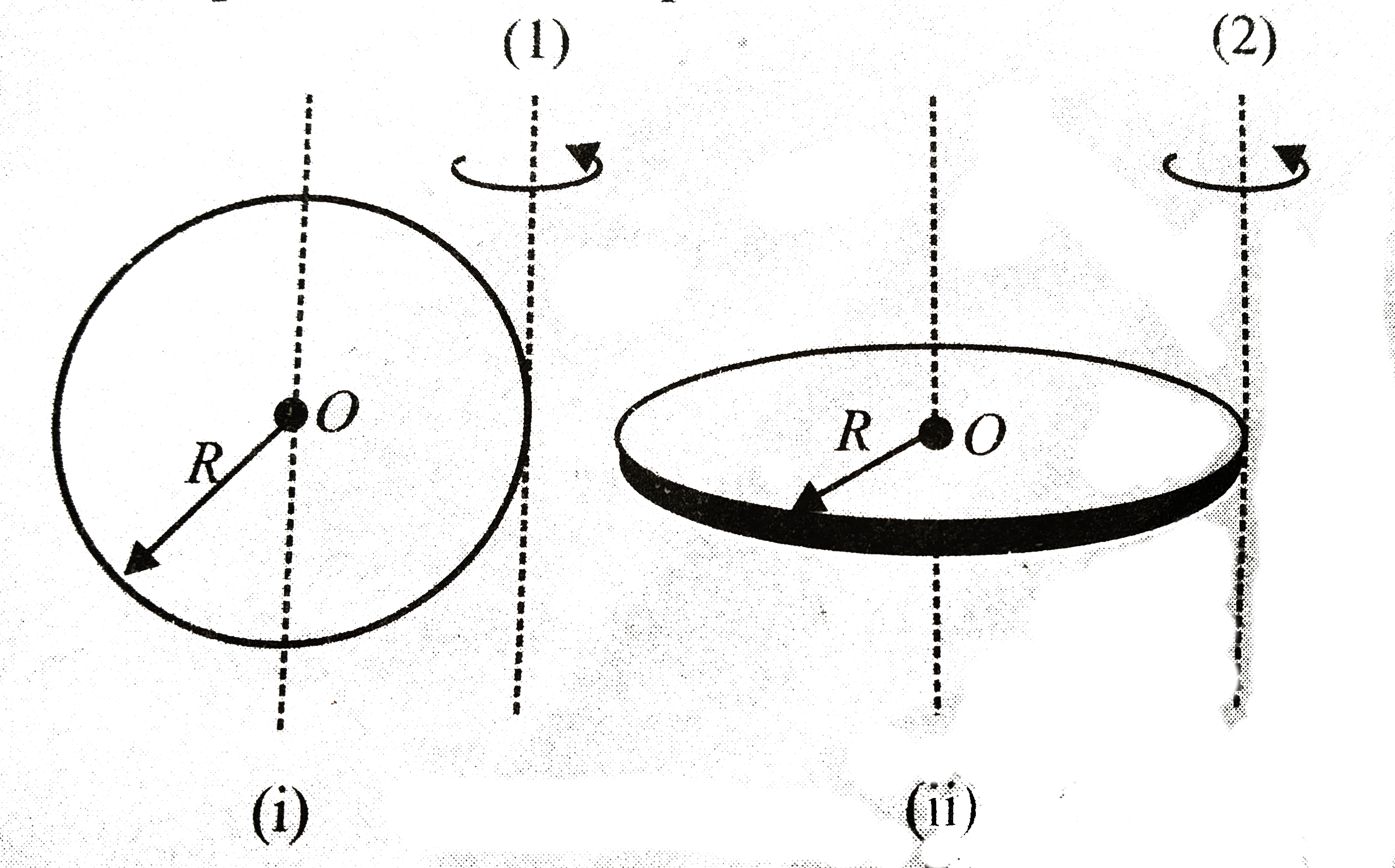
The moment of inertia of a ring about an axis passing through centre and perpendicular to plain of ring is Ix=Iy and using perpendicular axis theorem.

Iz=Ix+Iy⇒MR2=2Ix
⇒Ix=MR22=I0
For case a using parallel axis theorem
I1=I0+MR22+MR2=32MR2
For case b using parallel axis theorem,
I2=Iz+MR2=MR2+MR2=2MR2
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 1EXERCISE - Solved Examples9videos
RIGID BODY DYNAMICS 1
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 1EXERCISE - Exercise 2.16videos
RIGID BODY DYNAMICS 1
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - PROPERTIES OF SOLIDS AND FLUIDSEXERCISE - INTEGER_TYPE2videos
PROPERTIES OF SOLIDS AND FLUIDS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Interger2videos
RIGID BODY DYNAMICS 2
Similar Questions
Calculate the moment of inertia of a ring having mass M, radius Rand having uniform mass distribution about an axis passing through the centre of the ring and perpendicular to the plane of the ring?
 View Solution
View SolutionThe moment of inertia of a ring of mass M and radius R about PQ axis will be
 View Solution
View SolutionFind the moment of inertia, about a diameter, of a uniform ring of mass M = 8kg and radius a = 1m?
 View Solution
View SolutionFind the moment of inertia of a uniform ring of mass M and radius R about a diameter.
View SolutionFor a uniform ring of mass M and radius R at its centre
View SolutionMoment of inertia of a ring of mass M and radius R about an axis passing through the centre and perpendicular to the plane is
View SolutionCalculate the moment of inertia of
a. a ring of mass M and radius R about an axis coinciding with the diameter of the ring.
b. as thin disc about an axis coinciding with the diameter.View SolutionFind out the moment of inertia of the circular arcs shown, each having mass M, radius R and having uniform mass distribution about an axis passing through the centre and perpendicular to the plane ?
 View Solution
View SolutionWhat is the moment of inertia of a uniform circular ring about its diameters ?
View SolutionThe M.I. of a thin ring of mass M and radius R about an axis through the diameter in its plane will be:
View Solution
