A log of wood of length l and mass M is floating on the surface of a river perpendicular to the banks. One end of the log touches the banks. A man of mass m standing at the other end walks towards the bank. Calculate the displacement of the log when he reaches nearer end of the log
To solve the problem of the log floating on the river, we will analyze the situation step by step.
Step 1: Understand the System
We have a log of wood of length l and mass M floating on the surface of a river. One end of the log is touching the bank, and a man of mass m is standing at the other end of the log. As the man walks towards the bank, we need to find out how much the log will displace.
Hint: Visualize the log and the man’s position relative to the bank.
Step 2: Define the Center of Mass
We will consider the center of mass of the system (log + man). The initial position of the center of mass can be calculated as follows:
- The center of mass of the log is at l2 from the bank.
- The position of the man at the other end of the log is at l.
The initial center of mass xcm,initial can be calculated using the formula:
xcm,initial=M⋅l2+m⋅lM+m
Hint: Remember that the center of mass is a weighted average of the positions of the masses.
Step 3: Position After the Man Walks
When the man walks to the end of the log (which is now at the bank), the position of the man becomes 0 (since he is at the bank) and the position of the center of mass of the log remains at l2 from the bank.
Now, the final center of mass xcm,final can be expressed as:
xcm,final=M⋅l2+m⋅0M+m
Hint: Think about how the position of the man changes the center of mass.
Step 4: Set Initial and Final Center of Mass Equal
Since there are no external horizontal forces acting on the system, the center of mass of the system must remain in the same position. Therefore, we can set the initial and final center of mass equal to each other:
M⋅l2+m⋅lM+m=M⋅l2M+m
Hint: This step is crucial because it shows that the center of mass does not change due to the internal movement of the man.
Step 5: Solve for the Displacement of the Log
From the above equation, we can simplify to find the displacement x of the log when the man reaches the bank:
x=m⋅lM+m
This x represents the distance the log has moved away from the bank as the man walks towards it.
Hint: The displacement of the log is directly related to the ratio of the masses and the length of the log.
Final Answer
The displacement of the log when the man reaches the nearer end of the log is:
Displacement of the log=m⋅lM+m
We have a log of wood of length l and mass M floating on the surface of a river. One end of the log is touching the bank, and a man of mass m is standing at the other end of the log. As the man walks towards the bank, we need to find out how much the log will displace.
We will consider the center of mass of the system (log + man). The initial position of the center of mass can be calculated as follows:
- The center of mass of the log is at l2 from the bank.
- The position of the man at the other end of the log is at l.
xcm,initial=M⋅l2+m⋅lM+m
When the man walks to the end of the log (which is now at the bank), the position of the man becomes 0 (since he is at the bank) and the position of the center of mass of the log remains at l2 from the bank.
xcm,final=M⋅l2+m⋅0M+m
Since there are no external horizontal forces acting on the system, the center of mass of the system must remain in the same position. Therefore, we can set the initial and final center of mass equal to each other:
M⋅l2+m⋅lM+m=M⋅l2M+m
From the above equation, we can simplify to find the displacement x of the log when the man reaches the bank:
x=m⋅lM+m
The displacement of the log when the man reaches the nearer end of the log is:
Displacement of the log=m⋅lM+m
Topper's Solved these Questions
- CENTRE OF MASSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Solved Examples
13 Videos
- CENTRE OF MASSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Exercise 1.1
20 Videos
- CALORIMETRYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CALORIMETRYEXERCISE - Solved Example
13 Videos
- DIMENSIONS & MEASUREMENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Integer
2 Videos
Similar Questions
A chain of length l and mass m lies on the surface of a smooth sphere of radius R>l with one end tied to the top of the sphere.
View SolutionA plank of mas M and length L is at rest on as frictionless floor. The top surface of the plank has friction. At one end of it a man of mass m is standing as shown in figure. If the man walks towards the other end the find the distance, which the plank moves a till the man reaches the centre of the plank. b. till the man reaches the other end of the plank.

View SolutionA boat of length 10m and mass 450kg is floating without motion in still water. A man of mass 50kg standing at one end of it walks to the other end of it and stops. The magnitude of the displacement of the boat in metres relative to ground is
View SolutionA wooden plank of mass 20kg is resting on a smooth horizontal floor. A man of mass 60kg starts moving from one end of the plank to the other end. The length of the plank is 10m. Find the displacement of the plank over the floor when the man reaches the other end of the plank.

View SolutionThere is a rod of length l and mass m. It is hinged at one end to the ceiling. The period of small oscillation is
View SolutionA man of mass m is standing at one end of of a plank of mass M. The length of the plank is L and it rests on a frictionless horizontal ground. The man walks to the other end of the plank. Find displacement of the plank and man relative to the ground.

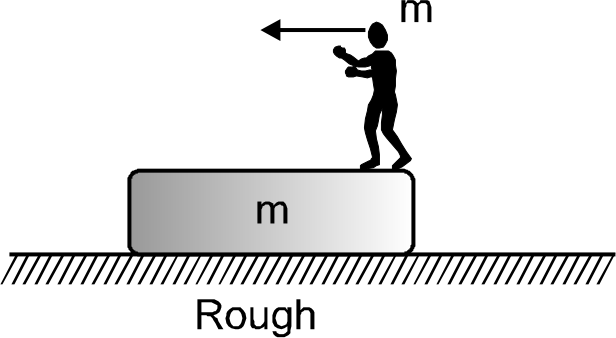
View SolutionA block of mass m and length l is kept at rest on a rough horizontal ground of friction coefficient μk. A man of mass m is standing at the right end. Now the man starts walking towards left and reaches the left end within time ‘t’. During this time, the displacement of the block is : (Assume the pressing force between the block and the ground remains constant and its value is same as it was initially. Also as sume that the block slides during the entire time (t))
View SolutionA uniform stick of length l and mass m lies on a smooth table. It rotates with angular velocity ω about an axis perpendicular to the table and through one end of the stick. The angular momentum of the stick about the end is
View SolutionA chain of length L and mass m is placed upon a smooth surface. The length of BA is (L−b). Calculate the velocity of the chain when its and reaches B
View SolutionA man standing on the bank of a river observes that the angle of elevation of a tree on the opposite bank is 60∘ . When he moves 50 m away from the bank, he finds the angle of elevation to be 30∘ . Calculate :
the width of the river and
View Solution
