A single conservative force acting on a particle varies as F=(−Ax+Bx2)ˆiN, where A and B are constants and x is in meters. (a) Calculate the potential-energy function U(x) associated with this force, taking U=0 at x=0.
(b) Find the change in potential energy and the change in kinetic energy as the particle moves from x=2.00m to x=3.00m.
a. U=−x∫0(−Ax+Bx2)dx=Ax22−Bx33
b. ΔU=ΔUx=3m−ΔUx=2m
=A[(3.002)−(2.00)2]2−B[(3.00)3−(2.00)3]3
=5.002A−19.03B
ΔK=−ΔU=(−5.002A+19.03B)
Topper's Solved these Questions
- WORK, POWER & ENERGYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WORK, POWER & ENERGYEXERCISE - Exercise 8.3
26 Videos
- WORK, POWER & ENERGYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WORK, POWER & ENERGYEXERCISE - Exercise 8.4
16 Videos
- WORK, POWER & ENERGYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WORK, POWER & ENERGYEXERCISE - Exercise 8.1
25 Videos
- VECTORSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - VECTORSEXERCISE - Exercise Multiple Correct
5 Videos
Similar Questions
A single conservative force acting on a particle varies as →F=(−Ax+Bx2)ˆiN, where A and B are constants and x is in meters. (a) Calculate the potential energy function U(x) associated with this force, taking U=0 at x=0. (b) Find the change in potential energy and the change in kinetic energy of the system as the particles moves from x=2.00m to x=3.00m.
View SolutionA particle free to move along x-axis is acted upon by a force F=−ax+bx2whrteaandbarepositiveconstants., the correct variation of potential energy function U(x) is best represented by.
View Solution
Knowledge Check
A particle is acted upon by a force F which varies with position x is shown in figure .If the particle at x=0 kinetic energy of 25J then the kinetic energy of the particle at x=16m is
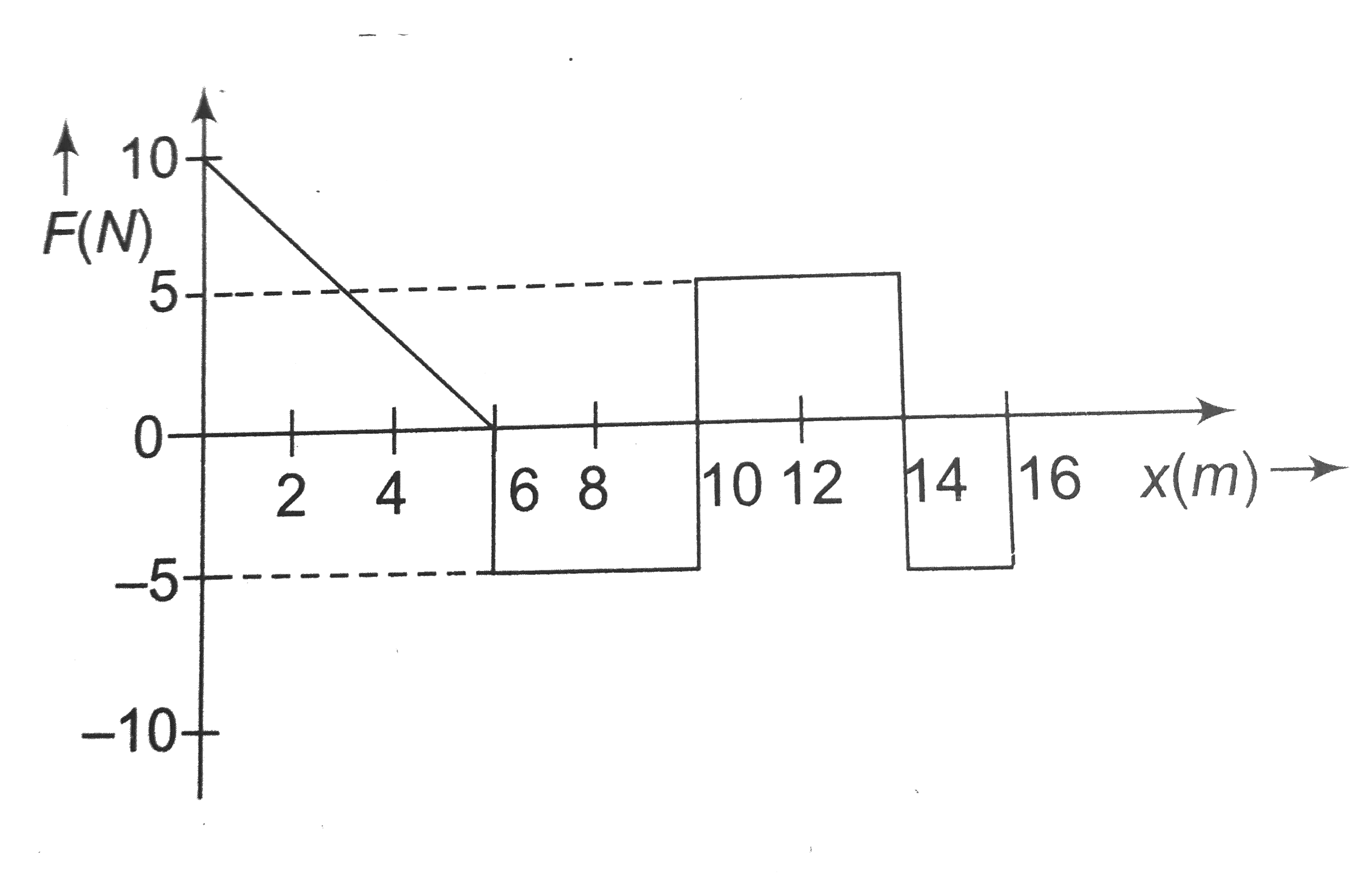 A45 JB30 JC70 JD20 J
A45 JB30 JC70 JD20 J
athe potential energy of a particle of mass 2kg moving along the x-axis is given by U(x)=4x2−2x3( where U is in joules and x is in meters). The kinetic energy of the particle is maximum at
View SolutionThe potential energy function of a particle is given by U(r)=A2r2−B3r, where A and B are constant and r is the radial distance from the centre of the force. Choose the correct option (s)
View SolutionOn a particle placed at origin a variable force F=−ax(where a is a positive constant) is applied. If U(0)=0, the graph between potential energy of particle U(x) and x is best represented by:-
View SolutionThe potential energy for a conservative force system is given by U=ax2−bx. Where a and b are constants find out (a) The expression of force (b) Potential energy at equilibrium.
View SolutionThe potential energy for a conservative force system is given by U=ax2−bx. Where a and b are constants find out (a) The expression of force (b) Potential energy at equilibrium.
View SolutionThe potential energy of a particle oscillating along x-axis is given as
U=20+(x−2)2
Here, U is in joules and x in meters. Total mechanical energy of the particle is 36J.
(a) State whether the motion of the particle is simple harmonic or not.
(b) Find the mean position.
(c) Find the maximum kinetic energy of the particle.
View SolutionA potential energy function for a two-dimensional force is the form U=3x2y−7x. Find the force that acts at the point (x,y).
View Solution
