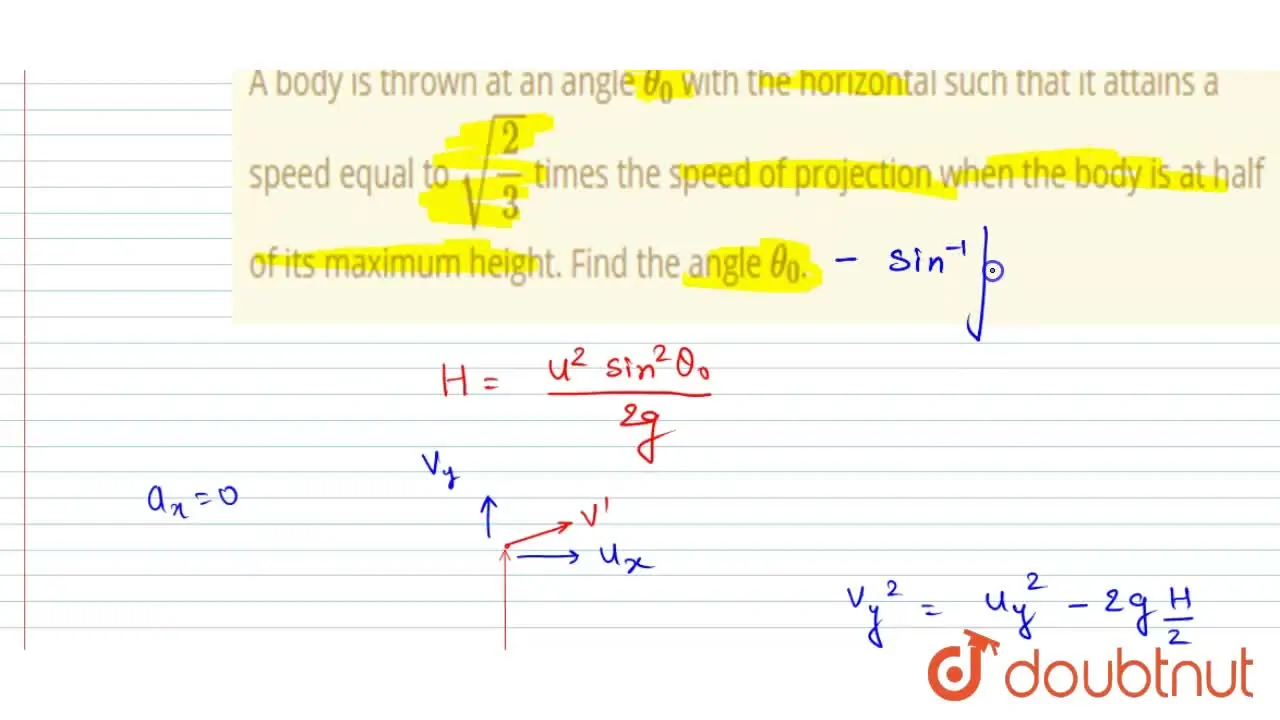
A body is thrown at an angle $θ_{0}$ with the horizontal such that it attains a speed equal to $\sqrt{\frac{2}{3}}$ times the speed of projection when the body is at half of its maximum height. Find the angle $θ_{0}$ .

A

$15^{\circ}$

B

$30^{\circ}$

C

$45^{\circ}$

D

$60^{\circ}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

(b) $v_{y}^{2} - v^{2} {sin}^{2} = - 2 g [\frac{1}{2} \frac{v^{2} {sin}^{2} θ}{2 g}]$
or $v_{y}^{2} = v^{2} {sin}^{2} θ - \frac{v^{2} {sin}^{2} θ}{2} or v_{y} = \frac{v sin θ}{\sqrt{2}}$
$v'^{2} = {(v cos θ)}^{2} + {(\frac{v sin θ}{\sqrt{2}})}^{2}$
= $v^{2} {cos}^{2} θ + \frac{v^{2} {sin}^{2} θ}{2} = v^{2} ({cos}^{2} θ + \frac{{sin}^{2} θ}{2})$
$v' = v \sqrt{{cos}^{2} θ + \frac{{sin}^{2} θ}{2}}$
$v^{2} {cos}^{2} θ = \frac{2}{5} v^{2} [{cos}^{2} θ + \frac{{sin}^{2} θ}{2}]$
or $5 {cos}^{2} θ = 2 {cos}^{2} θ + {sin}^{2} θ or 3 {cos}^{2} θ = {sin}^{2} θ$
or ${tan}^{2} θ = 3 or tan θ = \sqrt{3} or θ = 60^{\circ}$
Angle of projection with vertical is $90^{\circ} - 60^{\circ} = 30^{\circ}$ .

Updated on:21/07/2023

|

Class 11 PHYSICS PROJECTILE MOTION

Topper's Solved these Questions

Similar Questions

Knowledge Check

If a body is projected with an angle $θ$ to the horizontal, then
Aits velocity is always perpendicular to its acceleration then
Bits velocity becomes zero at its maximum height.
Cits velocity makes zero angle with the horizontal at its maximum height.
Dthe body just before hitting the ground, the direction of velocity coincides with the acceleration.

CENGAGE PHYSICS ENGLISH-KINEMATICS-2-Exercise Comprehension

Home
Ask Doubt
Profile