Find the average velocity of a projectile between the instants it crosses half the maximum height. It is projected with speed u at angle θ with the horizontal.
The correct Answer is:5m
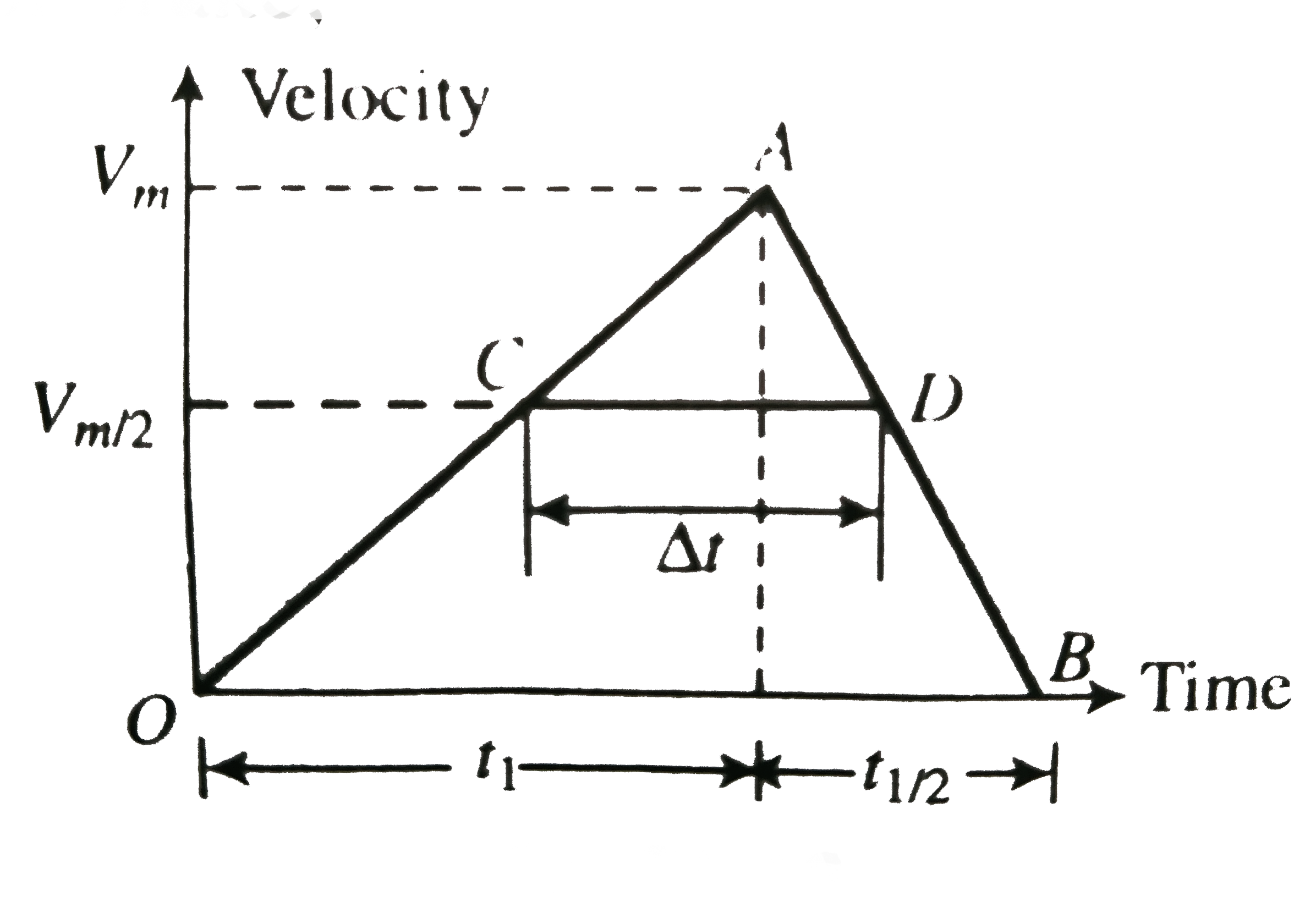
Velocity time curve of the process is shown. Acceleration before applecation of brake,
 .
.
a1=vmt1⇒t1=vma1 (i)
Displacement =(1/2)vm
(t1+t2/2)
But velocity (vm/2) is observed at C and D.
CD=Δ=Δt=1s (given)
C and D are mid-points of OA and OB .
From similar triangles, OB=(t1_t2/2)=2CD=2Δt
Displacement=(12)vm(2Δt)=vmΔt=5×1=5m,
Topper's Solved these Questions
- KINEMATICS-1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-1EXERCISE - Single Correct
52 Videos
- KINEMATICS-1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-1EXERCISE - Graphical Concept
17 Videos
- KINEMATICS-1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-1EXERCISE - Exercise 4.4
16 Videos
- GRAVITATIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GRAVITATIONEXERCISE - INTEGER_TYPE
1 Video
- KINEMATICS-2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-2EXERCISE - Exercise Integer
9 Videos
Similar Questions
A stone projected with a velocity u at an angle (theta )with the horizontal reaches maximum heights H1. When it is projected with velocity u at an angle (π2−θ) with the horizontal, it reaches maximum height H2. The relations between the horizontal range R of the projectile, H1andH2, is
View SolutionA particle is projected at an angle θ with an initial speed u.
View Solution
Knowledge Check
If R and H represent horizontal range and maximum height of the projectile, then the angle of projection with the horizontal is
Atan−1(HR)Btan−1(2HR)Ctan−1(4HR)Dtan−1(4HH)
What is the radius of curvature of the parabola traced out by the projectile in which a particle is projected with a speed u at an angle θ with the horizontal, at a point where the velocity of particle makes an angle θ/2 with the horizontal.
View SolutionFind the angle of projection of a projectile for which the horizontal range and maximum height are equal.
View SolutionFind the angle of projection of a projectile for which the horizontal range and maximum height are equal.
View SolutionA stone projected from ground with certain speed at an angle θ with horizontal attains maximum height h1 when it is projected with same speed at an angle θ with vertical attains height h2. The horizontal range of projectile is
View SolutionWhat is the radius of curvature of the parabola traced out by the projectile in which a particle is projected by a speed u at an angle 'theta' with the horizontal, at a point where the particle velocity makes and angle θ2 with the horizontal?
View SolutionWhat is the radius of curvature of the parabola traced out by the projectile in the previous problem projected with speed v at an angle of theta with the horizontal at a point where the particle velocity makes an angle θ2 with the horizontal?
View SolutionThe horizontal range of a projectile is 4√3 times its maximum height. Its angle of projection will be
View Solution
