A liquid drop of density ρ , radius r, and surface tension σ oscillates with time period T . Which of the following expressions for T2 is correct?
ρr3σ
ρσr3
r3σρ
None of these
The correct Answer is:A
To solve the problem of determining the correct expression for T2 in terms of the given quantities (density ρ, radius r, and surface tension σ), we will use dimensional analysis. Here’s a step-by-step breakdown of the solution:
Step 1: Identify the quantities and their dimensions
We have three quantities:
- Density ρ has dimensions (ρ)=ML−3
- Radius r has dimensions (r)=L
- Surface tension σ has dimensions (σ)=MT−2
Step 2: Set up the relationship for the time period T
We assume that the time period T is related to these quantities by the equation:
T=k⋅ρa⋅rb⋅σc
where k is a dimensionless constant and a, b, and c are the powers we need to determine.
Step 3: Write the dimensions of T
The dimension of time T is:
(T)=T
Step 4: Substitute the dimensions into the equation
Substituting the dimensions of ρ, r, and σ into the equation gives:
(T)=(ML−3)a⋅(L)b⋅(MT−2)c
This can be expanded to:
(T)=Ma+c⋅L−3a+b⋅T−2c
Step 5: Equate the dimensions
Now we equate the dimensions on both sides:
- For mass M: a+c=0
- For length L: −3a+b=0
- For time T: −2c=1
Step 6: Solve the equations
From the third equation −2c=1:
c=−12
Substituting c into the first equation:
a−12=0⟹a=12
Substituting a into the second equation:
−3(12)+b=0⟹b=32
Step 7: Write the expression for T2
Now we have:
- a=12
- b=32
- c=−12
Substituting these values back into the equation for T:
T=k⋅ρ1/2⋅r3/2⋅σ−1/2
To find T2:
T2=k2⋅ρ⋅r3⋅σ−1
Final Expression
Thus, the expression for T2 is:
T2=k2⋅ρ⋅r3σ
Conclusion
The correct expression for T2 is proportional to ρr3σ.
We have three quantities:
- Density ρ has dimensions (ρ)=ML−3
- Radius r has dimensions (r)=L
- Surface tension σ has dimensions (σ)=MT−2
We assume that the time period T is related to these quantities by the equation:
T=k⋅ρa⋅rb⋅σc
where k is a dimensionless constant and a, b, and c are the powers we need to determine.
The dimension of time T is:
(T)=T
Substituting the dimensions of ρ, r, and σ into the equation gives:
(T)=(ML−3)a⋅(L)b⋅(MT−2)c
This can be expanded to:
(T)=Ma+c⋅L−3a+b⋅T−2c
Now we equate the dimensions on both sides:
- For mass M: a+c=0
- For length L: −3a+b=0
- For time T: −2c=1
From the third equation −2c=1:
c=−12
a−12=0⟹a=12
−3(12)+b=0⟹b=32
Now we have:
- a=12
- b=32
- c=−12
T=k⋅ρ1/2⋅r3/2⋅σ−1/2
T2=k2⋅ρ⋅r3⋅σ−1
Thus, the expression for T2 is:
T2=k2⋅ρ⋅r3σ
The correct expression for T2 is proportional to ρr3σ.
Topper's Solved these Questions
- DIMENSIONS & MEASUREMENTBook:CENGAGE PHYSICS ENGLISHChapter:DIMENSIONS & MEASUREMENTExercise:Multiple Correct
Explore 2 Videos
- DIMENSIONS & MEASUREMENTBook:CENGAGE PHYSICS ENGLISHChapter:DIMENSIONS & MEASUREMENTExercise:Linked Comprehension
Explore 3 Videos
- DIMENSIONS & MEASUREMENTBook:CENGAGE PHYSICS ENGLISHChapter:DIMENSIONS & MEASUREMENTExercise:Subjective
Explore 31 Videos
- CENTRE OF MASSBook:CENGAGE PHYSICS ENGLISHChapter:CENTRE OF MASSExercise:INTEGER_TYPE
Explore 1 Video
- FLUID MECHANICSBook:CENGAGE PHYSICS ENGLISHChapter:FLUID MECHANICSExercise:INTEGER_TYPE
Explore 1 Video
Similar Questions
A spherical liquid drop is placed on a horizontal plane . A small distrubance cause the volume of the drop to oscillate . The time period oscillation (T) of the liquid drop depends on radius (r) of the drop , density (ρ) and surface tension tension (S) of the liquid. Which amount the following will be be a possible expression for T (where k is a dimensionless constant)?
View SolutionThe time period of oscillation T of a small drop of liquid under surface tension depends upon the density ρ, the radius r and surface tension σ. Using dimensional analysis show that T∝√ρr3σ
View SolutionFrequency is the function of density (ρ) , length (a) and surface tension (T) . Then its value is
View SolutionA liquid of density ρ and surface tension σ rises in a capillary tube of diameter d. Angle of contact between the tube and liquid is zero. The weight of the liquid in the capillary tube is:
View SolutionA liquid of density ρ and surface tension σ rises in a capillary tube of diameter d. Angle of contact between the tube and liquid is zero.
In the above problem, the increase in gravitational potential energy of the liquid due to rise in the capillary is :
View SolutionIf the time period t of the oscillation of a drop of liquid of density d, radius r, vibrating under surface tension s is given by the formula t=√r2bscda/2. It is observed that the time period is directly proportional to √ds . The value of b should therefore be :
View SolutionTime period of an oscillating drop of radius r, density ρ and surface tension T is t=k√ρr5T. Check the correctness of the relation
View SolutionA needle of length l and density ρ will float on a liquid of surface tension σ if its radius r is less than or equal to:
View SolutionA sphere of radius r is dropped in a liquid from its surface. Which of the following graphs is/are correct?

View SolutionCalculate the pressure inside a small air bubble of radus r situated at a depth h below the free surface of liquids of densities ρ1 and ρ2 and surface tennsions T1 and T2. The thickness of the first and second liquids are h1 and h2 respectively. Take atmosphere pressure =P0.
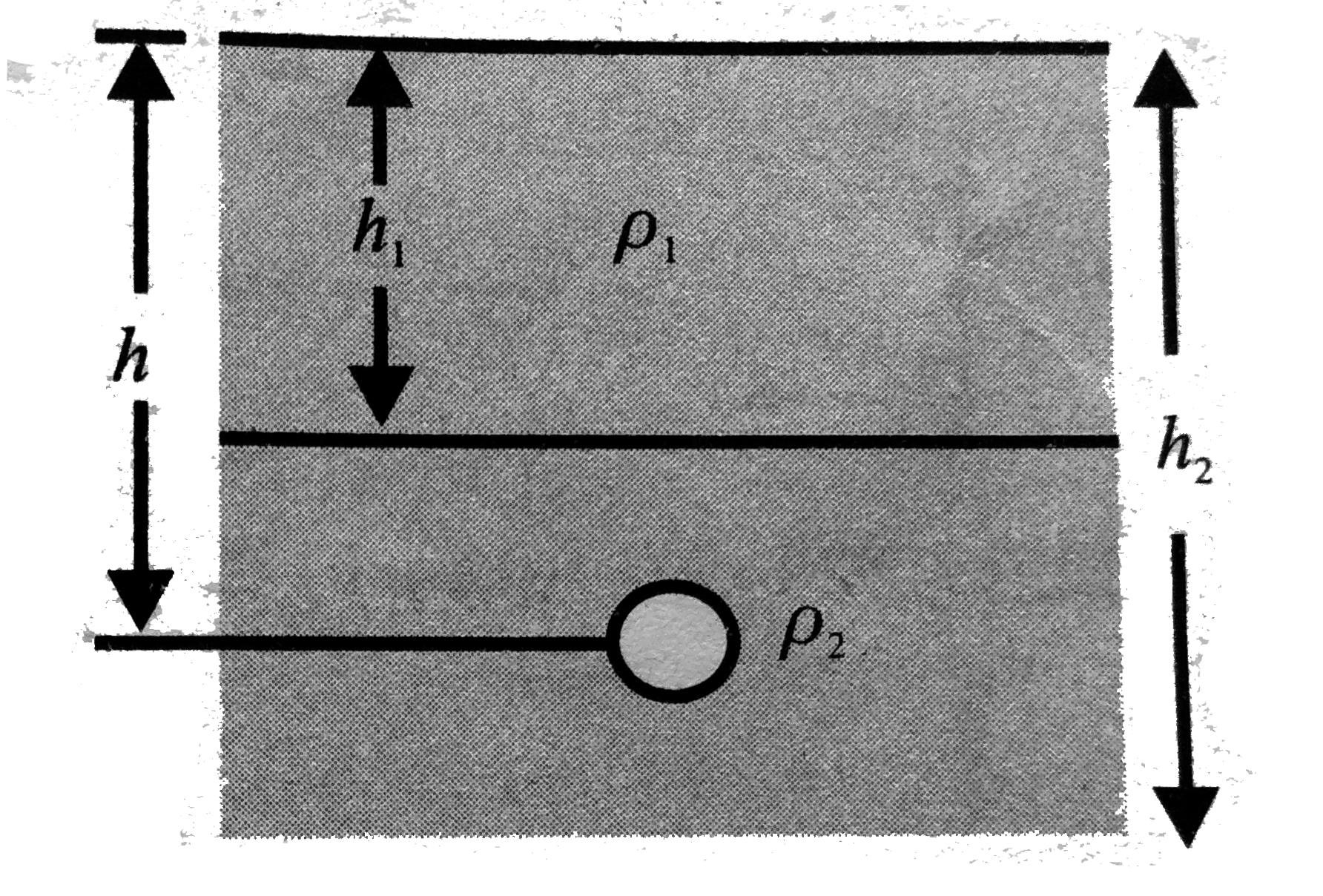
View Solution