Choose the correct statement(s).
A dimensionally correct equation must be correct.
A dimensionally correct equation may br incorrect.
A dimensionally incorrect equation must be correct.
A dimensionally incorrect equation may be correct.
The correct Answer is:B, D
To solve the question, we need to evaluate the four statements regarding dimensional correctness and numerical correctness of equations. Let's break it down step by step.
Step 1: Understand the concept of dimensional correctness
- A dimensionally correct equation is one where the dimensions on both sides of the equation match. However, this does not guarantee that the equation is numerically correct.
Hint: Remember that dimensional analysis checks the units but does not confirm the numerical values.
Step 2: Evaluate the first statement
- Statement 1: "A dimensionally correct equation must be correct."
- This statement is false because an equation can be dimensionally correct but still yield incorrect numerical results. For example, the equation s=ut+12at2 is dimensionally correct but can be numerically incorrect if the values of u, a, and t are not accurate.
Hint: Think of examples where the dimensions match but the values do not.
Step 3: Evaluate the second statement
- Statement 2: "A dimensionally correct equation may be incorrect."
- This statement is true. As explained in the previous step, a dimensionally correct equation can still be numerically incorrect.
Hint: Consider the implications of using incorrect values in a dimensionally correct equation.
Step 4: Evaluate the third statement
- Statement 3: "A dimensionally incorrect equation must be correct."
- This statement is false. An equation can be dimensionally incorrect and still yield incorrect numerical results. For example, if an equation does not maintain dimensional consistency, it cannot be relied upon to give correct results.
Hint: Reflect on the importance of dimensional analysis in verifying the validity of equations.
Step 5: Evaluate the fourth statement
- Statement 4: "A dimensionally incorrect equation may be correct."
- This statement is true. There are instances where an equation may not be dimensionally correct but can still provide a numerically accurate result. For example, the equation S=u+a2 might yield a correct numerical value despite being dimensionally incorrect.
Hint: Think about cases where empirical formulas might not adhere to dimensional analysis yet still work in practice.
Conclusion
Based on the evaluations:
- The correct statements are:
- Statement 2: A dimensionally correct equation may be incorrect.
- Statement 4: A dimensionally incorrect equation may be correct.
Final Answer: The correct statements are 2 and 4.
- A dimensionally correct equation is one where the dimensions on both sides of the equation match. However, this does not guarantee that the equation is numerically correct.
- Statement 1: "A dimensionally correct equation must be correct."
- This statement is false because an equation can be dimensionally correct but still yield incorrect numerical results. For example, the equation s=ut+12at2 is dimensionally correct but can be numerically incorrect if the values of u, a, and t are not accurate.
- Statement 2: "A dimensionally correct equation may be incorrect."
- This statement is true. As explained in the previous step, a dimensionally correct equation can still be numerically incorrect.
- Statement 3: "A dimensionally incorrect equation must be correct."
- This statement is false. An equation can be dimensionally incorrect and still yield incorrect numerical results. For example, if an equation does not maintain dimensional consistency, it cannot be relied upon to give correct results.
- Statement 4: "A dimensionally incorrect equation may be correct."
- This statement is true. There are instances where an equation may not be dimensionally correct but can still provide a numerically accurate result. For example, the equation S=u+a2 might yield a correct numerical value despite being dimensionally incorrect.
Based on the evaluations:
- The correct statements are:
- Statement 2: A dimensionally correct equation may be incorrect.
- Statement 4: A dimensionally incorrect equation may be correct.
Topper's Solved these Questions
- DIMENSIONS & MEASUREMENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Multiple Correct
2 Videos
- DIMENSIONS & MEASUREMENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Linked Comprehension
3 Videos
- DIMENSIONS & MEASUREMENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Subjective
31 Videos
- CENTRE OF MASSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - INTEGER_TYPE
1 Videos
- FLUID MECHANICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - INTEGER_TYPE
1 Videos
Similar Questions
Choose the correct statements(s):
View SolutionConsider the following compounds:
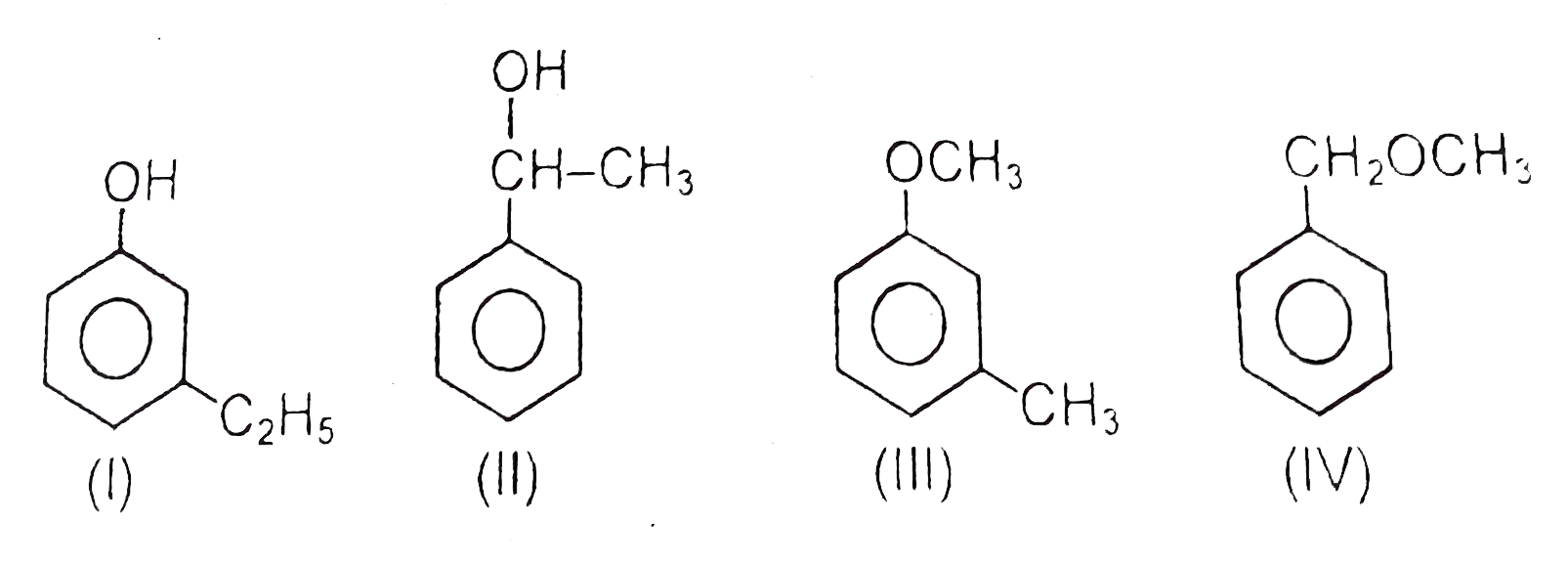
Choose the correct statements(s) from the following:
View Solution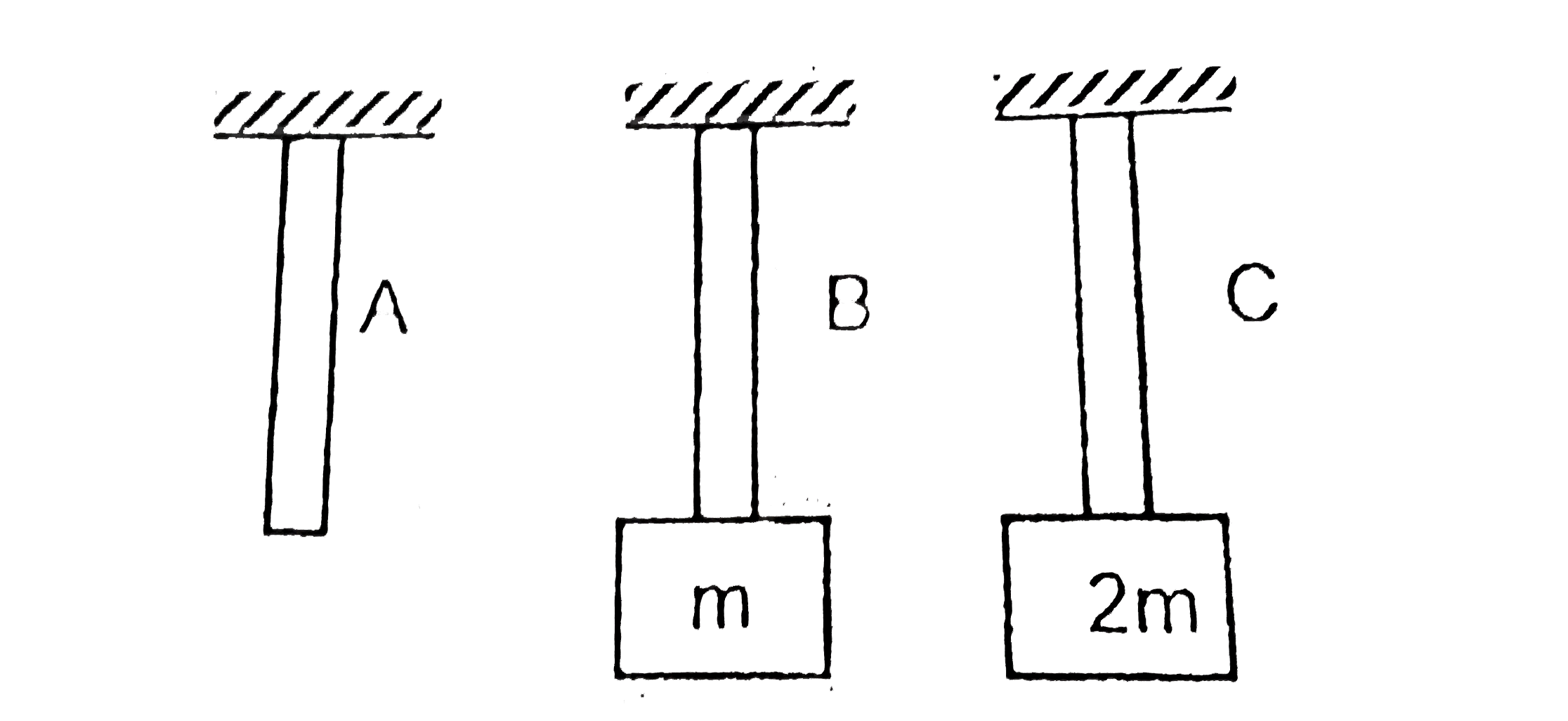
Consider the three ropes of same length and same linear density as (shown in figure) suspended from the ceiling.
A pulse is generated at the lowest point of the three ropes simultaneously. Choose the correct statements(s)
View Solution
In the adjacent figure blocks A and B of weight W each are lying at rest on a rough horizontal surface. They are pulled by applying forces F1 and F2 as shown in figure. When blocks are in limiting equilibrium F2=5F1 Choose the correct statement (s) (Assume same coefficient of friction for both the blocks)
View SolutionA curved surface of radius R separates two medium of refractive indices μ1andμ2 as shown in figures A and B .
_E01_077_Q01.png)
Choose the correct statement(s) related to the real image formed by the object O placed at a distance x, as shown in figure A.
View SolutionA curved surface of radius R separates two medium of refractive indices μ1andμ2 as shown in figures A and B .
_E01_078_Q01.png)
Choose the correct statement(s) related to the virtual image formed by object O placed at a distance x, as shown in figure
View SolutionThe instantaneous charge on capacitor in two discharging RC circuils is plotted with respect to time in figure. Choose the correct statement (s) (where E1 and E2 are emfs of two DC sources in two different charging circuits and capacitors are fully charged ).
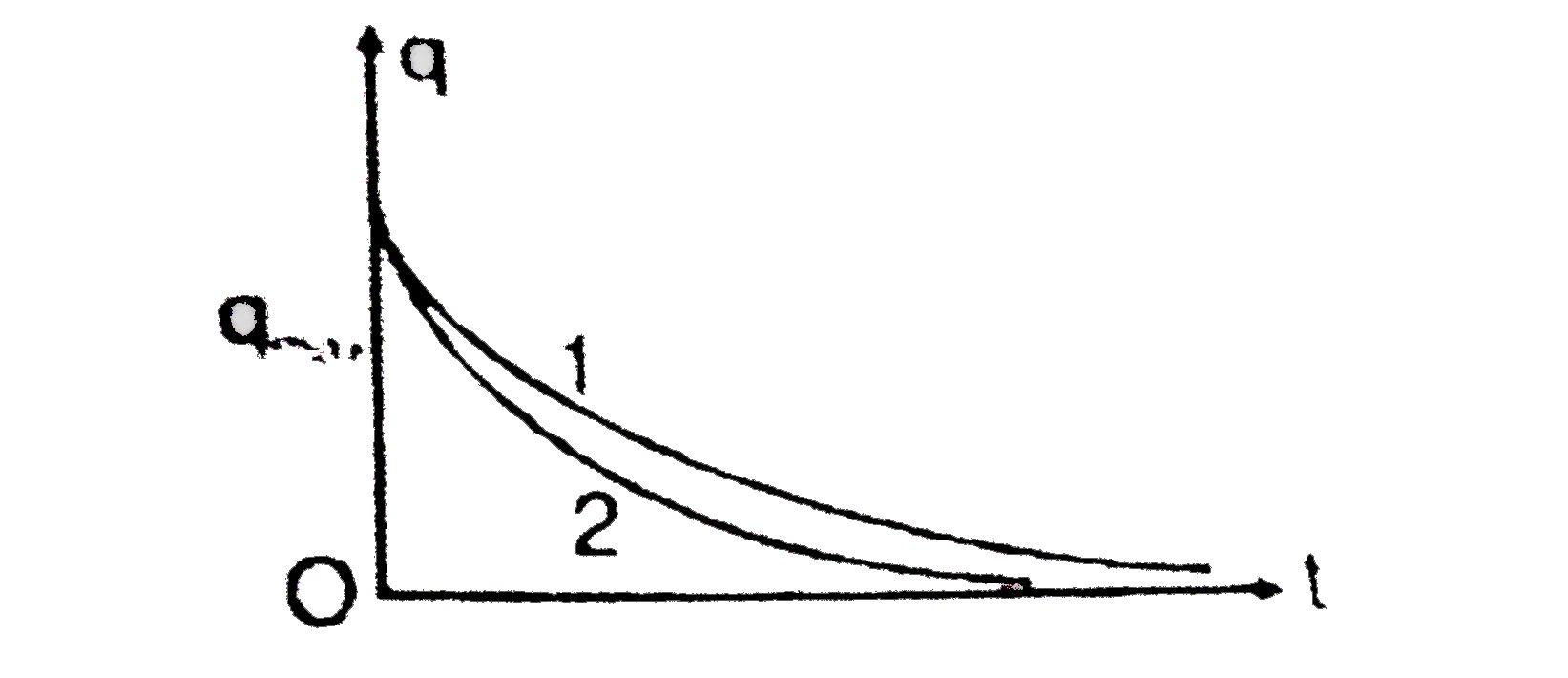
View SolutionChoose the correct statements
View SolutionChoose the correct statements.
View SolutionChoose the correct statements
View Solution
