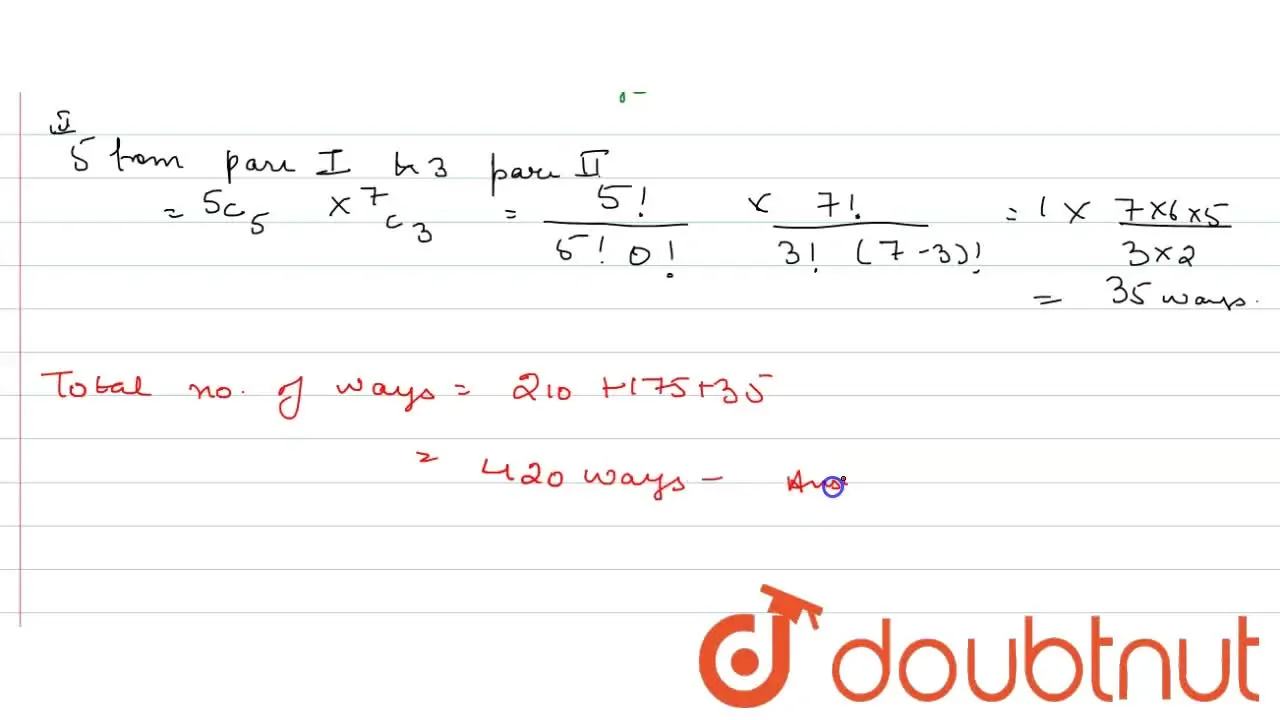
In an examination, a question pater consists of 12 questions divided into two parts i.e., Part I and Part II, containing 5 and 7 questions, respectively. A student is required to attempt 8 questions in all, selecting at least 3 from each part. In how many ways can a students select the questions ?
The correct Answer is:420
Step by step video, text & image solution for In an examination, a question pater consists of 12 questions divided into two parts i.e., Part I and Part II, containing 5 and 7 questions, respectively. A student is required to attempt 8 questions in all, selecting at least 3 from each part. In how many ways can a students select the questions ? by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Related Playlists
- BOOK - ICSECHAPTER - MODEL TEST PAPER -11EXERCISE - Sections - B11videos
MODEL TEST PAPER -11
- BOOK - ICSECHAPTER - MODEL TEST PAPER -11EXERCISE - Sections - C10videos
MODEL TEST PAPER -11
- BOOK - ICSECHAPTER - MODEL TEST PAPER - 9 EXERCISE - SECTION - C9videos
MODEL TEST PAPER - 9
- BOOK - ICSECHAPTER - MODEL TEST PAPER -12EXERCISE - Section - C10videos
MODEL TEST PAPER -12
Similar Questions
In an examination, a question paper consists of 12 questions divided into two parts i.e., Part I and Part II, containing 5 and 7 questions, respectively. A student is required to attempt 8 questions in all, selecting at least 3 from each part. In how many ways can a student select the questions?
View SolutionA mathematics paper consists of 10 questions divided into two parts I and II each part containing 5 questions. A student is required to attempt 6 questions in al, taking at least 2 questions from each part. In how many ways can student select the questions?
View SolutionA question paper on mathematics consists of 12 questions divided into 3 parts A, B and C, each containing 4 questions. In how many ways can an examinee answer 5 questions selecting at least one from each part.
View SolutionA question paper on mathematics consists of 12 questions divided in to 3 pars A, B and C, each containing 4 questions. In how many ways can an examinee answer questions selecting at least one from each part.
View SolutionThere are 3 parts A,B and C in a question paper of Math's, which includes 6,7 and 8 questions respectivelty. From these parts 3,4 and 5 questions respectively are to be solved. In how many ways can a student select the questions from these parts?
View SolutionA question paper consists of two sections having respectively, 3 and 5 questions. The followinng note is given on the paper "it is not necessory to attempt all the questions one questions from each section is compulsory". In how many ways can candidate select the questions?
View SolutionTwo subjects are compulsory for a student in an examination. In how many ways can a student select 5 subjects out of given 10 subjects?
View SolutionA student has to answer 10 questions, choosing at least 4 from each of part A and B. If there are 6 questions in part A and 7 in part B. In how many ways can the student choose 10 questions?
View SolutionThere are three sections in a question paper, each containing 5 questions. A candidate has to solve any 5 questions, choosing at least one from each section. Find the number of ways in which the candidate can choose the questions.
View SolutionFritz is taking an examination that consists of two parts , A and B , with the following instructions:
Part A contains three questions, and a student must answer tow .
Part B contains four questions, and a student must answer two.
Part A must be completed before starting part B.
How many ways can the test be completed?View Solution